Next: Lagrange points Up: Three-body problem Previous: Tisserand criterion
 about an axis normal
to the orbital plane of masses
about an axis normal
to the orbital plane of masses 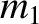 and
and  , and passing through their center of mass.
It
follows that masses
, and passing through their center of mass.
It
follows that masses 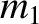 and
and  appear stationary in this new reference frame.
Let us define a Cartesian coordinate system
appear stationary in this new reference frame.
Let us define a Cartesian coordinate system 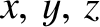 in the rotating frame of reference that is
such that masses
in the rotating frame of reference that is
such that masses 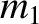 and
and  always lie on the
always lie on the  -axis, and the
-axis, and the  -axis
is parallel to the previously defined
-axis
is parallel to the previously defined  -axis. It follows that masses
-axis. It follows that masses
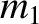 and
and  have the fixed position vectors
have the fixed position vectors
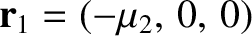 and
and
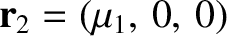 in our new coordinate system. Finally, let the position vector of
mass
in our new coordinate system. Finally, let the position vector of
mass  be
be
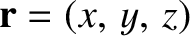 . See Figure 9.5.
. See Figure 9.5.
According to Section 6.2, the equation of motion of mass  in the rotating
reference frame takes the form
in the rotating
reference frame takes the form

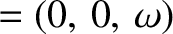 , and
, and
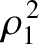 |
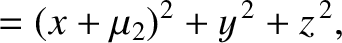 |
(9.22) |
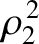 |
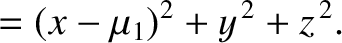 |
(9.23) |
 |
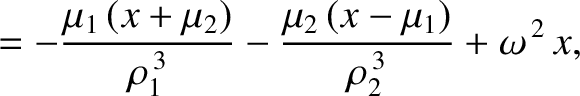 |
(9.24) |
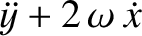 |
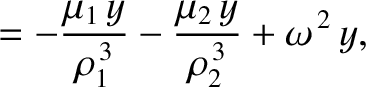 |
(9.25) |
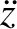 |
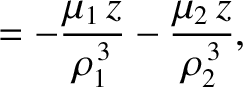 |
(9.26) |
It follows from Equations (9.27)–(9.29) that
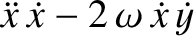 |
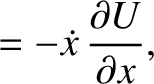 |
(9.31) |
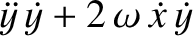 |
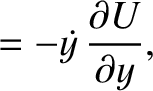 |
(9.32) |
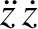 |
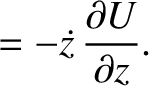 |
(9.33) |
![$\displaystyle \frac{d}{dt}\!\left[\frac{1}{2}\left(\skew{3}\dot{x}^{\,2}+\skew{3}\dot{y}^{\,2}+\skew{3}\dot{z}^{\,2}\right) + U\right] = 0.$](img2171.png) |
(9.34) |
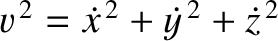 . In fact,
. In fact,  is the
Jacobi integral introduced in Section 9.3 [it is easily demonstrated that Equations (9.10) and
(9.35) are identical; see Section 9.9, Exercise 4].
Note, finally, that
the mass
is the
Jacobi integral introduced in Section 9.3 [it is easily demonstrated that Equations (9.10) and
(9.35) are identical; see Section 9.9, Exercise 4].
Note, finally, that
the mass  is restricted to regions in which
is restricted to regions in which
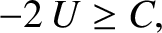 |
(9.36) |
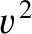 is a positive definite quantity.
is a positive definite quantity.