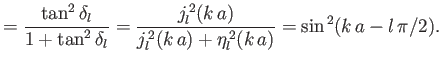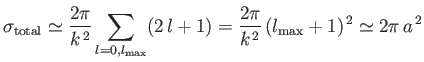

Next: Low-Energy Scattering
Up: Scattering Theory
Previous: Determination of Phase-Shifts
Hard-Sphere Scattering
Let us try out the scheme outlined in the previous section using a particularly simple example. Consider
scattering by a hard sphere, for which the potential is infinite
for 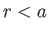 , and zero for
, and zero for 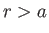 . It follows that
. It follows that
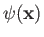 is
zero in the region
is
zero in the region 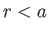 , which implies that
, which implies that 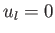 for all
for all 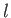 .
Thus,
.
Thus,
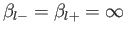 |
(10.103) |
for all 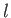 . Equation (10.97) yields
. Equation (10.97) yields
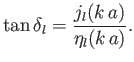 |
(10.104) |
In fact, this result is most easily obtained from the obvious requirement that 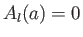 . [See Equation (10.95).]
. [See Equation (10.95).]
Consider the 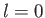 partial wave, which is usually referred to as the S-wave.
Equation (10.105) gives
partial wave, which is usually referred to as the S-wave.
Equation (10.105) gives
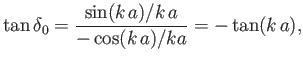 |
(10.105) |
where use has been made of Equations (10.60) and (10.61). It follows that
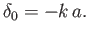 |
(10.106) |
The S-wave radial wave function
is
[See Equation (10.95).]
The corresponding radial wavefunction for the incident wave
takes the form
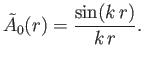 |
(10.108) |
[See Equations (10.79), (10.80), (10.94), and (10.107).]
It is clear that the actual  radial wavefunction is similar to the
incident
radial wavefunction is similar to the
incident  wavefunction, except that it is phase-shifted by
wavefunction, except that it is phase-shifted by  .
.
Let us consider the low- and high-energy asymptotic limits of
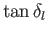 .
Low energy corresponds to
.
Low energy corresponds to 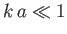 . In this limit, the spherical Bessel functions
and Neumann functions reduce to
. In this limit, the spherical Bessel functions
and Neumann functions reduce to
where
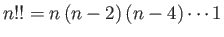 [1]. It follows that
[1]. It follows that
![$\displaystyle \tan\delta_l = \frac{-(k\,a)^{\,2\,l+1}}{(2\,l+1) \,[(2\,l-1)!!]^{\,2}}.$](img3619.png) |
(10.111) |
It is clear that we can neglect 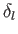 , with
, with 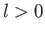 , with respect to
, with respect to
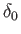 . In other words, at low energy, only S-wave scattering
(i.e., spherically symmetric scattering) is important. It follows
from Equations (10.28), (10.81), and (10.107) that
. In other words, at low energy, only S-wave scattering
(i.e., spherically symmetric scattering) is important. It follows
from Equations (10.28), (10.81), and (10.107) that
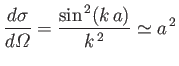 |
(10.112) |
for 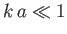 . Note that the total cross-section,
. Note that the total cross-section,
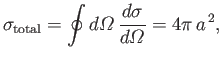 |
(10.113) |
is four times the geometric cross-section,
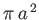 (i.e., the cross-section for classical particles bouncing off a
hard sphere of radius
(i.e., the cross-section for classical particles bouncing off a
hard sphere of radius 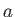 ).
However,
low-energy scattering implies relatively long de Broglie wavelengths, so we would not
expect to obtain the classical result in this limit.
).
However,
low-energy scattering implies relatively long de Broglie wavelengths, so we would not
expect to obtain the classical result in this limit.
Consider the high-energy limit, 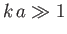 . At high energies, by analogy with classical scattering, the scattered particles with the largest
angular momenta about the origin have angular momenta
. At high energies, by analogy with classical scattering, the scattered particles with the largest
angular momenta about the origin have angular momenta
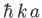 (i.e., the product of their
incident momenta,
(i.e., the product of their
incident momenta, 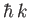 , and their maximum possible impact parameters,
, and their maximum possible impact parameters, 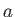 ). Given that particles in the
). Given that particles in the
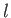 th partial wave have angular momenta
th partial wave have angular momenta
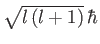 , we deduce that
all partial
waves up to
, we deduce that
all partial
waves up to
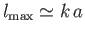 contribute significantly to
the scattering cross-section. It follows from Equation (10.90) that
contribute significantly to
the scattering cross-section. It follows from Equation (10.90) that
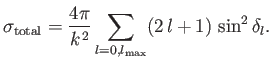 |
(10.114) |
Making use of Equation (10.105), as well as the asymptotic expansions (10.62) and (10.63),
we find that
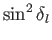 |
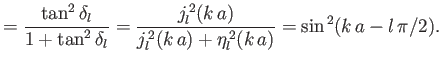 |
(10.115) |
In particular,
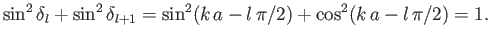 |
(10.116) |
Hence, it is a good approximation to write
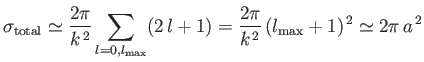 |
(10.117) |
[59].
This is twice the classical result, which is somewhat surprising,
because we might expect to obtain the classical result in the short-wavelength limit. In fact, for hard-sphere scattering, all incident particles with
impact parameters less than 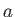 are deflected. However, in order to
produce a shadow behind the sphere, there must be scattering
in the forward direction (recall the optical theorem) to produce
destructive interference with the incident plane wave. The effective cross-section associated with
this forward scattering is
are deflected. However, in order to
produce a shadow behind the sphere, there must be scattering
in the forward direction (recall the optical theorem) to produce
destructive interference with the incident plane wave. The effective cross-section associated with
this forward scattering is
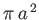 , which, when combined with the
cross-section for classical reflection,
, which, when combined with the
cross-section for classical reflection,
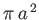 , gives the actual
cross-section of
, gives the actual
cross-section of
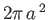 [95].
[95].


Next: Low-Energy Scattering
Up: Scattering Theory
Previous: Determination of Phase-Shifts
Richard Fitzpatrick
2016-01-22
![]() partial wave, which is usually referred to as the S-wave.
Equation (10.105) gives
partial wave, which is usually referred to as the S-wave.
Equation (10.105) gives
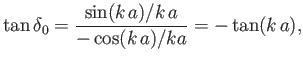
![$\displaystyle = \exp(-{\rm i}\, k\,a) \left[\frac{\cos (k\,a) \,\sin (k\,r) -\sin (k\,a) \,\cos( k\,r)}{k\,r}\right]$](img3608.png)
![$\displaystyle =\exp(-{\rm i}\, k\,a)\, \frac{ \sin[k\,(r-a)]}{k\,r}.$](img3609.png)
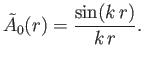
![]() .
Low energy corresponds to
.
Low energy corresponds to ![]() . In this limit, the spherical Bessel functions
and Neumann functions reduce to
. In this limit, the spherical Bessel functions
and Neumann functions reduce to
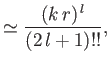
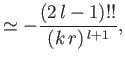
![$\displaystyle \tan\delta_l = \frac{-(k\,a)^{\,2\,l+1}}{(2\,l+1) \,[(2\,l-1)!!]^{\,2}}.$](img3619.png)
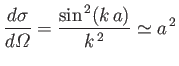
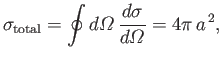
![]() . At high energies, by analogy with classical scattering, the scattered particles with the largest
angular momenta about the origin have angular momenta
. At high energies, by analogy with classical scattering, the scattered particles with the largest
angular momenta about the origin have angular momenta
![]() (i.e., the product of their
incident momenta,
(i.e., the product of their
incident momenta, ![]() , and their maximum possible impact parameters,
, and their maximum possible impact parameters, ![]() ). Given that particles in the
). Given that particles in the
![]() th partial wave have angular momenta
th partial wave have angular momenta
![]() , we deduce that
all partial
waves up to
, we deduce that
all partial
waves up to
![]() contribute significantly to
the scattering cross-section. It follows from Equation (10.90) that
contribute significantly to
the scattering cross-section. It follows from Equation (10.90) that