Center of Mass
The center of mass of a dynamical system is an imaginary point whose coordinates are the
mass-weighted average of the coordinates of the system's constituent particles. It follows that
the displacement of the center of mass is
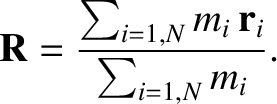 |
(1.68) |
The velocity of the center of mass, which is obtained by differentiating the previous expression with respect to
time, is
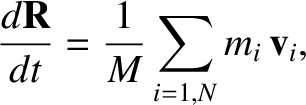 |
(1.69) |
where
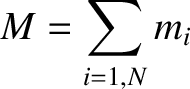 |
(1.70) |
is the total mass of the system. Likewise, the acceleration of the center of mass is
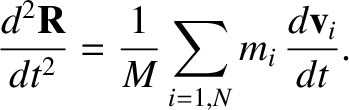 |
(1.71) |
A comparison of Equations (1.66) and (1.71) reveals that
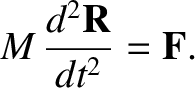 |
(1.72) |
We conclude that the center of mass moves like a particle of mass  subject to the net external
force,
subject to the net external
force, 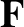 , acting on the system. In particular, the motion of the center of mass is completely
unaffected by the internal forces that the system's constituent particle exert on one another.
, acting on the system. In particular, the motion of the center of mass is completely
unaffected by the internal forces that the system's constituent particle exert on one another.
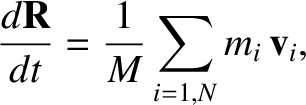
 subject to the net external
force,
subject to the net external
force, 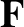 , acting on the system. In particular, the motion of the center of mass is completely
unaffected by the internal forces that the system's constituent particle exert on one another.
, acting on the system. In particular, the motion of the center of mass is completely
unaffected by the internal forces that the system's constituent particle exert on one another.