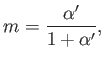Next: Two-Dimensional Jets
Up: Two-Dimensional Incompressible Inviscid Flow
Previous: Inviscid Flow Past a
Inviscid Flow Over a Semi-Infinite Wedge
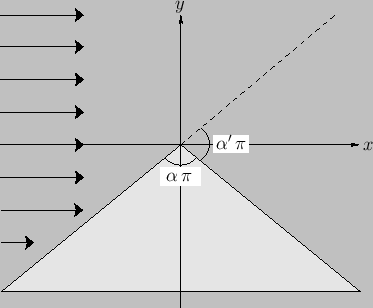
Consider the situation illustrated in Figure 5.12 in which an incompressible irrotational fluid flows over an
impenetrable rigid wedge whose apex subtends an angle
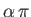 . Let the cross-section of the wedge in the
. Let the cross-section of the wedge in the  -
- plane
be both
plane
be both  -independent and symmetric about the
-independent and symmetric about the  -axis. Furthermore, let the apex of
the wedge lie at
-axis. Furthermore, let the apex of
the wedge lie at  . Finally, let the upstream flow a large distance from the
wedge be parallel to the
. Finally, let the upstream flow a large distance from the
wedge be parallel to the  -axis.
-axis.
Figure 5.12:
Inviscid flow over a wedge.
 |
Because the flow is two-dimensional, incompressible, and irrotational, it can be represented in terms of a stream function that
satisfies Laplace's equation. The
boundary conditions on the stream function are
![$\displaystyle \psi\left(r,[3-\alpha]\,\pi/2\right) =\psi\left(r,-[1-\alpha]\,\pi/2\right)=0.$](img1878.png) |
(5.130) |
These boundary conditions ensure that the normal velocity at the surface of the wedge is zero.
It is easily demonstrated that
![$\displaystyle \psi(r,\theta) = -\frac{A}{1-m}\,r^{\,1-m}\,\cos\left[(1-m)\,(\theta-\pi/2)\right]$](img1879.png) |
(5.131) |
is a solution of Laplace's equation, (5.61). Moreover, this solution satisfies the boundary conditions provided that
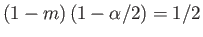 , or
, or
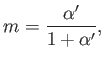 |
(5.132) |
where
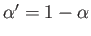 .
Because the solutions to Laplace's equation are
unique, we can again be sure that Equation (5.131) is the correct
solution to the problem under investigation. According to this solution, the tangential velocity on the surface of the
wedge is given by
.
Because the solutions to Laplace's equation are
unique, we can again be sure that Equation (5.131) is the correct
solution to the problem under investigation. According to this solution, the tangential velocity on the surface of the
wedge is given by
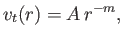 |
(5.133) |
where 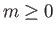 .
Note that the tangential velocity, and hence the flow speed, is infinite at the apex of the wedge. However, this
singularity in the flow can be eliminated by slightly rounding the apex.
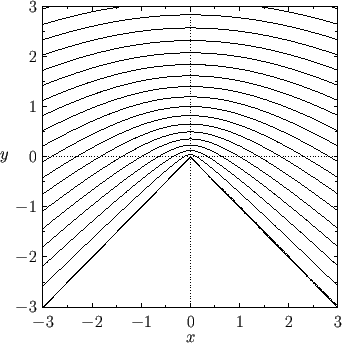
Figure 5.13 shows the streamlines of the
flow for the case
.
Note that the tangential velocity, and hence the flow speed, is infinite at the apex of the wedge. However, this
singularity in the flow can be eliminated by slightly rounding the apex.
Figure 5.13 shows the streamlines of the
flow for the case
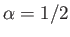 .
.
Figure:
Streamlines of inviscid incompressible irrotational flow over a 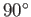 wedge.
wedge.
 |



Next: Two-Dimensional Jets
Up: Two-Dimensional Incompressible Inviscid Flow
Previous: Inviscid Flow Past a
Richard Fitzpatrick
2016-01-22