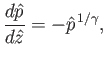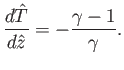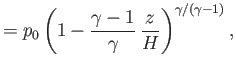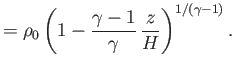Next: Atmospheric Stability
Up: Equilibrium of Compressible Fluids
Previous: Isothermal Atmosphere
In fact, the temperature of the Earth's atmosphere is not uniform, but instead decreases steadily with increasing altitude. This effect is largely due to the
action of convection currents. When a packet of air ascends, under the influence of such currents, the diminished pressure at higher altitudes causes it to
expand. Because this expansion generally takes place far more rapidly than heat can diffuse into the packet, the work done
against the pressure of the surrounding gas, as the packet expands, leads to a reduction in its internal energy, and, hence, in its temperature. Assuming that the atmosphere is in a continually mixed state, while remaining in approximate vertical force balance (such
a state is known as a convective equilibrium),
and that the effect of heat conduction is
negligible (because the mixing takes place too rapidly for thermal diffusion to affect the temperature), we would expect the adiabatic gas law, (13.4),
to offer a much more accurate description of the relationship between atmospheric pressure and density than the isothermal gas law, (13.3).
Let
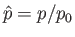 ,
,
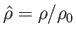 , and
, and
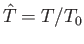 , where
, where 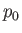 ,
, 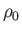 , and
, and 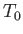 are the
pressure, mass density, and temperature of the atmosphere, respectively, at ground level. The adiabatic
gas law, (13.4), can be combined with the ideal gas equation of state, (13.6), to give
are the
pressure, mass density, and temperature of the atmosphere, respectively, at ground level. The adiabatic
gas law, (13.4), can be combined with the ideal gas equation of state, (13.6), to give
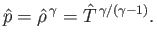 |
(13.11) |
The isothermal scale height of the atmosphere is conveniently redefined as [cf., Equation (13.9)]
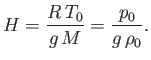 |
(13.12) |
Equations (13.5), (13.11), and (13.12)
yield
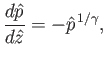 |
(13.13) |
where
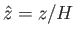 ,
or, from Equation (13.11),
,
or, from Equation (13.11),
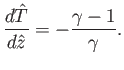 |
(13.14) |
The previous equation can be integrated to give
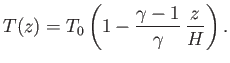 |
(13.15) |
It follows that the temperature in an adiabatic atmosphere decreases linearly with increasing altitude at the rate
of
![$ [(\gamma-1)/\gamma]\,(T_0/H)$](img5144.png) degrees per meter. This rate is known as the adiabatic lapse rate of
the atmosphere. Using the values
degrees per meter. This rate is known as the adiabatic lapse rate of
the atmosphere. Using the values
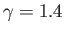 ,
,
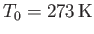 , and
, and
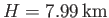 , which
are typical of the Earth's atmosphere, we estimate the lapse rate to be
, which
are typical of the Earth's atmosphere, we estimate the lapse rate to be
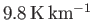 .
In reality, the lapse rate only takes this value in dry air. In moist air, the lapse rate is considerably reduced because of
the latent heat released when water vapor condenses.
.
In reality, the lapse rate only takes this value in dry air. In moist air, the lapse rate is considerably reduced because of
the latent heat released when water vapor condenses.
Equations (13.11) and (13.15) yield
Because
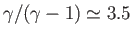 and
and
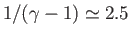 , it follows that pressure decreases more
rapidly than density in an adiabatic atmosphere. Moreover, the previous three equations imply that an adiabatic
atmosphere has a sharp upper boundary at
, it follows that pressure decreases more
rapidly than density in an adiabatic atmosphere. Moreover, the previous three equations imply that an adiabatic
atmosphere has a sharp upper boundary at
![$ z=[\gamma/(\gamma-1)]\,H\simeq 28\,{\rm km}$](img5153.png) . At this altitude,
the temperature, pressure, and density all fall to zero. Of course, above this altitude, the temperature, pressure, and
density remain zero (because they cannot take negative or imaginary values). In contrast, an isothermal
atmosphere has a diffuse upper boundary in which the pressure and density never fall to zero, even at extreme altitudes.
It should be noted that, in reality, the Earth's atmosphere does not have a sharp upper boundary, because the adiabatic
gas law does not hold at very high altitudes.
. At this altitude,
the temperature, pressure, and density all fall to zero. Of course, above this altitude, the temperature, pressure, and
density remain zero (because they cannot take negative or imaginary values). In contrast, an isothermal
atmosphere has a diffuse upper boundary in which the pressure and density never fall to zero, even at extreme altitudes.
It should be noted that, in reality, the Earth's atmosphere does not have a sharp upper boundary, because the adiabatic
gas law does not hold at very high altitudes.



Next: Atmospheric Stability
Up: Equilibrium of Compressible Fluids
Previous: Isothermal Atmosphere
Richard Fitzpatrick
2016-01-22
![]() ,
,
![]() , and
, and
![]() , where
, where ![]() ,
, ![]() , and
, and ![]() are the
pressure, mass density, and temperature of the atmosphere, respectively, at ground level. The adiabatic
gas law, (13.4), can be combined with the ideal gas equation of state, (13.6), to give
are the
pressure, mass density, and temperature of the atmosphere, respectively, at ground level. The adiabatic
gas law, (13.4), can be combined with the ideal gas equation of state, (13.6), to give