Introduction

This chapter investigates incompressible flow in which viscosity plays a significant role throughout the bulk of the fluid. Such flow generally takes place at relatively low Reynolds number. From Section 1.14, the equations governing incompressible viscous fluid motion can be written

$$\nabla\cdot{\bf v} = 0,$$ (10.1)

$$\rho\,\frac{D{\bf v}}{Dt} = - \nabla P + \mu\,\nabla^{\,2}{\bf v},$$ (10.2)

where the quantity

$$P = p + \rho\,{\mit\Psi},$$ (10.3)

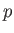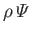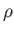which is a combination of the actual fluid pressure, $p$ , and the gravitational potential energy per unit volume, $\rho\,{\mit\Psi}$ , is known as the effective pressure. Here, $\rho$ is the fluid mass density, 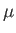$\mu$ the fluid viscosity, and 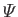${\mit\Psi}$ the gravitational potential. More information on this topic can be found in Batchelor 2000.