

Next: The wave equation
Up: The diffusion equation
Previous: An example 2-d solution
The techniques discussed above for solving
the diffusion equation in two dimensions with a restricted class of boundary conditions can easily
be generalized to three dimensions. In the 3-d case, it is necessary to Fourier
transform in two directions (the 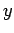 and
and 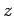 directions, say) in order to reduce the
problem to a system of uncoupled tridiagonal matrix equations. These equations can be inverted
in the usual manner, and the solution can then be reconstructed via a double inverse Fourier
transform.
directions, say) in order to reduce the
problem to a system of uncoupled tridiagonal matrix equations. These equations can be inverted
in the usual manner, and the solution can then be reconstructed via a double inverse Fourier
transform.
Richard Fitzpatrick
2006-03-29