An example 1D PIC code

The following code is an implementation of the ideas developed above.

The main function reads in the calculation parameters, checks that they are sensible, initializes the electron coordinates, and then evolves the electron equations of motion from $t=0$ to some specified $t_{\rm max}$, using a fixed step RK4 routine with some specified time-step $\delta t$. Information on the electron phase-space coordinates and the electric field is periodically written to various data-files.

// 1-d PIC code to solve plasma two-stream instability problem.

#include <stdlib.h>
#include <stdio.h>
#include <math.h>
#include <time.h>
#include <blitz/array.h>
#include <fftw.h>

using namespace blitz;

void Output (char* fn1, char* fn2, double t, 
	     Array<double,1> r, Array<double,1> v);
void Density (Array<double,1> r, Array<double,1>& n);
void Electric (Array<double,1> phi, Array<double,1>& E);
void Poisson1D (Array<double,1>& u, Array<double,1> v, double kappa);
void rk4_fixed (double& x, Array<double,1>& y, 
              void (*rhs_eval)(double, Array<double,1>, Array<double,1>&), 
              double h);
void rhs_eval (double t, Array<double,1> y, Array<double,1>& dydt);
void Load (Array<double,1> r, Array<double,1> v, Array<double,1>& y);
void UnLoad (Array<double,1> y, Array<double,1>& r, Array<double,1>& v);
double distribution (double vb);

double L; int N, J;

int main()
{
  // Parameters
  L;            // Domain of solution 0 <= x <= L (in Debye lengths)
  N;            // Number of electrons
  J;            // Number of grid points
  double vb;    // Beam velocity
  double dt;    // Time-step (in inverse plasma frequencies)
  double tmax;  // Simulation run from t = 0. to t = tmax

  // Get parameters
  printf ("Please input N:  "); scanf ("%d", &N);
  printf ("Please input vb:  "); scanf ("%lf", &vb); 
  printf ("Please input L:  "); scanf ("%lf", &L);
  printf ("Please input J:  "); scanf ("%d", &J);
  printf ("Please input dt:  "); scanf ("%lf", &dt); 
  printf ("Please input tmax:  "); scanf ("%lf", &tmax);
  int skip = int (tmax / dt) / 10;
  if ((N < 1) || (J < 2) || (L <= 0.) || (vb <= 0.) 
      || (dt <= 0.) || (tmax <= 0.) || (skip < 1))
    {
      printf ("Error - invalid input parameters\n");
      exit (1);
    }

  // Set names of output files
  char* phase[11]; char* data[11];
  phase[0] = "phase0.out";phase[1] = "phase1.out";phase[2] = "phase2.out"; 
  phase[3] = "phase3.out";phase[4] = "phase4.out";phase[5] = "phase5.out"; 
  phase[6] = "phase6.out";phase[7] = "phase7.out";phase[8] = "phase8.out";
  phase[9] = "phase9.out";phase[10] = "phase10.out";data[0] = "data0.out";  
  data[1] = "data1.out"; data[2] = "data2.out"; data[3] = "data3.out"; 
  data[4] = "data4.out"; data[5] = "data5.out"; data[6] = "data6.out"; 
  data[7] = "data7.out"; data[8] = "data8.out"; data[9] = "data9.out"; 
  data[10] = "data10.out";

  // Initialize solution
  double t = 0.;
  int seed = time (NULL); srand (seed);
  Array<double,1> r(N), v(N);
  for (int i = 0; i < N; i++)
    {
      r(i) = L * double (rand ()) / double (RAND_MAX);
      v(i) = distribution (vb);
    }
  Output (phase[0], data[0], t, r, v);

  // Evolve solution
  Array<double,1> y(2*N);
  Load (r, v, y);
  for (int k = 1; k <= 10; k++)
    {
      for (int kk = 0; kk < skip; kk++)
        {
           // Take time-step
           rk4_fixed (t, y, rhs_eval, dt);

           // Make sure all coordinates in range 0 to L.
           for (int i = 0; i < N; i++)
             {
               if (y(i) < 0.) y(i) += L;
               if (y(i) > L) y(i) -= L;
             }
	  
           printf ("t = %11.4e\n", t);
        }
      printf ("Plot %3d\n", k);

      // Output data
      UnLoad (y, r, v);
      Output(phase[k], data[k], t, r, v);
    }

  return 0;
}

The following routine outputs the simulation data to various data-files.

// Write data to output files

void Output (char* fn1, char* fn2, double t,
	     Array<double,1> r, Array<double,1> v)
{  
  // Write phase-space data
  FILE* file = fopen (fn1, "w");
  for (int i = 0; i < N; i++)
    fprintf (file, "%e %e\n", r(i), v(i));
  fclose (file);

  // Write electric field data
  Array<double,1> ne(J), n(J), phi(J), E(J);  
  Density (r, ne);
  for (int j = 0; j < J; j++)
    n(j) = double (J) * ne(j) / double (N) - 1.;
  double kappa = 2. * M_PI / L; 
  Poisson1D (phi, n, kappa);
  Electric (phi, E);

  file = fopen (fn2, "w");
  for (int j = 0; j < J; j++)
    {
      double x = double (j) * L / double (J);
      fprintf (file, "%e %e %e %e\n", x, ne(j), n(j), E(j));
    }
  double x = L;
  fprintf (file, "%e %e %e %e\n", x, ne(0), n(0), E(0));
  fclose (file);
}

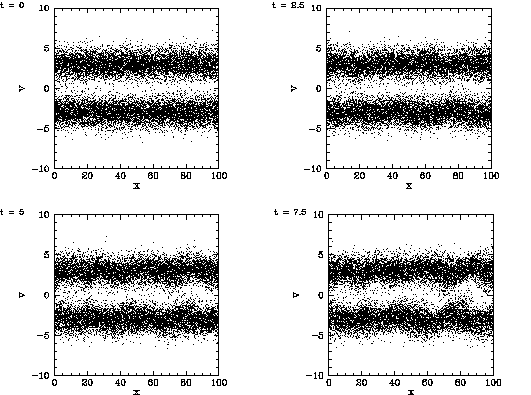
The following routine returns a random velocity distributed on a double Maxwellian distribution function corresponding to two counter-streaming beams. The algorithm used to achieve this is called the rejection method, and will be discussed later in this course.

// Function to distribute electron velocities randomly so as 
// to generate two counter propagating warm beams of thermal
// velocities unity and mean velocities +/- vb.
// Uses rejection method.

double distribution (double vb)
{ 
  // Initialize random number generator
  static int flag = 0;
  if (flag == 0)
    {
      int seed = time (NULL);
      srand (seed);
      flag = 1;
    }

  // Generate random v value
  double fmax = 0.5 * (1. + exp (-2. * vb * vb));
  double vmin = - 5. * vb;
  double vmax = + 5. * vb;
  double v = vmin + (vmax - vmin) * double (rand ()) / double (RAND_MAX);

  // Accept/reject value
  double f = 0.5 * (exp (-(v - vb) * (v - vb) / 2.) +
		    exp (-(v + vb) * (v + vb) / 2.));
  double x = fmax * double (rand ()) / double (RAND_MAX);
  if (x > f) return distribution (vb);
  else return v;
}

The routine below evaluates the electron number density on an evenly spaced mesh given the instantaneous electron coordinates.

// Evaluates electron number density n(0:J-1) from 
// array r(0:N-1) of electron coordinates.

void Density (Array<double,1> r, Array<double,1>& n)
{
  // Initialize 
  double dx = L / double (J);
  n = 0.;

  // Evaluate number density.
  for (int i = 0; i < N; i++)
    {
      int j = int (r(i) / dx);
      double y = r(i) / dx - double (j);
      n(j) += (1. - y) / dx;
      if (j+1 == J) n(0) += y / dx;
      else n(j+1) += y / dx;
    }
}

The following functions are wrapper routines for using the fftw library with periodic functions.

// Functions to calculate Fourier transforms of real data 
// using fftw Fast-Fourier-Transform routine.
// Input/ouput arrays are assumed to be of extent J.

// Calculates Fourier transform of array f in arrays Fr and Fi
void fft_forward (Array<double,1>f, Array<double,1>&Fr, 
      Array<double,1>& Fi)
{
  fftw_complex ff[J], FF[J];

  // Load data
  for (int j = 0; j < J; j++)
    {
      c_re (ff[j]) = f(j); c_im (ff[j]) = 0.;
    }

  // Call fftw routine
  fftw_plan p = fftw_create_plan (J, FFTW_FORWARD, FFTW_ESTIMATE);
  fftw_one (p, ff, FF);
  fftw_destroy_plan (p); 

  // Unload data
  for (int j = 0; j < J; j++)
    {
      Fr(j) = c_re (FF[j]); Fi(j) = c_im (FF[j]);
    }

  // Normalize data
  Fr /= double (J);
  Fi /= double (J);
}

// Calculates inverse Fourier transform of arrays Fr and Fi in array f
void fft_backward (Array<double,1> Fr, Array<double,1> Fi, 
      Array<double,1>& f)
{
  fftw_complex ff[J], FF[J];

  // Load data
  for (int j = 0; j < J; j++)
    {
      c_re (FF[j]) = Fr(j); c_im (FF[j]) = Fi(j);
    }

  // Call fftw routine
  fftw_plan p = fftw_create_plan (J, FFTW_BACKWARD, FFTW_ESTIMATE);
  fftw_one (p, FF, ff);
  fftw_destroy_plan (p); 

  // Unload data
  for (int j = 0; j < J; j++)
      f(j) = c_re (ff[j]); 
}

The following routine solves Poisson's equation in 1-D to find the instantaneous electric potential on a uniform grid.

// Solves 1-d Poisson equation:
//    d^u / dx^2 = v   for  0 <= x <= L
// Periodic boundary conditions:
//    u(x + L) = u(x),  v(x + L) = v(x)
// Arrays u and v assumed to be of length J.
// Now, jth grid point corresponds to
//    x_j = j dx  for j = 0,J-1
// where dx = L / J.
// Also,
//    kappa = 2 pi / L

void Poisson1D (Array<double,1>& u, Array<double,1> v, double kappa)
{
  // Declare local arrays.
  Array<double,1> Vr(J), Vi(J), Ur(J), Ui(J);

  // Fourier transform source term
  fft_forward (v, Vr, Vi);

  // Calculate Fourier transform of u
  Ur(0) = Ui(0) = 0.;
  for (int j = 1; j <= J/2; j++)
    {
      Ur(j) = - Vr(j) / double (j * j) / kappa / kappa;
      Ui(j) = - Vi(j) / double (j * j) / kappa / kappa;
    } 
  for (int j = J/2; j < J; j++)
    {
      Ur(j) = Ur(J-j);
      Ui(j) = - Ui(J-j);
    }

  // Inverse Fourier transform to obtain u
  fft_backward (Ur, Ui, u);
}

The following function evaluates the electric field on a uniform grid from the electric potential.

// Calculate electric field from potential

void Electric (Array<double,1> phi, Array<double,1>& E)
{
  double dx = L / double (J);

  for (int j = 1; j < J-1; j++)
    E(j) = (phi(j-1) - phi(j+1)) / 2. / dx;
  E(0) = (phi(J-1) - phi(1)) / 2. / dx;
  E(J-1) = (phi(J-2) - phi(0)) / 2. / dx;
}

The following routine is the right-hand side routine for the electron equations of motion. Is is designed to be used with the fixed-step RK4 solver described earlier in this course.

// Electron equations of motion:
//    y(0:N-1)  = r_i
//    y(N:2N-1) = dr_i/dt

void rhs_eval (double t, Array<double,1> y, Array<double,1>& dydt)
{
  // Declare local arrays
  Array<double,1> r(N), v(N), rdot(N), vdot(N), r0(N);
  Array<double,1> ne(J), rho(J), phi(J), E(J);

  // Unload data from y
  UnLoad (y, r, v);

  // Make sure all coordinates in range 0 to L
  r0 = r;
  for (int i = 0; i < N; i++)
    {
      if (r0(i) < 0.) r0(i) += L;
      if (r0(i) > L) r0(i) -= L;
    }

  // Calculate electron number density
  Density (r0, ne);

  // Solve Poisson's equation
  double n0 = double (N) / L;
  for (int j = 0; j < J; j++)
    rho(j) = ne(j) / n0 - 1.;
  double kappa = 2. * M_PI / L; 
  Poisson1D (phi, rho, kappa);

  // Calculate electric field
  Electric (phi, E);

  // Equations of motion
  for (int i = 0; i < N; i++)
    {
      double dx = L / double (J);
      int j = int (r0(i) / dx);
      double y = r0(i) / dx - double (j);
      
      double Efield;
      if (j+1 == J)
         Efield = E(j) * (1. - y) + E(0) * y;
      else
         Efield = E(j) * (1. - y) + E(j+1) * y;

      rdot(i) = v(i);
      vdot(i) = - Efield;
    }

  // Load data into dydt
  Load (rdot, vdot, dydt);
}

The following functions load and unload the electron phase-space coordinates into the solution vector y used by the RK4 routine.

// Load particle coordinates into solution vector

void Load (Array<double,1> r, Array<double,1> v, Array<double,1>& y)
{
  for (int i = 0; i < N; i++)
    {
      y(i) = r(i);
      y(N+i) = v(i);
    }
}

// Unload particle coordinates from solution vector

void UnLoad (Array<double,1> y, Array<double,1>& r, Array<double,1>& v)
{
  for (int i = 0; i < N; i++)
    {
      r(i) = y(i);
      v(i) = y(N+i);
    }
}

Figure 89: The electron phase-space distribution evaluated at various times for a 1-dimensional two-stream instability calculation performed with $N=20000$, $J=1000$, $L=100$, $v_b = 3$, and $\delta t = 0.1$.