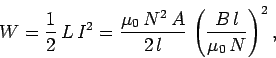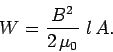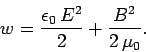Next: The Circuit
Up: Inductance
Previous: Self Inductance
Energy Stored in an Inductor
Suppose that an inductor of inductance 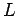 is connected to a
variable DC voltage supply.
The supply is adjusted so as to increase the current
is connected to a
variable DC voltage supply.
The supply is adjusted so as to increase the current 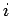 flowing through
the inductor from zero to some final value
flowing through
the inductor from zero to some final value 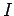 . As the current through the
inductor is ramped up, an emf
. As the current through the
inductor is ramped up, an emf
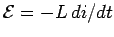 is generated, which acts to oppose the increase in the current. Clearly, work must
be done against this emf by the voltage source in order to establish the
current in the inductor. The work done by the voltage source during a
time interval
is generated, which acts to oppose the increase in the current. Clearly, work must
be done against this emf by the voltage source in order to establish the
current in the inductor. The work done by the voltage source during a
time interval 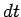 is
is
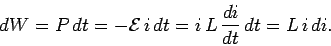 |
(247) |
Here,
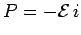 is the instantaneous rate at which the voltage source performs work.
To find the total work
is the instantaneous rate at which the voltage source performs work.
To find the total work 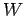 done in establishing the final current
done in establishing the final current 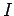 in the
inductor, we must integrate the above expression. Thus,
in the
inductor, we must integrate the above expression. Thus,
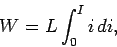 |
(248) |
giving
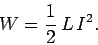 |
(249) |
This energy is actually stored in the magnetic field generated by the current
flowing through the inductor. In a pure inductor, the energy is stored without
loss, and is returned to the rest of the circuit when the current through the
inductor is ramped down, and its associated magnetic field collapses.
Consider a simple solenoid.
Equations (244), (246), and (249) can be combined to give
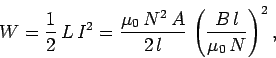 |
(250) |
which reduces to
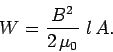 |
(251) |
This represents the energy stored in the magnetic field of the solenoid.
However, the volume of the field-filled core of the solenoid is 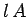 , so the magnetic
energy density (i.e., the energy per unit volume) inside the
solenoid is
, so the magnetic
energy density (i.e., the energy per unit volume) inside the
solenoid is 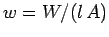 , or
, or
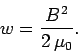 |
(252) |
It turns out that this result is quite general. Thus, we can calculate the
energy content of any magnetic field by dividing space into little cubes
(in each of which the magnetic field is approximately uniform), applying the
above formula to find the energy content of each cube, and summing
the energies thus obtained to find the total energy.
When electric and magnetic fields exist together in space, Eqs. (122) and
(252) can be combined to give an expression for
the total energy stored in the combined
fields per unit volume:
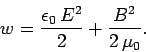 |
(253) |



Next: The Circuit
Up: Inductance
Previous: Self Inductance
Richard Fitzpatrick
2007-07-14