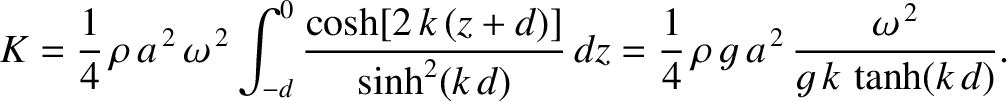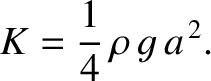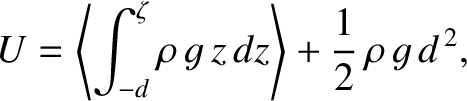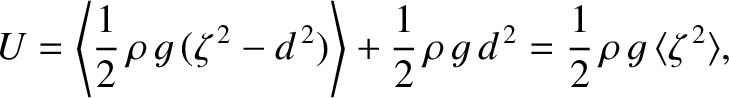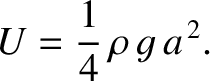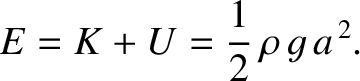Gravity Waves
Consider a stationary body of water, of uniform depth  , located on the surface of the Earth. Let us
find the dispersion relation of a plane wave propagating across the water's surface. Suppose that the Cartesian coordinate
, located on the surface of the Earth. Let us
find the dispersion relation of a plane wave propagating across the water's surface. Suppose that the Cartesian coordinate  measures horizontal distance,
while the coordinate
measures horizontal distance,
while the coordinate  measures vertical height, with
measures vertical height, with 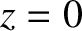 corresponding to the unperturbed surface of the water.
Let there be no variation in the
corresponding to the unperturbed surface of the water.
Let there be no variation in the  -direction. In other words, let the wavefronts of the wave all be parallel to the
-direction. In other words, let the wavefronts of the wave all be parallel to the  -axis. Finally, let
-axis. Finally, let
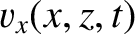 and
and
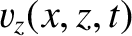 be the perturbed horizontal and
vertical velocity fields of the water due to the wave. It is assumed that there is no
motion in the
be the perturbed horizontal and
vertical velocity fields of the water due to the wave. It is assumed that there is no
motion in the  -direction.
-direction.
Water is essentially incompressible (i.e., its bulk modulus is very large) (Batchelor 2000). Thus, any (low speed) wave disturbance in water is
constrained to preserve the volume of a co-moving volume element. Equivalently, the inflow rate of water into a stationary volume element must match the outflow rate.
Consider a stationary
cubic volume element lying between  and
and 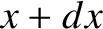 ,
,  and
and 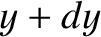 , and
, and  and
and 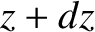 . The element has two faces, of area
. The element has two faces, of area 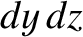 , perpendicular to the
, perpendicular to the  -axis, located at
-axis, located at
 and
and 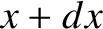 . Water flows into the element through the former face
at the rate
. Water flows into the element through the former face
at the rate
 (i.e., the product of the area of the face and the
normal velocity), and out of the element though the
latter face at the rate
(i.e., the product of the area of the face and the
normal velocity), and out of the element though the
latter face at the rate
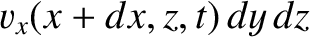 . The element also has two
faces perpendicular to the
. The element also has two
faces perpendicular to the  -axis, but there is no flow through these faces, because
-axis, but there is no flow through these faces, because
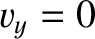 . Finally, the element has two faces, of area
. Finally, the element has two faces, of area 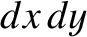 , perpendicular to the
, perpendicular to the  -axis, located at
-axis, located at
 and
and 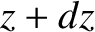 . Water flows into the element through the former face
at the rate
. Water flows into the element through the former face
at the rate
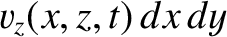 , and out of the element though the
latter face at the rate
, and out of the element though the
latter face at the rate
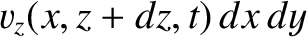 .
Thus, the net rate at which water flows into the element is
.
Thus, the net rate at which water flows into the element is
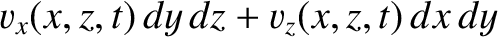 .
Likewise, the net rate at which water flows out of the element is
.
Likewise, the net rate at which water flows out of the element is
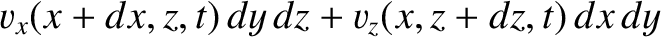 . If the water is to remain incompressible
then the inflow and outflow rates must match. In other words,
. If the water is to remain incompressible
then the inflow and outflow rates must match. In other words,
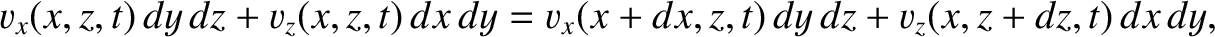 |
(9.258) |
or
![$\displaystyle \left[\frac{v_x(x+dx,z,t)-v_x(x,z,t)}{dx} + \frac{v_z(x,z+dz,t)-v_z(x,z,t)}{dz} \right]dx\,dy\,dz=0.$](img3003.png) |
(9.259) |
Hence, the incompressibility constraint reduces to
 |
(9.260) |
which is consistent with Equation (7.194) in the incompressible limit
 .9.1 Incidentally,
it is reasonable to neglect the finite compressibility of water in this investigation because sound waves (which require finite compressibility)
propagate much faster than surface waves, and, therefore, do not couple to them. (See the two footnotes in this section.)
.9.1 Incidentally,
it is reasonable to neglect the finite compressibility of water in this investigation because sound waves (which require finite compressibility)
propagate much faster than surface waves, and, therefore, do not couple to them. (See the two footnotes in this section.)
Consider the equation of motion of a small volume element of water lying between  and
and 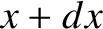 ,
,  and
and 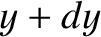 , and
, and  and
and 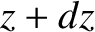 . The mass of this element is
. The mass of this element is
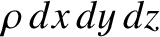 , where
, where
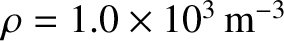 is the uniform mass density of water. Suppose
that
is the uniform mass density of water. Suppose
that 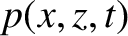 is the pressure in the water, which is assumed to be isotropic (Batchelor 2000).
The net horizontal force on the element is
is the pressure in the water, which is assumed to be isotropic (Batchelor 2000).
The net horizontal force on the element is
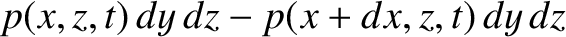 (because force is pressure times area, and the external pressure forces
acting on the element are directed inward normal to its surface). Hence, the element's horizontal equation of motion is
(because force is pressure times area, and the external pressure forces
acting on the element are directed inward normal to its surface). Hence, the element's horizontal equation of motion is
![$\displaystyle \rho\,dx\,dy\,dz\,\frac{\partial v_x(x,z,t)}{\partial t} = -\left[\frac{p(x+dx,z,t)-p(x,z,t)}{dx}\right]dx\,dy\,dz,$](img3013.png) |
(9.261) |
which reduces to
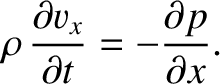 |
(9.262) |
[See Equation (7.192).]
The vertical equation of motion is similar, except that the element is subject to
a downward acceleration,
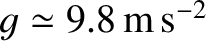 , due to gravity. Hence, we obtain
, due to gravity. Hence, we obtain
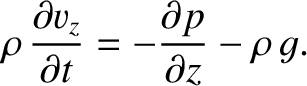 |
(9.263) |
[See Equation (7.193).]
We can write
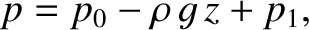 |
(9.264) |
where 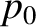 is atmospheric pressure (i.e., the air pressure just above the surface of the water), and
is atmospheric pressure (i.e., the air pressure just above the surface of the water), and 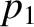 is the pressure perturbation due to the wave. In the absence of
the wave, the water pressure at a depth
is the pressure perturbation due to the wave. In the absence of
the wave, the water pressure at a depth 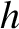 below the surface is
below the surface is
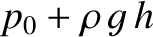 (Batchelor 2000).
Substitution into Equations (9.262) and (9.263) yields
It follows that
(Batchelor 2000).
Substitution into Equations (9.262) and (9.263) yields
It follows that
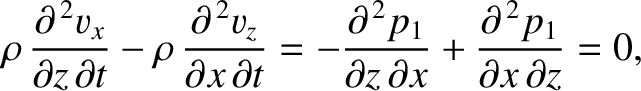 |
(9.267) |
which implies that
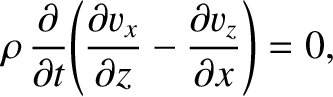 |
(9.268) |
or
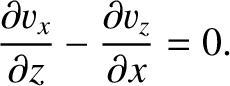 |
(9.269) |
(Actually, this quantity could be non-zero and constant in time, but this is
not consistent with an oscillating wave-like solution.)
Equation (9.269) is automatically satisfied by writing the fluid velocity in terms of a velocity potential; that is,
(See Section 7.12.)
Equation (9.260) then gives 9.2
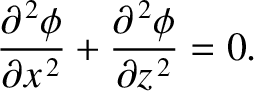 |
(9.272) |
Finally, Equations (9.265) and (9.266) yield
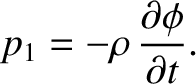 |
(9.273) |
As we have just demonstrated, surface waves in water are governed by Equation (9.272),
which is known as Laplace's equation. We now need to derive the physical
constraints that must be satisfied by the solution to this equation at
the water's upper and lower boundaries. The water is bounded from below by a
solid surface located at 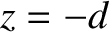 . Assuming that the water always remains in contact
with this surface, the appropriate physical constraint at the lower boundary is
. Assuming that the water always remains in contact
with this surface, the appropriate physical constraint at the lower boundary is
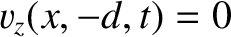 (i.e., there is no vertical motion of the water at the lower boundary), or
(i.e., there is no vertical motion of the water at the lower boundary), or
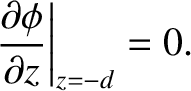 |
(9.274) |
The physical constraint at the water's upper boundary is a little more complicated, because this boundary is a free surface.
Let
 represent the vertical displacement of the water's surface. It
follows that
represent the vertical displacement of the water's surface. It
follows that
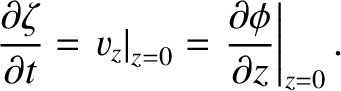 |
(9.275) |
The physical constraint at the surface is that the water pressure is equal to atmospheric pressure, because there cannot be a pressure discontinuity across a free surface (in the absence of surface tension).
Thus, it follows from Equation (9.264) that
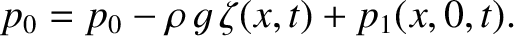 |
(9.276) |
Finally, differentiating with respect to  , and making use of Equations (9.273) and (9.275), we obtain
, and making use of Equations (9.273) and (9.275), we obtain
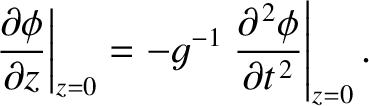 |
(9.277) |
Hence, the problem boils down to solving Laplace's equation, (9.272), subject
to the physical constraints (9.274) and (9.277).
Let us search for a propagating wave-like solution of Equation (9.272) of the form
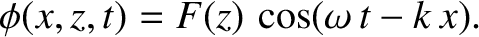 |
(9.278) |
Substitution into Equation (9.272) yields
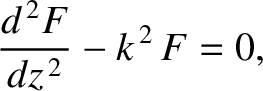 |
(9.279) |
whose independent solutions are
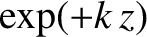 and
and
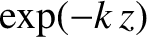 .
Hence, the most general wave-like solution to Laplace's equation is
.
Hence, the most general wave-like solution to Laplace's equation is
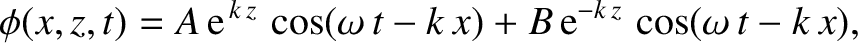 |
(9.280) |
where  and
and 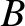 are arbitrary constants. The boundary condition (9.274)
is satisfied provided that
are arbitrary constants. The boundary condition (9.274)
is satisfied provided that
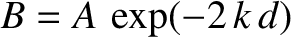 , giving
, giving
![$\displaystyle \phi(x,z,t) = A\left[{\rm e}^{\,k\,z}\ + {\rm e}^{-k\,(z+2\,d)}\right]\cos(\omega\,t-k\,x),$](img3044.png) |
(9.281) |
The boundary condition (9.277) yields
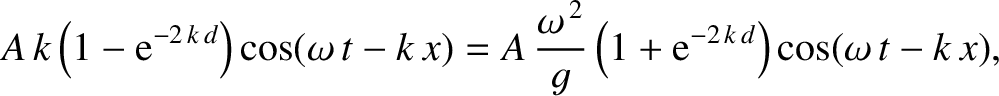 |
(9.282) |
which reduces to the dispersion relation
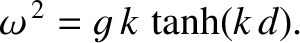 |
(9.283) |
The type of wave just described is generally known
as a gravity wave.
We conclude that a gravity wave of arbitrary wavenumber  , propagating horizontally through water of depth
, propagating horizontally through water of depth  , has a phase velocity
, has a phase velocity
![$\displaystyle v_p = \frac{\omega}{k}=(g\,d)^{1/2}\left[\frac{\tanh(k\,d)}{k\,d}\right]^{1/2}.$](img3047.png) |
(9.284) |
Moreover, the ratio of the group to the phase velocity is
![$\displaystyle \frac{v_g}{v_p} =\frac{k}{\omega}\,\frac{d\omega}{dk}=\frac{1}{2}\left[1+\frac{2\,k\,d}{\sinh(2\,k\,d)}\right].$](img3048.png) |
(9.285) |
Finally, the
velocity fields associated with a gravity wave of surface amplitude 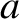 are
are
In shallow water (i.e., 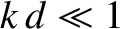 ), Equation (9.283) reduces to
the linear dispersion relation
), Equation (9.283) reduces to
the linear dispersion relation
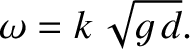 |
(9.288) |
Here, use has been made of the small argument expansion
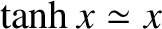 for
for 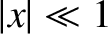 . (See Appendix B.)
It follows that gravity waves in shallow water are non-dispersive in nature, and
propagate at the phase velocity
. (See Appendix B.)
It follows that gravity waves in shallow water are non-dispersive in nature, and
propagate at the phase velocity
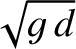 . On the other hand, in deep water (i.e.,
. On the other hand, in deep water (i.e., 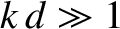 ), Equation (9.283) reduces to
the nonlinear dispersion relation
), Equation (9.283) reduces to
the nonlinear dispersion relation
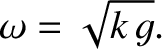 |
(9.289) |
Here, use has been made of the large argument expansion
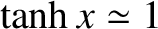 for
for 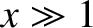 .
We conclude that gravity waves in deep water are dispersive in nature. The
phase velocity of the waves is
.
We conclude that gravity waves in deep water are dispersive in nature. The
phase velocity of the waves is
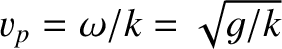 , whereas the
group velocity is
, whereas the
group velocity is
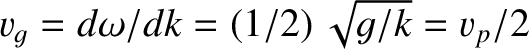 . In other
words, the group velocity is half the phase velocity, and is largest for long wavelength
(i.e., small
. In other
words, the group velocity is half the phase velocity, and is largest for long wavelength
(i.e., small  ) waves.
) waves.
The mean kinetic energy per unit surface area associated with a gravity wave is defined
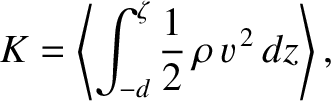 |
(9.290) |
where
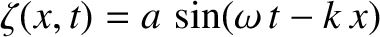 |
(9.291) |
is the vertical displacement at the surface, and
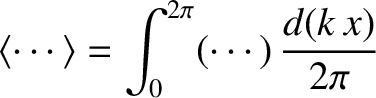 |
(9.292) |
represents an average over a wavelength. Given that
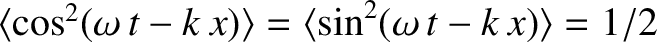 , it follows from Equations (9.286) and (9.287) that, to second order in
, it follows from Equations (9.286) and (9.287) that, to second order in  ,
,
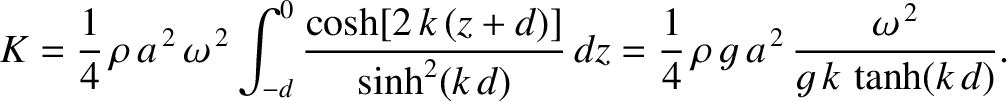 |
(9.293) |
Making use of the general dispersion relation (9.283), we obtain
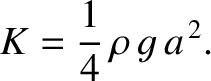 |
(9.294) |
The mean potential energy perturbation per unit surface area associated with a gravity wave is defined
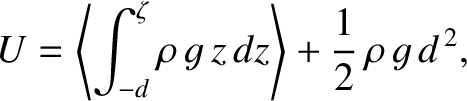 |
(9.295) |
which yields
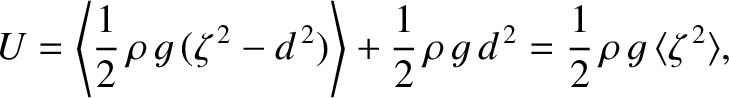 |
(9.296) |
or
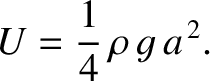 |
(9.297) |
In other words, the mean potential energy per unit surface area of a gravity wave is equal to its mean kinetic
energy per unit surface area.
Finally, the mean total energy per unit surface area associated with a gravity wave is
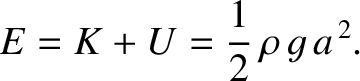 |
(9.298) |
This energy depends on the wave amplitude at the surface, but is independent of the wavelength, or the water depth.
 , located on the surface of the Earth. Let us
find the dispersion relation of a plane wave propagating across the water's surface. Suppose that the Cartesian coordinate
, located on the surface of the Earth. Let us
find the dispersion relation of a plane wave propagating across the water's surface. Suppose that the Cartesian coordinate  measures horizontal distance,
while the coordinate
measures horizontal distance,
while the coordinate  measures vertical height, with
measures vertical height, with 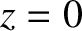 corresponding to the unperturbed surface of the water.
Let there be no variation in the
corresponding to the unperturbed surface of the water.
Let there be no variation in the  -direction. In other words, let the wavefronts of the wave all be parallel to the
-direction. In other words, let the wavefronts of the wave all be parallel to the  -axis. Finally, let
-axis. Finally, let
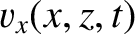 and
and
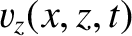 be the perturbed horizontal and
vertical velocity fields of the water due to the wave. It is assumed that there is no
motion in the
be the perturbed horizontal and
vertical velocity fields of the water due to the wave. It is assumed that there is no
motion in the  -direction.
-direction.
 and
and 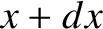 ,
,  and
and 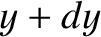 , and
, and  and
and 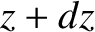 . The element has two faces, of area
. The element has two faces, of area 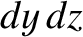 , perpendicular to the
, perpendicular to the  -axis, located at
-axis, located at
 and
and 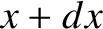 . Water flows into the element through the former face
at the rate
. Water flows into the element through the former face
at the rate
 (i.e., the product of the area of the face and the
normal velocity), and out of the element though the
latter face at the rate
(i.e., the product of the area of the face and the
normal velocity), and out of the element though the
latter face at the rate
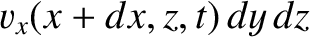 . The element also has two
faces perpendicular to the
. The element also has two
faces perpendicular to the  -axis, but there is no flow through these faces, because
-axis, but there is no flow through these faces, because
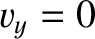 . Finally, the element has two faces, of area
. Finally, the element has two faces, of area 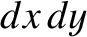 , perpendicular to the
, perpendicular to the  -axis, located at
-axis, located at
 and
and 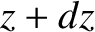 . Water flows into the element through the former face
at the rate
. Water flows into the element through the former face
at the rate
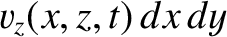 , and out of the element though the
latter face at the rate
, and out of the element though the
latter face at the rate
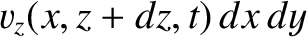 .
Thus, the net rate at which water flows into the element is
.
Thus, the net rate at which water flows into the element is
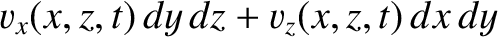 .
Likewise, the net rate at which water flows out of the element is
.
Likewise, the net rate at which water flows out of the element is
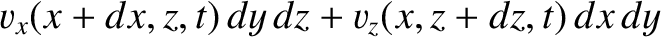 . If the water is to remain incompressible
then the inflow and outflow rates must match. In other words,
. If the water is to remain incompressible
then the inflow and outflow rates must match. In other words,
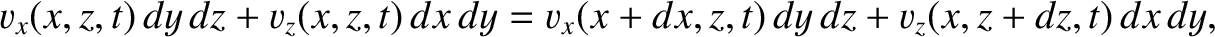
![$\displaystyle \left[\frac{v_x(x+dx,z,t)-v_x(x,z,t)}{dx} + \frac{v_z(x,z+dz,t)-v_z(x,z,t)}{dz} \right]dx\,dy\,dz=0.$](img3003.png)
 .9.1 Incidentally,
it is reasonable to neglect the finite compressibility of water in this investigation because sound waves (which require finite compressibility)
propagate much faster than surface waves, and, therefore, do not couple to them. (See the two footnotes in this section.)
.9.1 Incidentally,
it is reasonable to neglect the finite compressibility of water in this investigation because sound waves (which require finite compressibility)
propagate much faster than surface waves, and, therefore, do not couple to them. (See the two footnotes in this section.)
 and
and  ,
,  and
and  , and
, and  and
and 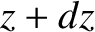 . The mass of this element is
. The mass of this element is
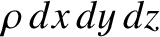 , where
, where
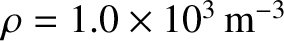 is the uniform mass density of water. Suppose
that
is the uniform mass density of water. Suppose
that 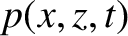 is the pressure in the water, which is assumed to be isotropic (Batchelor 2000).
The net horizontal force on the element is
is the pressure in the water, which is assumed to be isotropic (Batchelor 2000).
The net horizontal force on the element is
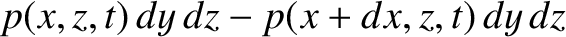 (because force is pressure times area, and the external pressure forces
acting on the element are directed inward normal to its surface). Hence, the element's horizontal equation of motion is
(because force is pressure times area, and the external pressure forces
acting on the element are directed inward normal to its surface). Hence, the element's horizontal equation of motion is
![$\displaystyle \rho\,dx\,dy\,dz\,\frac{\partial v_x(x,z,t)}{\partial t} = -\left[\frac{p(x+dx,z,t)-p(x,z,t)}{dx}\right]dx\,dy\,dz,$](img3013.png)
 , due to gravity. Hence, we obtain
[See Equation (7.193).]
, due to gravity. Hence, we obtain
[See Equation (7.193).]
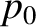 is atmospheric pressure (i.e., the air pressure just above the surface of the water), and
is atmospheric pressure (i.e., the air pressure just above the surface of the water), and 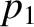 is the pressure perturbation due to the wave. In the absence of
the wave, the water pressure at a depth
is the pressure perturbation due to the wave. In the absence of
the wave, the water pressure at a depth 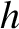 below the surface is
below the surface is
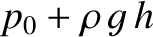 (Batchelor 2000).
Substitution into Equations (9.262) and (9.263) yields
It follows that
(Batchelor 2000).
Substitution into Equations (9.262) and (9.263) yields
It follows that
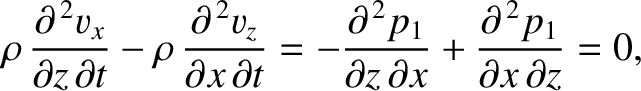
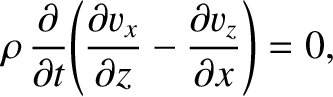
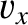
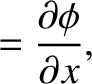
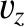
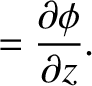
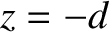 . Assuming that the water always remains in contact
with this surface, the appropriate physical constraint at the lower boundary is
. Assuming that the water always remains in contact
with this surface, the appropriate physical constraint at the lower boundary is
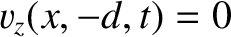 (i.e., there is no vertical motion of the water at the lower boundary), or
(i.e., there is no vertical motion of the water at the lower boundary), or
 represent the vertical displacement of the water's surface. It
follows that
The physical constraint at the surface is that the water pressure is equal to atmospheric pressure, because there cannot be a pressure discontinuity across a free surface (in the absence of surface tension).
Thus, it follows from Equation (9.264) that
Finally, differentiating with respect to
represent the vertical displacement of the water's surface. It
follows that
The physical constraint at the surface is that the water pressure is equal to atmospheric pressure, because there cannot be a pressure discontinuity across a free surface (in the absence of surface tension).
Thus, it follows from Equation (9.264) that
Finally, differentiating with respect to  , and making use of Equations (9.273) and (9.275), we obtain
Hence, the problem boils down to solving Laplace's equation, (9.272), subject
to the physical constraints (9.274) and (9.277).
, and making use of Equations (9.273) and (9.275), we obtain
Hence, the problem boils down to solving Laplace's equation, (9.272), subject
to the physical constraints (9.274) and (9.277).
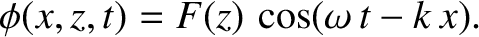
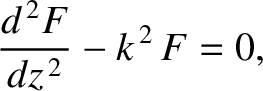
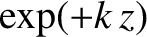 and
and
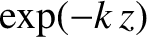 .
Hence, the most general wave-like solution to Laplace's equation is
.
Hence, the most general wave-like solution to Laplace's equation is
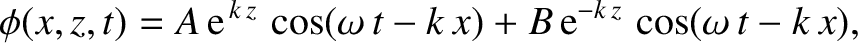
 and
and 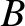 are arbitrary constants. The boundary condition (9.274)
is satisfied provided that
are arbitrary constants. The boundary condition (9.274)
is satisfied provided that
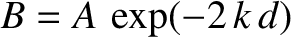 , giving
The boundary condition (9.277) yields
, giving
The boundary condition (9.277) yields
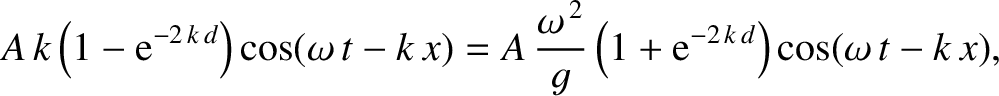
 , propagating horizontally through water of depth
, propagating horizontally through water of depth  , has a phase velocity
Moreover, the ratio of the group to the phase velocity is
Finally, the
velocity fields associated with a gravity wave of surface amplitude
, has a phase velocity
Moreover, the ratio of the group to the phase velocity is
Finally, the
velocity fields associated with a gravity wave of surface amplitude  are
are
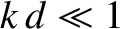 ), Equation (9.283) reduces to
the linear dispersion relation
), Equation (9.283) reduces to
the linear dispersion relation
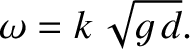
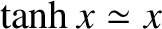 for
for 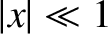 . (See Appendix B.)
It follows that gravity waves in shallow water are non-dispersive in nature, and
propagate at the phase velocity
. (See Appendix B.)
It follows that gravity waves in shallow water are non-dispersive in nature, and
propagate at the phase velocity
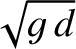 . On the other hand, in deep water (i.e.,
. On the other hand, in deep water (i.e., 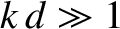 ), Equation (9.283) reduces to
the nonlinear dispersion relation
), Equation (9.283) reduces to
the nonlinear dispersion relation
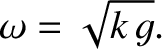
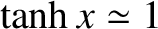 for
for 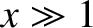 .
We conclude that gravity waves in deep water are dispersive in nature. The
phase velocity of the waves is
.
We conclude that gravity waves in deep water are dispersive in nature. The
phase velocity of the waves is
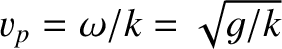 , whereas the
group velocity is
, whereas the
group velocity is
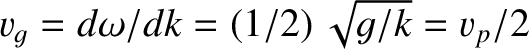 . In other
words, the group velocity is half the phase velocity, and is largest for long wavelength
(i.e., small
. In other
words, the group velocity is half the phase velocity, and is largest for long wavelength
(i.e., small  ) waves.
) waves.
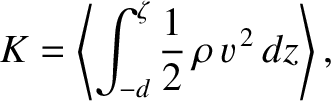
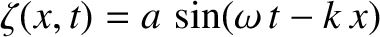
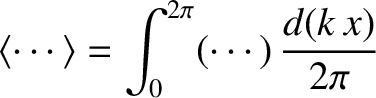
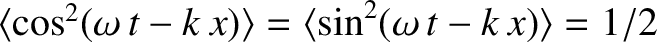 , it follows from Equations (9.286) and (9.287) that, to second order in
, it follows from Equations (9.286) and (9.287) that, to second order in 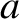 ,
,