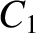Alpha Decay
Many types of heavy atomic nuclei spontaneously decay to produce daughter nuclei
via the emission of  -particles (i.e., helium nuclei) of some characteristic energy.
This process is known as
-particles (i.e., helium nuclei) of some characteristic energy.
This process is known as
 -decay. Let us investigate the
-decay. Let us investigate the  -decay of a particular type of atomic nucleus of radius
-decay of a particular type of atomic nucleus of radius 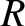 , charge-number
, charge-number 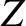 ,
and mass-number
,
and mass-number  . Such a nucleus thus decays to produce a daughter
nucleus of charge-number
. Such a nucleus thus decays to produce a daughter
nucleus of charge-number 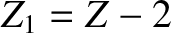 and mass-number
and mass-number 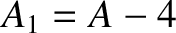 ,
and an
,
and an  -particle of charge-number
-particle of charge-number  and mass-number
and mass-number
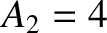 . Let the characteristic energy of the
. Let the characteristic energy of the  -particle
be
-particle
be 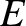 . Incidentally, nuclear radii
are found to satisfy the empirical formula
. Incidentally, nuclear radii
are found to satisfy the empirical formula
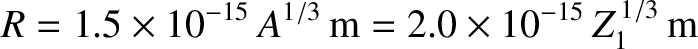 |
(11.132) |
for 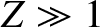 (Park 1974).
(Park 1974).
In 1928, George Gamov proposed a very successful theory of  -decay,
according to which the
-decay,
according to which the  -particle moves freely inside the nucleus, and is emitted after tunneling through the
potential barrier between itself and the daughter nucleus. In other words,
the
-particle moves freely inside the nucleus, and is emitted after tunneling through the
potential barrier between itself and the daughter nucleus. In other words,
the  -particle, whose energy is
-particle, whose energy is 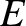 , is trapped in a potential well of radius
, is trapped in a potential well of radius 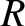 by the
potential barrier
by the
potential barrier
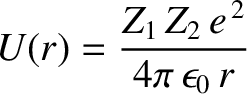 |
(11.133) |
for  . Here,
. Here, 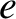 is the magnitude of the electron charge.
is the magnitude of the electron charge.
Making use of the WKB approximation (and neglecting the fact
that 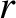 is a radial, rather than a Cartesian, coordinate), the probability
of the
is a radial, rather than a Cartesian, coordinate), the probability
of the  -particle tunneling through the barrier is
-particle tunneling through the barrier is
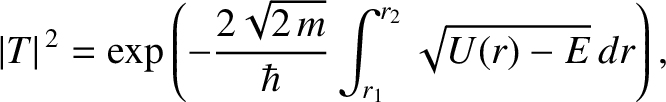 |
(11.134) |
where 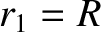 and
and
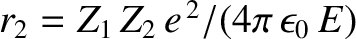 . Here,
. Here,
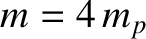 is the
is the  -particle mass, and
-particle mass, and 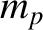 is the proton mass. The previous expression
reduces to
is the proton mass. The previous expression
reduces to
![$\displaystyle \vert T\vert^{\,2} = \exp\left[-2\sqrt{2}\,\beta \int_{1}^{E_c/E}\left(\frac{1}{y}-\frac{E}{E_c}\right)^{1/2} dy\right],$](img4103.png) |
(11.135) |
where
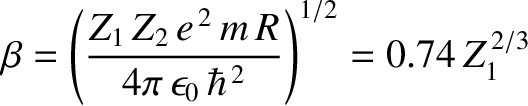 |
(11.136) |
is a dimensionless constant, and
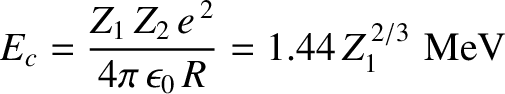 |
(11.137) |
is the characteristic energy the  -particle would need in order to escape
from the nucleus without tunneling. Of course,
-particle would need in order to escape
from the nucleus without tunneling. Of course, 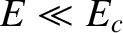 .
It is easily demonstrated that
.
It is easily demonstrated that
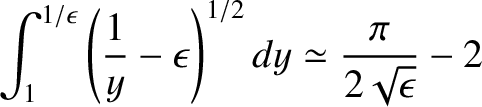 |
(11.138) |
when
 .
Hence.
.
Hence.
![$\displaystyle \vert T\vert^{\,2} \simeq \exp\left[-2\sqrt{2}\,\beta\left(\frac{\pi}{2}\sqrt{\frac{E_c}{E}}-2\right)\right].$](img4109.png) |
(11.139) |
Now, the  -particle moves inside the nucleus at the characteristic
velocity
-particle moves inside the nucleus at the characteristic
velocity
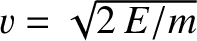 . It follows that the particle bounces backward
and forward within the nucleus at the frequency
. It follows that the particle bounces backward
and forward within the nucleus at the frequency
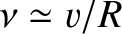 , giving
, giving
 |
(11.140) |
for a 1 MeV  -particle trapped inside a typical heavy nucleus of radius
-particle trapped inside a typical heavy nucleus of radius 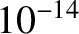 m.
Thus, the
m.
Thus, the  -particle effectively attempts to tunnel through the potential
barrier
-particle effectively attempts to tunnel through the potential
barrier  times a second. If each of these attempts has a probability
times a second. If each of these attempts has a probability
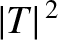 of succeeding then the probability of decay per unit time
is
of succeeding then the probability of decay per unit time
is
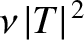 . Hence, if there are
. Hence, if there are  intact nuclei at time
intact nuclei at time  then
there are only
then
there are only 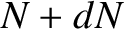 at time
at time 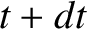 , where
, where
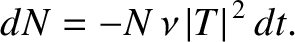 |
(11.141) |
This expression can be integrated to give
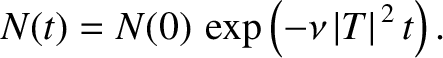 |
(11.142) |
The half-life,  , is defined as the time which must elapse
in order for half of the nuclei originally present to decay. It follows from
the previous formula that
, is defined as the time which must elapse
in order for half of the nuclei originally present to decay. It follows from
the previous formula that
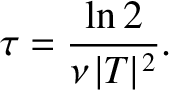 |
(11.143) |
Note that the half-life is independent of  .
.
Finally, making use of the previous results, we obtain
![$\displaystyle \log_{10}[\tau ({\rm yr})] = -C_1 - C_2\,Z_1^{\,2/3} + C_3\,\frac{Z_1}{\sqrt{E({\rm MeV})}},$](img4122.png) |
(11.144) |
where
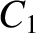 |
 |
(11.145) |
 |
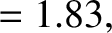 |
(11.146) |
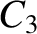 |
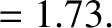 |
(11.147) |
Figure 11.13:
The experimentally determined half-life,
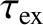 , of various atomic nuclei that decay via
, of various atomic nuclei that decay via  -emission versus the best-fit theoretical half-life
-emission versus the best-fit theoretical half-life
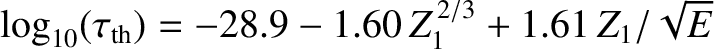 . Both half-lives are measured in years. Here,
. Both half-lives are measured in years. Here, 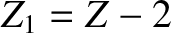 , where
, where 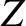 is the charge-number of the nucleus, and
is the charge-number of the nucleus, and 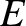 the characteristic energy of the emitted
the characteristic energy of the emitted  -particle in MeV. In
order of increasing half-life, the points correspond to the
following nuclei: Rn 215, Po 214, Po 216, Po 197, Fm 250, Ac 225, U 230, U 232, U 234, Gd 150, U 236, U 238, Pt 190, Gd 152, Nd 144. (Data obtained from International Atomic Energy Agency, Nuclear Data Center.)
-particle in MeV. In
order of increasing half-life, the points correspond to the
following nuclei: Rn 215, Po 214, Po 216, Po 197, Fm 250, Ac 225, U 230, U 232, U 234, Gd 150, U 236, U 238, Pt 190, Gd 152, Nd 144. (Data obtained from International Atomic Energy Agency, Nuclear Data Center.)
|
|
The half-life,  , the daughter charge-number,
, the daughter charge-number, 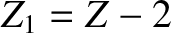 , and
the
, and
the  -particle energy,
-particle energy, 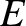 , for atomic nuclei that undergo
, for atomic nuclei that undergo  -decay
are indeed found to satisfy a relationship of the form (11.144). The
best fit to the data (see Figure 11.13) is obtained using
-decay
are indeed found to satisfy a relationship of the form (11.144). The
best fit to the data (see Figure 11.13) is obtained using
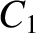 |
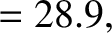 |
(11.148) |
 |
 |
(11.149) |
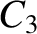 |
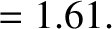 |
(11.150) |
 -particles (i.e., helium nuclei) of some characteristic energy.
This process is known as
-particles (i.e., helium nuclei) of some characteristic energy.
This process is known as
 -decay. Let us investigate the
-decay. Let us investigate the  -decay of a particular type of atomic nucleus of radius
-decay of a particular type of atomic nucleus of radius 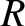 , charge-number
, charge-number 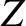 ,
and mass-number
,
and mass-number  . Such a nucleus thus decays to produce a daughter
nucleus of charge-number
. Such a nucleus thus decays to produce a daughter
nucleus of charge-number 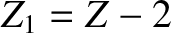 and mass-number
and mass-number 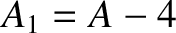 ,
and an
,
and an  -particle of charge-number
-particle of charge-number  and mass-number
and mass-number
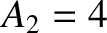 . Let the characteristic energy of the
. Let the characteristic energy of the  -particle
be
-particle
be 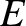 . Incidentally, nuclear radii
are found to satisfy the empirical formula
. Incidentally, nuclear radii
are found to satisfy the empirical formula
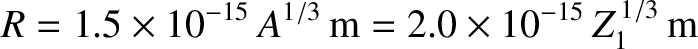
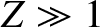 (Park 1974).
(Park 1974).
 -decay,
according to which the
-decay,
according to which the  -particle moves freely inside the nucleus, and is emitted after tunneling through the
potential barrier between itself and the daughter nucleus. In other words,
the
-particle moves freely inside the nucleus, and is emitted after tunneling through the
potential barrier between itself and the daughter nucleus. In other words,
the  -particle, whose energy is
-particle, whose energy is 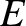 , is trapped in a potential well of radius
, is trapped in a potential well of radius 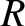 by the
potential barrier
by the
potential barrier
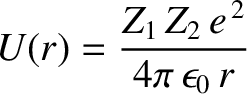
 . Here,
. Here, 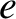 is the magnitude of the electron charge.
is the magnitude of the electron charge.
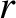 is a radial, rather than a Cartesian, coordinate), the probability
of the
is a radial, rather than a Cartesian, coordinate), the probability
of the  -particle tunneling through the barrier is
-particle tunneling through the barrier is
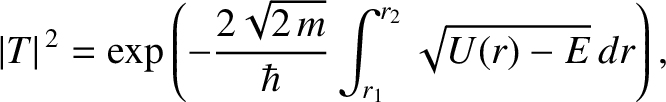
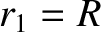 and
and
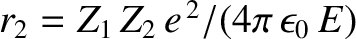 . Here,
. Here,
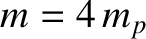 is the
is the  -particle mass, and
-particle mass, and 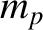 is the proton mass. The previous expression
reduces to
is the proton mass. The previous expression
reduces to
![$\displaystyle \vert T\vert^{\,2} = \exp\left[-2\sqrt{2}\,\beta \int_{1}^{E_c/E}\left(\frac{1}{y}-\frac{E}{E_c}\right)^{1/2} dy\right],$](img4103.png)
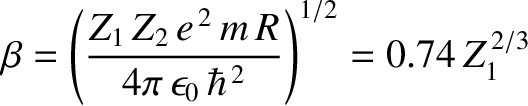
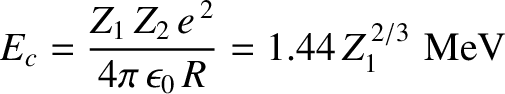
 -particle would need in order to escape
from the nucleus without tunneling. Of course,
-particle would need in order to escape
from the nucleus without tunneling. Of course, 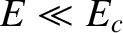 .
It is easily demonstrated that
.
It is easily demonstrated that
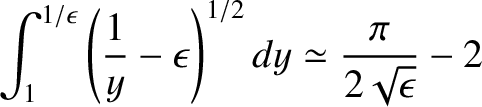
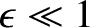 .
Hence.
.
Hence.
![$\displaystyle \vert T\vert^{\,2} \simeq \exp\left[-2\sqrt{2}\,\beta\left(\frac{\pi}{2}\sqrt{\frac{E_c}{E}}-2\right)\right].$](img4109.png)
 -particle moves inside the nucleus at the characteristic
velocity
-particle moves inside the nucleus at the characteristic
velocity
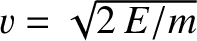 . It follows that the particle bounces backward
and forward within the nucleus at the frequency
. It follows that the particle bounces backward
and forward within the nucleus at the frequency
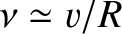 , giving
, giving

 -particle trapped inside a typical heavy nucleus of radius
-particle trapped inside a typical heavy nucleus of radius 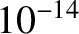 m.
Thus, the
m.
Thus, the  -particle effectively attempts to tunnel through the potential
barrier
-particle effectively attempts to tunnel through the potential
barrier  times a second. If each of these attempts has a probability
times a second. If each of these attempts has a probability
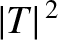 of succeeding then the probability of decay per unit time
is
of succeeding then the probability of decay per unit time
is
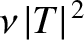 . Hence, if there are
. Hence, if there are  intact nuclei at time
intact nuclei at time  then
there are only
then
there are only 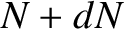 at time
at time 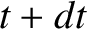 , where
, where
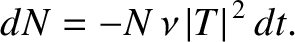
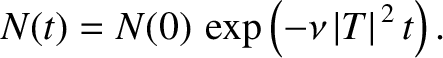
 , is defined as the time which must elapse
in order for half of the nuclei originally present to decay. It follows from
the previous formula that
, is defined as the time which must elapse
in order for half of the nuclei originally present to decay. It follows from
the previous formula that
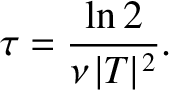
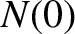 .
.
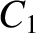
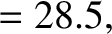

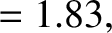
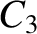
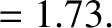
![\includegraphics[width=0.85\textwidth]{Chapter11/fig11_13.eps}](img4129.png)
 , the daughter charge-number,
, the daughter charge-number, 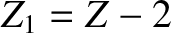 , and
the
, and
the  -particle energy,
-particle energy, 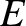 , for atomic nuclei that undergo
, for atomic nuclei that undergo  -decay
are indeed found to satisfy a relationship of the form (11.144). The
best fit to the data (see Figure 11.13) is obtained using
-decay
are indeed found to satisfy a relationship of the form (11.144). The
best fit to the data (see Figure 11.13) is obtained using