Next: Heisenberg's Uncertainty Principle Up: Wave Mechanics Previous: Probability Interpretation of Wavefunction Contents
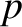 and energy
and energy 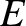 , moving in free space along the
, moving in free space along the  -axis, can be written
where
-axis, can be written
where
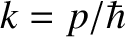 ,
,
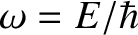 , and
, and
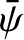 is a complex constant. Here,
is a complex constant. Here,  and
and
 are linked via the matter-wave dispersion relation (11.24). Expression (11.37) represents a plane wave that propagates in the
are linked via the matter-wave dispersion relation (11.24). Expression (11.37) represents a plane wave that propagates in the  -direction
with the phase velocity
-direction
with the phase velocity
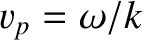 . However, it follows from Equation (11.25) that this phase velocity is only half of the classical velocity of a massive particle.
. However, it follows from Equation (11.25) that this phase velocity is only half of the classical velocity of a massive particle.
According to the discussion in the previous section, the most reasonable physical interpretation of the wavefunction is that
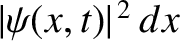 is proportional to (assuming that the wavefunction is not
properly normalized) the probability of finding the particle
between
is proportional to (assuming that the wavefunction is not
properly normalized) the probability of finding the particle
between  and
and 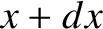 at time
at time  . However, the modulus squared of the wavefunction (11.37) is
. However, the modulus squared of the wavefunction (11.37) is
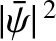 , which is a constant that depends on neither
, which is a constant that depends on neither  nor
nor  . In other words, the previous wavefunction represents a particle
that is equally likely to be found anywhere on the
. In other words, the previous wavefunction represents a particle
that is equally likely to be found anywhere on the  -axis at all times.
Hence, the fact that this wavefunction propagates at
a phase velocity that does not correspond to the classical particle velocity has no observable consequences.
-axis at all times.
Hence, the fact that this wavefunction propagates at
a phase velocity that does not correspond to the classical particle velocity has no observable consequences.
How can we write the wavefunction of a particle that is localized
in  ? In other words, a particle that is more likely to be found at some
positions on the
? In other words, a particle that is more likely to be found at some
positions on the  -axis than at others. It turns out that we can achieve this goal by forming
a linear combination of plane waves of different wavenumbers;
that is,
-axis than at others. It turns out that we can achieve this goal by forming
a linear combination of plane waves of different wavenumbers;
that is,
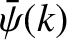 represents the complex amplitude of plane waves of wavenumber
represents the complex amplitude of plane waves of wavenumber  within this combination. In writing the previous expression,
we are relying on the assumption that matter waves are superposable.
In other words, it is possible to add two valid wave solutions to form a third valid wave solution.
The ultimate justification for this assumption is that matter waves
satisfy the linear wave equation (11.23).
within this combination. In writing the previous expression,
we are relying on the assumption that matter waves are superposable.
In other words, it is possible to add two valid wave solutions to form a third valid wave solution.
The ultimate justification for this assumption is that matter waves
satisfy the linear wave equation (11.23).
There is a fundamental mathematical theorem, known as Fourier's theorem (see Section 8.2 and Exercise 11), which states that if
then Here,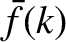 is known as the Fourier transform of the
function
is known as the Fourier transform of the
function  . We can use Fourier's theorem to find the
. We can use Fourier's theorem to find the  -space function
-space function
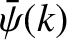 that generates any given
that generates any given  -space wavefunction
-space wavefunction 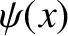 at a given time.
at a given time.
For instance, suppose that at 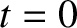 the wavefunction of our particle takes the
form
the wavefunction of our particle takes the
form
 -coordinate is
This particular distribution is called a Gaussian distribution (see Section 8.2), and is plotted in Figure 11.3.
It can be seen that a measurement of the particle's position is most
likely to yield the value
-coordinate is
This particular distribution is called a Gaussian distribution (see Section 8.2), and is plotted in Figure 11.3.
It can be seen that a measurement of the particle's position is most
likely to yield the value 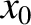 , and very
unlikely to yield a value which differs from
, and very
unlikely to yield a value which differs from 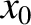 by more than
by more than
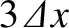 . Thus, Equation (11.41) is the wavefunction of a particle
that is initially localized in some region of
. Thus, Equation (11.41) is the wavefunction of a particle
that is initially localized in some region of  -space, centered on
-space, centered on 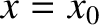 , whose width is
of order
, whose width is
of order
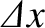 . This type of wavefunction is
known as a wave packet. However, a wave packet is just another name for a wave pulse. (See Chapter 8.)
. This type of wavefunction is
known as a wave packet. However, a wave packet is just another name for a wave pulse. (See Chapter 8.)
According to Equation (11.38),
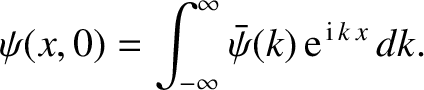 |
(11.43) |
![$\displaystyle \bar{\psi}(k) \propto
{\rm e}^{-{\rm i}\,(k-k_0)\,x_0}\int_{-\inf...
...i}\,(k-k_0)\,(x-x_0) - \frac{(x-x_0)^{\,2}}{4\,({\mit\Delta}x)^{\,2}}\right]dx.$](img3881.png) |
(11.45) |
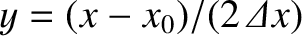 , the previous expression reduces to
, the previous expression reduces to
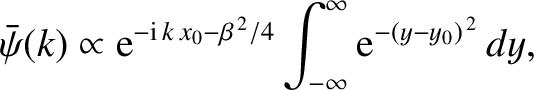 |
(11.46) |
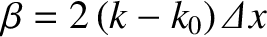 and
and
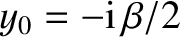 . The integral in the previous equation is now just a number,
as can easily be seen by making the second change of variable
. The integral in the previous equation is now just a number,
as can easily be seen by making the second change of variable 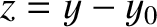 .
Hence, we deduce that
where
.
Hence, we deduce that
where
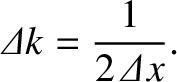 |
(11.48) |
If
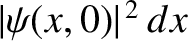 is proportional to the probability of a measurement of the
particle's position yielding a value in the range
is proportional to the probability of a measurement of the
particle's position yielding a value in the range  to
to 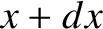 at time
at time 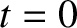 then it stands to reason that
then it stands to reason that
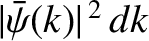 is proportional to the probability of a measurement of the
particle's wavenumber yielding a value in the range
is proportional to the probability of a measurement of the
particle's wavenumber yielding a value in the range  to
to 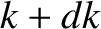 . (Recall that
. (Recall that
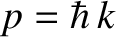 ,
so a measurement of the particle's wavenumber,
,
so a measurement of the particle's wavenumber,  , is equivalent to a measurement of the particle's
momentum,
, is equivalent to a measurement of the particle's
momentum, 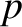 .) According to Equation (11.47),
.) According to Equation (11.47),
 -space. [See
Equation (11.42) and Figure 11.3.] Hence, a measurement of
-space. [See
Equation (11.42) and Figure 11.3.] Hence, a measurement of  is
most likely to yield the value
is
most likely to yield the value 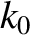 , and very unlikely to yield
a value that differs from
, and very unlikely to yield
a value that differs from 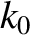 by more than
by more than
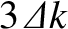 .
.
We have just seen that a wave packet with a Gaussian probability distribution of characteristic
width
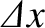 in
in  -space [see Equation (11.42)] is equivalent to a wave packet with a Gaussian probability distribution of characteristic width
-space [see Equation (11.42)] is equivalent to a wave packet with a Gaussian probability distribution of characteristic width
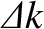 in
in  -space [see Equation (11.49)],
where
-space [see Equation (11.49)],
where
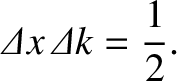 |
(11.50) |
 -space (i.e., with small
-space (i.e., with small
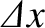 ) we need
to combine plane waves with a very wide range of different
) we need
to combine plane waves with a very wide range of different  -values
(i.e., with large
-values
(i.e., with large
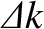 ). Conversely, if we only combine
plane waves whose wavenumbers differ by a small amount (i.e., if
). Conversely, if we only combine
plane waves whose wavenumbers differ by a small amount (i.e., if
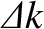 is small) then the resulting wave packet is highly
extended in
is small) then the resulting wave packet is highly
extended in  -space (i.e.,
-space (i.e.,
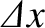 is large).
is large).
According to Section 9.2, a wave packet made up of a superposition of
plane waves that is strongly peaked around some
central wavenumber 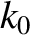 propagates at the group velocity,
propagates at the group velocity,
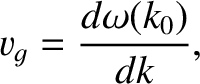 |
(11.51) |
 ,
assuming that all of the constituent plane waves satisfy a dispersion relation of the form
,
assuming that all of the constituent plane waves satisfy a dispersion relation of the form
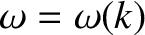 . For the case of
matter waves, the dispersion relation is (11.24). Thus, the associated group velocity is
. For the case of
matter waves, the dispersion relation is (11.24). Thus, the associated group velocity is
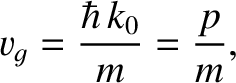 |
(11.52) |
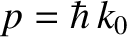 . This velocity is identical to the classical
velocity of a (non-relativistic) massive particle. We conclude that the matter-wave dispersion relation (11.24) is perfectly consistent
with classical physics, as long as we recognize that particles must be identified with
wave packets (which propagate at the group velocity) rather than plane waves (which propagate at the phase velocity).
. This velocity is identical to the classical
velocity of a (non-relativistic) massive particle. We conclude that the matter-wave dispersion relation (11.24) is perfectly consistent
with classical physics, as long as we recognize that particles must be identified with
wave packets (which propagate at the group velocity) rather than plane waves (which propagate at the phase velocity).
In Section 9.2, it was demonstrated that the spatial extent of a wave packet of initial extent
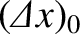 grows, as the packet evolves in time, like
grows, as the packet evolves in time, like
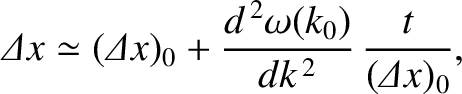 |
(11.53) |
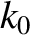 is the packet's central wavenumber. Thus, it follows from the matter-wave dispersion relation, (11.24), that the
width of a particle wave packet grows in time as
For example, if an electron wave packet is initially localized in a region of atomic dimensions (i.e.,
is the packet's central wavenumber. Thus, it follows from the matter-wave dispersion relation, (11.24), that the
width of a particle wave packet grows in time as
For example, if an electron wave packet is initially localized in a region of atomic dimensions (i.e.,
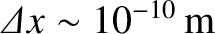 )
then the width of the packet doubles in about
)
then the width of the packet doubles in about
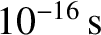 .
.