


Next: Example 8.2: Charged particle
Up: Magnetism
Previous: Worked Examples
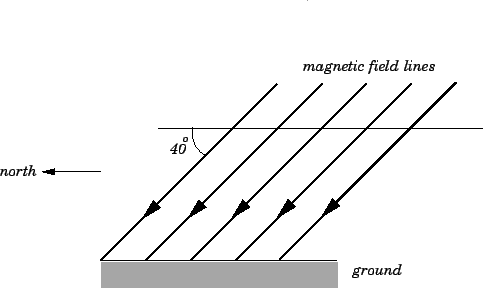
Question: In Texas, the
Earth's magnetic field is approximately uniform, and of magnitude
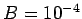 T. The horizontal component of the field is directed northward.
The field also has a vertical component which is directed into the ground. The
angle the field lines dip below the horizontal is
T. The horizontal component of the field is directed northward.
The field also has a vertical component which is directed into the ground. The
angle the field lines dip below the horizontal is 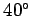 . A metal
bar of length
. A metal
bar of length 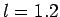 m carries a current of
m carries a current of 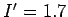 A. Suppose that the
bar is held horizontally such that the current flows from
East to West. What is the magnitude and direction of the magnetic
force on the bar? Suppose that the direction of the current is reversed.
What, now,
is the magnitude and direction of the magnetic
force on the bar? Suppose that the bar is held vertically such the current
flows upward. What
is the magnitude and direction of the magnetic
force on the bar? Suppose, finally, that the direction of the current is
reversed. What, now, is the magnitude and direction of the magnetic
force on the bar?
A. Suppose that the
bar is held horizontally such that the current flows from
East to West. What is the magnitude and direction of the magnetic
force on the bar? Suppose that the direction of the current is reversed.
What, now,
is the magnitude and direction of the magnetic
force on the bar? Suppose that the bar is held vertically such the current
flows upward. What
is the magnitude and direction of the magnetic
force on the bar? Suppose, finally, that the direction of the current is
reversed. What, now, is the magnitude and direction of the magnetic
force on the bar?
Answer: If the current in the bar flows horizontally
from East to West then the direction
of the current makes an angle of 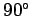 with the direction of the
magnetic field. So, from Eq. (152), the magnetic force per unit length
acting on the bar is
with the direction of the
magnetic field. So, from Eq. (152), the magnetic force per unit length
acting on the bar is
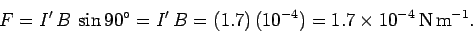
Thus, the total force acting on the bar
is
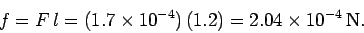
Using the right-hand rule, if the index finger of a right-hand points horizontally from
East to West, and the middle finger points northward, but dips 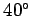 below
the horizontal, then the thumb points southward, but dips
below
the horizontal, then the thumb points southward, but dips 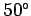 below
the horizontal. Thus, the force on the bar is directed southward, and
dips
below
the horizontal. Thus, the force on the bar is directed southward, and
dips 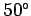 below
the horizontal.
If the current in the bar is reversed, so that it now flows horizontally from West to
East, then the angle subtended between the direction of current flow and
the direction of the magnetic field is still
below
the horizontal.
If the current in the bar is reversed, so that it now flows horizontally from West to
East, then the angle subtended between the direction of current flow and
the direction of the magnetic field is still 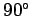 , so the magnitude
of the force on the bar remains unchanged. According to the right-hand rule,
if the index finger of a right-hand points horizontally from
West to East, and the middle finger points northward, but dips
, so the magnitude
of the force on the bar remains unchanged. According to the right-hand rule,
if the index finger of a right-hand points horizontally from
West to East, and the middle finger points northward, but dips 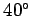 below
the horizontal, then the thumb points northward, but is directed
below
the horizontal, then the thumb points northward, but is directed 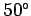 above
the horizontal. Thus, the force on the bar is directed northward at an angle of
above
the horizontal. Thus, the force on the bar is directed northward at an angle of
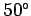 above
the horizontal. In other words, the new force points in exactly the opposite
direction to the old one.
above
the horizontal. In other words, the new force points in exactly the opposite
direction to the old one.
If the current in the bar flows vertically upward then the direction of
current flow subtends an angle of 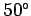 degrees with the direction
of the magnetic field. So, from Eq. (152), the magnetic force per unit length
acting on the bar is
degrees with the direction
of the magnetic field. So, from Eq. (152), the magnetic force per unit length
acting on the bar is
Thus, the total force acting on the bar
is
Using the right-hand rule, if the index finger of a right-hand points vertically
upward, and the middle finger points northward, but dips 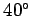 below
the horizontal, then the thumb points horizontally westward. Thus, the force on the bar is directed horizontally westward. If the current in the bar is reversed, so that
it flows vertically downward, then the force on the bar is of the same magnitude, but points in the opposite direction, which means that the new force
points horizontally eastward.
below
the horizontal, then the thumb points horizontally westward. Thus, the force on the bar is directed horizontally westward. If the current in the bar is reversed, so that
it flows vertically downward, then the force on the bar is of the same magnitude, but points in the opposite direction, which means that the new force
points horizontally eastward.



Next: Example 8.2: Charged particle
Up: Magnetism
Previous: Worked Examples
Richard Fitzpatrick
2007-07-14


![]() degrees with the direction
of the magnetic field. So, from Eq. (152), the magnetic force per unit length
acting on the bar is
degrees with the direction
of the magnetic field. So, from Eq. (152), the magnetic force per unit length
acting on the bar is