Worked example 10.4: Truck crossing a bridge

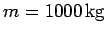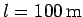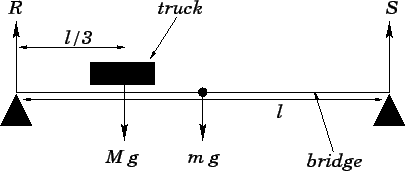
Question: A truck of mass $M=5000 {\rm kg}$ is crossing a uniform horizontal bridge of mass $m=1000 {\rm kg}$ and length $l=100 {\rm m}$. The bridge is supported at its two end-points. What are the reactions at these supports when the truck is one third of the way across the bridge?

Answer: Let $R$ and $S$ be the reactions at the bridge supports. Here, $R$ is the reaction at the support closest to the truck. Setting the net vertical force acting on the bridge to zero, we obtain

$$R+S-M g - m g = 0.$$

Setting the torque acting on the bridge about the left-most support to zero, we get

$$M g l/3 + m g l/2 - S l = 0.$$

Here, we have made use of the fact that centre of mass of the bridge lies at its mid-point. It follows from the above two equations that

$$S = M g/3 + m g/2 = 5000\times 9.81/3 + 1000\times 9.81 /2 = 2.13\times 10^4 {\rm N},$$

and

$$R = M g + m g - S = (5000+1000)\times 9.81- 2.13\times 10^4 = 3.76\times 10^4 {\rm N}.$$