

Next: Mercury
Up: The Inferior Planets
Previous: Venus
The geocentric orbit of an inferior planet is similar to that of the
superior planet shown in Fig. 32, except for the fact that the sun
is coincident with guide-point 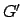 in the former case.
It follows that it is impossible
for an inferior planet to have an opposition with the sun (i.e, for the
earth to lie directly between the planet and the sun). However, inferior planets
do have two different kinds of conjunctions with the sun. A superior
conjuction takes place when the sun lies directly between the planet and the earth.
Conversely, an inferior conjunction takes place when the planet lies
directly between the sun and the earth. It is clear from Fig. 32
that a superior conjunction corresponds to
in the former case.
It follows that it is impossible
for an inferior planet to have an opposition with the sun (i.e, for the
earth to lie directly between the planet and the sun). However, inferior planets
do have two different kinds of conjunctions with the sun. A superior
conjuction takes place when the sun lies directly between the planet and the earth.
Conversely, an inferior conjunction takes place when the planet lies
directly between the sun and the earth. It is clear from Fig. 32
that a superior conjunction corresponds to 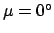 , and
an inferior conjunction to
, and
an inferior conjunction to 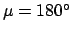 . Now, the equation of the epicycle,
. Now, the equation of the epicycle,
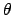 , measures the
angular separation between the planet and the sun (since the sun lies at
the guide-point). It is evident from Figure 32 that
, measures the
angular separation between the planet and the sun (since the sun lies at
the guide-point). It is evident from Figure 32 that 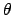 attains
a maximum and a minimum value each time the planet revolves
around its epicycle.
In other words, there is a limit to how large the angular separation between
an inferior planet and the sun can become.
The maximum value is termed the greatest eastern elongation
of the planet, whereas the modulus of the minimum value is
termed the greatest western elongation.
attains
a maximum and a minimum value each time the planet revolves
around its epicycle.
In other words, there is a limit to how large the angular separation between
an inferior planet and the sun can become.
The maximum value is termed the greatest eastern elongation
of the planet, whereas the modulus of the minimum value is
termed the greatest western elongation.
Tables 58-60 can be used to determine the dates of the conjunctions and greatest elongations
of Venus. Consider the first superior conjunction after the epoch (January 1, 2000 CE). We can estimate the
time at which this event occurs by approximating the epicyclic anomaly as the
mean epicyclic anomaly:
Thus,
A calculation of the epicyclic anomaly at this time, using Tables 58-60, yields
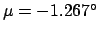 . Now, the actual conjunction
takes place when
. Now, the actual conjunction
takes place when 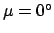 .
Hence, our final estimate is
.
Hence, our final estimate is
which corresponds to June 11, 2000 CE.
Consider the first inferior conjunction of Venus after the epoch. Our first estimate of the time at which this
event takes place is
A calculation of
the epicyclic anomaly at this time yields
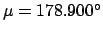 . Now, the
actual conjunction takes place when
. Now, the
actual conjunction takes place when 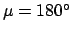 .
Hence, our final estimate is
.
Hence, our final estimate is
which corresponds to
March 30, 2001 CE. Incidentally, it is clear from the above analysis that the
mean time period between successive superior, or inferior, conjunctions of Venus is
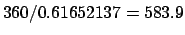 JD, which is
equivalent to
JD, which is
equivalent to 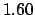 years.
years.
Consider the greatest elongations of Venus. We can approximate the
equation of the epicycle as
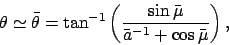 |
(202) |
where 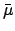 is the mean epicyclic anomaly, and
is the mean epicyclic anomaly, and
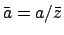 .
It follows that
.
It follows that
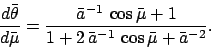 |
(203) |
Now, 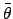 attains its maximum or minimum value when
attains its maximum or minimum value when
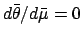 : i.e., when
: i.e., when
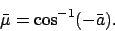 |
(204) |
For the case of Venus, we obtain
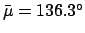 or
or 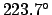 .
The first solution corresponds to the greatest eastern elongation, and the
second to the greatest western elongation. Substituting back into Eq. (202), we find that
.
The first solution corresponds to the greatest eastern elongation, and the
second to the greatest western elongation. Substituting back into Eq. (202), we find that
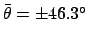 .
Hence, the mean value of the greatest eastern or western
elongation of Venus is
.
Hence, the mean value of the greatest eastern or western
elongation of Venus is 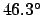 . The mean time period
between a greatest eastern elongation and the following
inferior conjunction, or between an inferior conjunction and the
following greatest western elongation, is
. The mean time period
between a greatest eastern elongation and the following
inferior conjunction, or between an inferior conjunction and the
following greatest western elongation, is
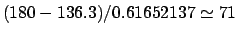 JD. Unfortunately, the only option for accurately determining the dates at which the greatest elongations occur is to calculate
the equation of the epicycle of Venus over a range of days centered 71 days before and after an inferior conjunction.
JD. Unfortunately, the only option for accurately determining the dates at which the greatest elongations occur is to calculate
the equation of the epicycle of Venus over a range of days centered 71 days before and after an inferior conjunction.
Table 61 shows the conjunctions, and greatest elongations
of Venus for the years 2000-2015 CE, calculated using the
techniques described above.


Next: Mercury
Up: The Inferior Planets
Previous: Venus
Richard Fitzpatrick
2010-07-21