


Next: The isothermal atmosphere
Up: Classical thermodynamics
Previous: Isothermal and adiabatic expansion
The gas which we are most familiar with in everyday life is, of course, the Earth's
atmosphere. In fact, we can use the isothermal
and adiabatic gas laws to
explain most of the observable
features of the atmosphere.
Let us, first of all, consider the hydrostatic equilibrium of the atmosphere.
Consider a thin vertical slice
of the atmosphere of cross-sectional area  which starts at height
which starts at height 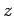 above
ground level and extends to
height
above
ground level and extends to
height 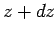 . The upwards force exerted on this slice from the gas below
is
. The upwards force exerted on this slice from the gas below
is 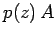 , where
, where 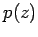 is the pressure at height
is the pressure at height 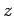 .
Likewise, the downward force exerted by the gas above the slice is
.
Likewise, the downward force exerted by the gas above the slice is
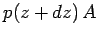 . The net upward force is clearly
. The net upward force is clearly
![$[p(z) - p(z+dz)]A$](img805.png) . In equilibrium,
this upward force must be balanced by the downward force due to the weight of
the slice: this is
. In equilibrium,
this upward force must be balanced by the downward force due to the weight of
the slice: this is
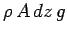 , where
, where 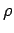 is the density of
the gas, and
is the density of
the gas, and  is the acceleration due to gravity. In follows that the
force balance condition can be written
is the acceleration due to gravity. In follows that the
force balance condition can be written
![\begin{displaymath}[p(z)- p(z+dz)]A = \rho \,A\,dz\,g,
\end{displaymath}](img807.png) |
(322) |
which reduces to
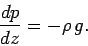 |
(323) |
This is called the equation of hydrostatic equilibrium for the atmosphere.
We can write the density of a gas in the following form,
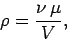 |
(324) |
where 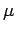 is the molecular weight of the gas,
and is equal to the mass of one mole of gas particles.
For instance, the molecular weight of Nitrogen gas is 28 grams.
The above formula for the density of a gas
combined with the ideal gas law
is the molecular weight of the gas,
and is equal to the mass of one mole of gas particles.
For instance, the molecular weight of Nitrogen gas is 28 grams.
The above formula for the density of a gas
combined with the ideal gas law
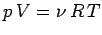 yields
yields
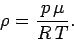 |
(325) |
It follows that the equation of hydrostatic equilibrium can be rewritten
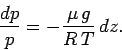 |
(326) |



Next: The isothermal atmosphere
Up: Classical thermodynamics
Previous: Isothermal and adiabatic expansion
Richard Fitzpatrick
2006-02-02
![]() which starts at height
which starts at height ![]() above
ground level and extends to
height
above
ground level and extends to
height ![]() . The upwards force exerted on this slice from the gas below
is
. The upwards force exerted on this slice from the gas below
is ![]() , where
, where ![]() is the pressure at height
is the pressure at height ![]() .
Likewise, the downward force exerted by the gas above the slice is
.
Likewise, the downward force exerted by the gas above the slice is
![]() . The net upward force is clearly
. The net upward force is clearly
![]() . In equilibrium,
this upward force must be balanced by the downward force due to the weight of
the slice: this is
. In equilibrium,
this upward force must be balanced by the downward force due to the weight of
the slice: this is
![]() , where
, where ![]() is the density of
the gas, and
is the density of
the gas, and ![]() is the acceleration due to gravity. In follows that the
force balance condition can be written
is the acceleration due to gravity. In follows that the
force balance condition can be written