 |
 |
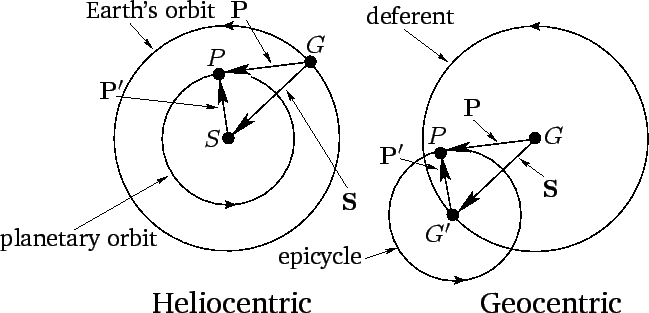
As we have seen, the deferent of a superior planet has the same elements as the planet's orbit about the sun, whereas the epicycle has the same elements as the sun's apparent orbit about the earth. On the other hand, the deferent of an inferior planet has the same elements as the sun's apparent orbit about the earth, whereas the epicycle has the same elements as the planet's orbit about the sun. It follows that we can formulate a procedure for determining the ecliptic longitude of an inferior planet by simply taking the procedure used in the previous section for determining the ecliptic longitude of a superior planet and exchanging the roles of the sun and the planet.
Our procedure is described below. As before, it is assumed that the ecliptic longitude, ![]() , and the
radial anomaly,
, and the
radial anomaly, ![]() , of the sun have already been calculated.
In the following,
, of the sun have already been calculated.
In the following, ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
,
![]() , and
, and ![]() represent elements of the orbit of the planet in question
about the sun, whereas
represent elements of the orbit of the planet in question
about the sun, whereas ![]() is the eccentricity
of the sun's apparent orbit about the earth.
Again,
is the eccentricity
of the sun's apparent orbit about the earth.
Again, ![]() is the major radius of the planetary orbit in
units in which the major radius of the sun's apparent orbit about the
earth is unity. The requisite elements for all of the inferior planets at the J2000 epoch (
is the major radius of the planetary orbit in
units in which the major radius of the sun's apparent orbit about the
earth is unity. The requisite elements for all of the inferior planets at the J2000 epoch (
![]() JD)
are listed in Table 30. The ecliptic longitude of an inferior planet
is specified by the following formulae:
JD)
are listed in Table 30. The ecliptic longitude of an inferior planet
is specified by the following formulae:
For the case of Venus, the above formulae are capable of matching NASA ephemeris data during the years 1995-2006 CE
with a mean error of ![]() and a maximum error of
and a maximum error of ![]() . For the case of Mercury, given its relatively large eccentricity of
. For the case of Mercury, given its relatively large eccentricity of
![]() , it is necessary to modify the formulae slightly by expressing
, it is necessary to modify the formulae slightly by expressing ![]() and
and ![]() to
third-order in the eccentricity:
to
third-order in the eccentricity:
| (200) | |||
| (201) |