For the case of Maxwell-Boltzmann statistics, the two particles are
considered to be distinguishable. Let us denote them ![]() and
and ![]() .
Furthermore, any number of particles can occupy the same quantum state.
The possible different states of the gas are shown in Table 8.1.
There are clearly nine distinct states.
.
Furthermore, any number of particles can occupy the same quantum state.
The possible different states of the gas are shown in Table 8.1.
There are clearly nine distinct states.
For the case of Bose-Einstein statistics, the two particles are
considered to be indistinguishable. Let us denote them both as ![]() .
Furthermore, any number of particles can occupy the same quantum state.
The possible different states of the gas are shown in Table 8.2.
There are clearly six distinct states.
.
Furthermore, any number of particles can occupy the same quantum state.
The possible different states of the gas are shown in Table 8.2.
There are clearly six distinct states.
Finally, for the case of Fermi-Dirac statistics, the two particles are
considered to be indistinguishable. Let us again denote them both as ![]() .
Furthermore, no more than one particle can occupy a given quantum state. The possible different states of the gas are shown in Table 8.3.
There are clearly only three distinct states.
.
Furthermore, no more than one particle can occupy a given quantum state. The possible different states of the gas are shown in Table 8.3.
There are clearly only three distinct states.
It follows, from the previous example, that Fermi-Dirac (FD) statistics are more restrictive (i.e., there are less possible states of the system) than Bose-Einstein (BE) statistics, which are, in turn, more restrictive than Maxwell-Boltzmann (MB) statistics. Let
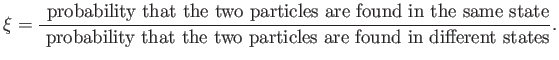 |
(8.12) |
| (8.13) | ||
| (8.14) | ||
| (8.15) |