


Next: Ideal Gas Equation of
Up: Classical Thermodynamics
Previous: Classical Thermodynamics
We have already learned that macroscopic quantities, such as energy, temperature, and pressure,
are, in fact, statistical in nature. In other words, in an equilibrium state, they exhibit random
fluctuations about some mean value. If we were to plot the probability
distribution for the energy (say) of a system in thermal equilibrium with its
surroundings then we would obtain a Gaussian distribution with a very small fractional width.
In fact, we expect
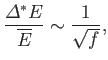 |
(6.1) |
where the number of degrees of freedom, 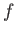 , is about
, is about 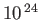 for laboratory-scale systems.
This implies that the statistical fluctuations of macroscopic
quantities about their mean values are typically only about 1 part in
for laboratory-scale systems.
This implies that the statistical fluctuations of macroscopic
quantities about their mean values are typically only about 1 part in 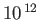 .
.
Because the statistical fluctuations of equilibrium quantities are so small, it is an excellent approximation to
neglect them altogether, and, thereby, to replace macroscopic
quantities, such as energy, temperature, and pressure, by their mean
values. In other words,
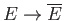 ,
,
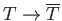 ,
,
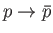 , et cetera.
In the following discussion, we shall drop the overbars, so that
, et cetera.
In the following discussion, we shall drop the overbars, so that  should
be understood to represent the mean energy,
should
be understood to represent the mean energy,
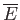 , et cetera. This
prescription, which is the essence of classical thermodynamics, is equivalent to
replacing all statistically-varying quantities by their most probable values.
, et cetera. This
prescription, which is the essence of classical thermodynamics, is equivalent to
replacing all statistically-varying quantities by their most probable values.
Although, formally, there are four laws of thermodynamics (i.e., the zeroth to the
third), the zeroth law is really a consequence of the second law, and the third
law is only important at temperatures close to absolute zero. So, for
most practical purposes, the two laws that actually matter are the first law and the second law.
For an infinitesimal process, the first law of thermodynamics is written
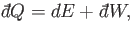 |
(6.2) |
where 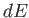 is the change in internal energy of the system,
is the change in internal energy of the system,
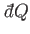 the heat
absorbed by the system from its surroundings, and
the heat
absorbed by the system from its surroundings, and
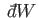 the work done
by the system on its surroundings. Note that this particular formulation of the first law is
merely a convention. We could equally well write the first law in terms of the heat
emitted by the system, or the work done on the system. It does not
really matter, as
long as we are consistent in our definitions.
The second law of thermodynamics implies that
the work done
by the system on its surroundings. Note that this particular formulation of the first law is
merely a convention. We could equally well write the first law in terms of the heat
emitted by the system, or the work done on the system. It does not
really matter, as
long as we are consistent in our definitions.
The second law of thermodynamics implies that
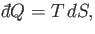 |
(6.3) |
for an infinitesimal quasi-static process,
where  is the thermodynamic temperature, and
is the thermodynamic temperature, and 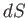 the change in entropy of
the system. Furthermore, for systems in which the only external parameter is the
volume (e.g., gases),
the work done on the environment is
the change in entropy of
the system. Furthermore, for systems in which the only external parameter is the
volume (e.g., gases),
the work done on the environment is
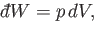 |
(6.4) |
where  is the pressure, and
is the pressure, and 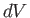 the change in volume.
Thus, the first
and second laws of thermodynamics can be combined to give the fundamental thermodynamic relation
the change in volume.
Thus, the first
and second laws of thermodynamics can be combined to give the fundamental thermodynamic relation
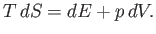 |
(6.5) |



Next: Ideal Gas Equation of
Up: Classical Thermodynamics
Previous: Classical Thermodynamics
Richard Fitzpatrick
2016-01-25
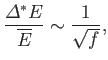
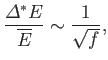
![]() ,
,
![]() ,
,
![]() , et cetera.
In the following discussion, we shall drop the overbars, so that
, et cetera.
In the following discussion, we shall drop the overbars, so that ![]() should
be understood to represent the mean energy,
should
be understood to represent the mean energy,
![]() , et cetera. This
prescription, which is the essence of classical thermodynamics, is equivalent to
replacing all statistically-varying quantities by their most probable values.
, et cetera. This
prescription, which is the essence of classical thermodynamics, is equivalent to
replacing all statistically-varying quantities by their most probable values.