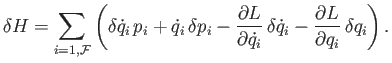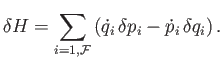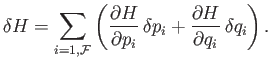Next: Wave Mechanics
Up: Classical Mechanics
Previous: Hamilton's Principle
Hamilton's Equations
Consider a dynamical system with  degrees of freedom that is described
by the generalized coordinates
degrees of freedom that is described
by the generalized coordinates 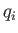 , for
, for
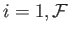 . Suppose that
neither the kinetic energy,
. Suppose that
neither the kinetic energy, 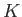 , nor the potential energy,
, nor the potential energy, 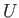 , depend
explicitly on the time,
, depend
explicitly on the time,  . In conventional dynamical systems, the potential energy is generally independent of the
. In conventional dynamical systems, the potential energy is generally independent of the 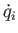 , whereas the kinetic
energy takes the form of a homogeneous quadratic function of
the
, whereas the kinetic
energy takes the form of a homogeneous quadratic function of
the 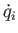 . In other words,
. In other words,
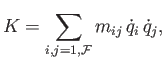 |
(B.68) |
where the 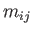 depend on the
depend on the 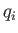 , but not on the
, but not on the 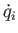 .
It is easily demonstrated from the previous equation that
.
It is easily demonstrated from the previous equation that
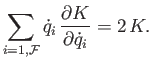 |
(B.69) |
Recall, from Section B.4, that generalized momentum conjugate to the 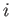 th
generalized coordinate is defined
th
generalized coordinate is defined
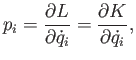 |
(B.70) |
where 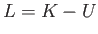 is the Lagrangian of the system, and we have made use of the fact that
is the Lagrangian of the system, and we have made use of the fact that 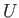 is independent of the
is independent of the 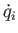 . Consider the
function
. Consider the
function
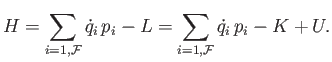 |
(B.71) |
If all of the conditions discussed previously are satisfied then Equations (B.69)
and (B.70)
yield
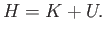 |
(B.72) |
In other words, the function 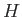 is equal to the total energy of the system.
is equal to the total energy of the system.
Consider the variation of the function 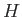 . We have
. We have
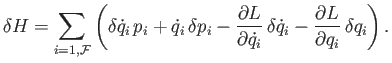 |
(B.73) |
The first and third terms in the bracket cancel, because
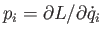 . Furthermore, because Lagrange's equation
can be written
. Furthermore, because Lagrange's equation
can be written
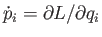 (see Section B.4), we obtain
(see Section B.4), we obtain
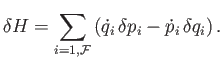 |
(B.74) |
Suppose, now, that we can express the total energy of the system, 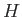 , solely
as a function of the
, solely
as a function of the 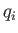 and the
and the 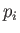 , with no explicit
dependence on the
, with no explicit
dependence on the 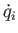 . In other words, suppose that we
can write
. In other words, suppose that we
can write
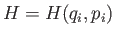 . When the energy is written
in this fashion it is generally termed the Hamiltonian of the system. The variation of the Hamiltonian function takes the form
. When the energy is written
in this fashion it is generally termed the Hamiltonian of the system. The variation of the Hamiltonian function takes the form
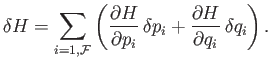 |
(B.75) |
A comparison of the previous two equations yields
for
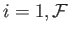 . These
. These 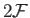 first-order differential equations are known
as Hamilton's equations. Hamilton's equations are often a
useful alternative to Lagrange's equations, which take the
form of
first-order differential equations are known
as Hamilton's equations. Hamilton's equations are often a
useful alternative to Lagrange's equations, which take the
form of  second-order differential equations.
second-order differential equations.



Next: Wave Mechanics
Up: Classical Mechanics
Previous: Hamilton's Principle
Richard Fitzpatrick
2016-01-25
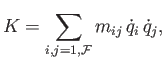
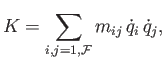
![]() th
generalized coordinate is defined
th
generalized coordinate is defined
![]() . We have
. We have