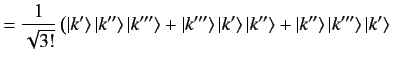Next: Symmetrization Postulate
Up: Identical Particles
Previous: Identical Particles
Consider a system consisting of a collection of identical particles.
In classical mechanics, it is, in principle, possible to continuously monitor the position of each particle as a function of time.
Hence, the constituent particles can be unambiguously labeled. In quantum mechanics, on the other hand, this is not possible because continuous position
measurements would disturb the system. It follows that identical particles cannot be unambiguously labeled in quantum
mechanics.
Consider a quantum system consisting of two identical particles. Suppose that one of the particles--particle 1, say--is characterized by the state ket
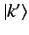 . Here,
. Here, 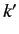 represents the eigenvalues of the complete set of commuting observables associated with the particle. Suppose that the other particle--particle 2--is
characterized by the state ket
represents the eigenvalues of the complete set of commuting observables associated with the particle. Suppose that the other particle--particle 2--is
characterized by the state ket
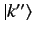 . The state ket for the whole system can be written in the product form
. The state ket for the whole system can be written in the product form
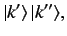 |
(1028) |
where it is understood that the first ket corresponds to particle 1, and the second to particle 2. We can also
consider the ket
 |
(1029) |
which corresponds to a state in which particle 1 has the eigenvalues 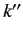 , and particle
, and particle 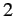 the eigenvalues
the eigenvalues 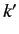 .
.
Suppose that we were to measure all of the simultaneously measurable properties of our two-particle system. We might obtain the results 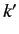 for one particle, and
for one particle, and 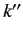 for the other.
However, we have no way of knowing whether the corresponding state ket is
for the other.
However, we have no way of knowing whether the corresponding state ket is
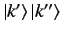 or
or
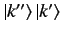 , or any
linear combination of these two kets. In other words, all state kets of the form
, or any
linear combination of these two kets. In other words, all state kets of the form
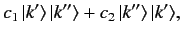 |
(1030) |
correspond to an identical set of results when the properties of the system are measured. This phenomenon is
known as exchange degeneracy. Such degeneracy is problematic because the specification of a complete set of observable eigenvalues in a system of identical particles does not seem to uniquely determine the corresponding state ket. Fortunately, nature has a way of avoiding this difficulty.
Consider the permutation operator 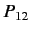 , which is defined such that
, which is defined such that
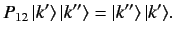 |
(1031) |
In other words, 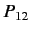 swaps the identities of particles
swaps the identities of particles 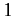 and
and 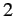 . It is
easily seen that
. It is
easily seen that
Now, the Hamiltonian of a system of two identical particles must necessarily be a symmetric function of each particle's observables (because
exchange of identical particles could not possibly affect the overall energy of the system). For instance,
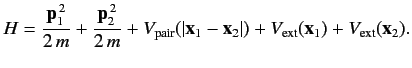 |
(1034) |
Here, we have separated the mutual interaction of the two particles from their interaction with an external potential. It follows that if
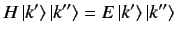 |
(1035) |
then
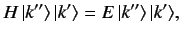 |
(1036) |
where 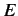 is the total energy.
Operating on both sides of (1035) with
is the total energy.
Operating on both sides of (1035) with 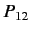 , and making use of Equation (1033), we obtain
, and making use of Equation (1033), we obtain
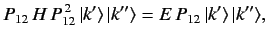 |
(1037) |
or
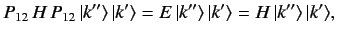 |
(1038) |
where use has been made of (1036).
We deduce that
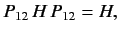 |
(1039) |
which implies [from (1033)] that
![$\displaystyle [H,P_{12}] = 0.$](img2391.png) |
(1040) |
In other words, an eigenstate of the Hamiltonian is a simultaneous eigenstate of the permutation operator 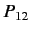 .
.
Now, according to Equation (1033), the permutation operator possesses the eigenvalues 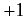 and
and  , respectively. The corresponding properly normalized eigenstates are
, respectively. The corresponding properly normalized eigenstates are
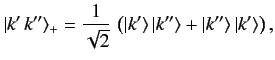 |
(1041) |
and
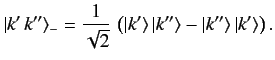 |
(1042) |
Here, it is assumed that
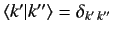 . Note that
. Note that
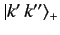 is symmetric with respect to interchange of particles--i.e.,
is symmetric with respect to interchange of particles--i.e.,
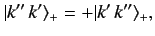 |
(1043) |
whereas
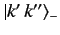 is antisymmetric--i.e.,
is antisymmetric--i.e.,
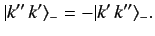 |
(1044) |
Let us now consider a system of three identical particles. We can represent the overall state ket as
 |
(1045) |
where 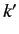 ,
, 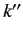 , and
, and 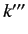 are the eigenvalues of particles 1, 2, and 3, respectively. We can also
define the two-particle permutation operators
are the eigenvalues of particles 1, 2, and 3, respectively. We can also
define the two-particle permutation operators
It is easily demonstrated that
and
As before, the Hamiltonian of the system must be a symmetric function of the particle's observables: i.e.,
where 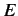 is the total energy.
Using analogous arguments to those employed for the two-particle system,
we deduce that
is the total energy.
Using analogous arguments to those employed for the two-particle system,
we deduce that
![$\displaystyle [H,P_{12}] = [H,P_{23}] = [H,P_{31}] = 0.$](img2427.png) |
(1061) |
Hence, an eigenstate of the Hamiltonian is a simultaneous eigenstate of the permutation operators 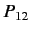 ,
, 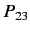 , and
, and 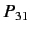 .
However, according to Equations (1052)-(1054), the possible eigenvalues of these operators are
.
However, according to Equations (1052)-(1054), the possible eigenvalues of these operators are 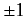 .
.
Let us define the cyclic permutation operator 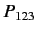 , where
, where
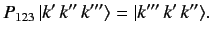 |
(1062) |
It follows that
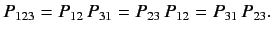 |
(1063) |
It is also clear from Equations (1055) and (1060) that
![$\displaystyle [H,P_{123}] = 0.$](img2433.png) |
(1064) |
Thus, an eigenstate of the Hamiltonian is a simultaneous eigenstate of the permutation operators 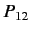 ,
, 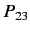 ,
, 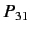 ,
and
,
and 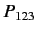 . Let
. Let
 ,
,
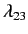 ,
,
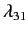 and
and
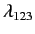 represent the eigenvalues of these operators,
respectively. We know that
represent the eigenvalues of these operators,
respectively. We know that
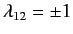 ,
,
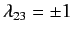 , and
, and
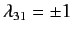 . Moreover, it follows from
(1063) that
. Moreover, it follows from
(1063) that
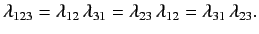 |
(1065) |
The above equations imply that
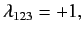 |
(1066) |
and
either
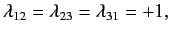 |
(1067) |
or
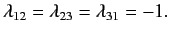 |
(1068) |
In other words, the multi-particle state ket must be either totally symmetric, or totally antisymmetric, with respect to swapping the
identities of any given pair
of particles.
Thus, in terms of properly normalized single particle kets, the properly normalized totally symmetric and totally antisymmetric kets are
and
respectively.
The above arguments can be generalized to systems of more than three identical particles in a straightforward manner.



Next: Symmetrization Postulate
Up: Identical Particles
Previous: Identical Particles
Richard Fitzpatrick
2013-04-08
![]() . Here,
. Here, ![]() represents the eigenvalues of the complete set of commuting observables associated with the particle. Suppose that the other particle--particle 2--is
characterized by the state ket
represents the eigenvalues of the complete set of commuting observables associated with the particle. Suppose that the other particle--particle 2--is
characterized by the state ket
![]() . The state ket for the whole system can be written in the product form
. The state ket for the whole system can be written in the product form
![]() for one particle, and
for one particle, and ![]() for the other.
However, we have no way of knowing whether the corresponding state ket is
for the other.
However, we have no way of knowing whether the corresponding state ket is
![]() or
or
![]() , or any
linear combination of these two kets. In other words, all state kets of the form
, or any
linear combination of these two kets. In other words, all state kets of the form
![]() , which is defined such that
, which is defined such that
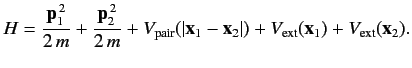
![]() and
and ![]() , respectively. The corresponding properly normalized eigenstates are
, respectively. The corresponding properly normalized eigenstates are
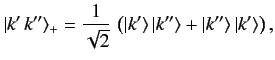
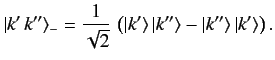
![]() , where
, where