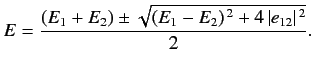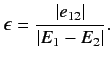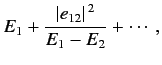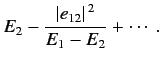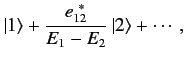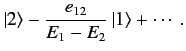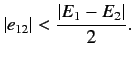Next: Non-Degenerate Perturbation Theory
Up: Time-Independent Perturbation Theory
Previous: Introduction
Let us start by considering time-independent perturbation theory,
in which the modification to the Hamiltonian, 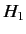 , has no explicit
dependence on time. It is usually assumed that the unperturbed
Hamiltonian,
, has no explicit
dependence on time. It is usually assumed that the unperturbed
Hamiltonian, 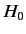 , is also time-independent.
, is also time-independent.
Consider the simplest non-trivial system, in which there are only two
independent eigenkets of the unperturbed Hamiltonian. These are denoted
It is assumed that these states, and their associated eigenvalues, are known.
Because 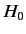 is, by definition, an Hermitian operator,
its two eigenkets are mutually orthogonal
and form a complete set. The lengths of these
eigenkets are both normalized to unity.
Let us now try to solve the modified energy eigenvalue problem
is, by definition, an Hermitian operator,
its two eigenkets are mutually orthogonal
and form a complete set. The lengths of these
eigenkets are both normalized to unity.
Let us now try to solve the modified energy eigenvalue problem
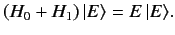 |
(586) |
In fact, we can solve this problem exactly. Since the eigenkets of 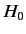 form a
complete set, we can write
form a
complete set, we can write
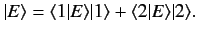 |
(587) |
Right-multiplication of Equation (586) by
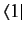 and
and
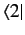 yields two
coupled equations, which can be written in matrix form:
yields two
coupled equations, which can be written in matrix form:
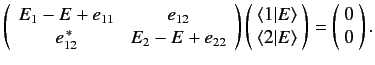 |
(588) |
Here,
In the special (but common) case of a perturbing Hamiltonian whose diagonal
matrix elements (in the unperturbed eigenstates) are zero, so that
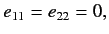 |
(592) |
the solution of Equation (588) (obtained by setting the determinant of the matrix
equal to zero) is
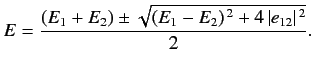 |
(593) |
Let us expand in the supposedly small parameter
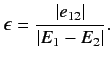 |
(594) |
We obtain
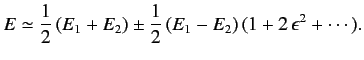 |
(595) |
The above expression yields the modifications to the energy eigenvalues due to
the perturbing Hamiltonian:
Note that 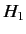 causes the upper eigenvalue to rise, and the lower
eigenvalue to fall. It is easily demonstrated that the modified eigenkets
take the form
causes the upper eigenvalue to rise, and the lower
eigenvalue to fall. It is easily demonstrated that the modified eigenkets
take the form
Thus, the modified energy eigenstates consist of one of the unperturbed eigenstates
with a slight admixture of the other. Note that the series expansion in Equation (595)
only converges if
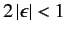 . This suggests that the condition for the
validity of the perturbation expansion is
. This suggests that the condition for the
validity of the perturbation expansion is
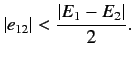 |
(600) |
In other words, when we say that 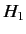 needs to be small compared to
needs to be small compared to 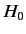 ,
what we really mean is that the above inequality needs to be satisfied.
,
what we really mean is that the above inequality needs to be satisfied.



Next: Non-Degenerate Perturbation Theory
Up: Time-Independent Perturbation Theory
Previous: Introduction
Richard Fitzpatrick
2013-04-08