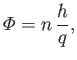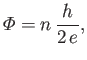Next: Exercises
Up: Quantum Dynamics
Previous: Gauge Transformations in Electromagnetism
Consider a situation in which the electric and magnetic fields are non-time-varying. In this case,
Equation (3.89) becomes
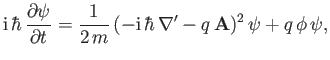 |
(3.103) |
where
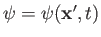 ,
,
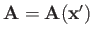 , and
, and
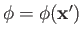 . The
previous equation has the formal solution
. The
previous equation has the formal solution
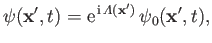 |
(3.104) |
where
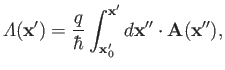 |
(3.105) |
and
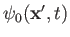 is a solution of
is a solution of
 |
(3.106) |
Here,
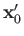 is an arbitrary fixed point. However, the solution (3.104) only makes sense in a
region in which
is an arbitrary fixed point. However, the solution (3.104) only makes sense in a
region in which
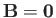 . To see this, let us imagine calculating the phase factor
. To see this, let us imagine calculating the phase factor
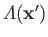 by evaluating the line integral specified in Equation (3.105) along two different paths, labelled 1 and 2, that join the
points
by evaluating the line integral specified in Equation (3.105) along two different paths, labelled 1 and 2, that join the
points
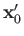 and
and 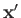 . We find that
. We find that
where
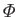 is the magnetic flux passing through a surface spanning the two paths. Here, we have
made use of the curl theorem [92], as well as Equation (3.73). Thus, the
phase factor
is the magnetic flux passing through a surface spanning the two paths. Here, we have
made use of the curl theorem [92], as well as Equation (3.73). Thus, the
phase factor
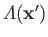 is only independent of the choice of path in the line integral when
is only independent of the choice of path in the line integral when
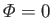 . Such independence is required if we insist that the wavefunction
be single valued.
. Such independence is required if we insist that the wavefunction
be single valued.
Suppose that a charged particle moves in a magnetic-field-free region that is not simply connected, but surrounds a
hole through which the magnetic flux
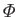 passes. Upon completing a circuit around the hole, the
particle's wavefunction is multiplied by
passes. Upon completing a circuit around the hole, the
particle's wavefunction is multiplied by
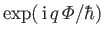 . The requirement that the wavefunction be
single-valued, and, hence, that the multiplication factor be unity, implies that the enclosed magnetic flux is quantized.
In fact.
. The requirement that the wavefunction be
single-valued, and, hence, that the multiplication factor be unity, implies that the enclosed magnetic flux is quantized.
In fact.
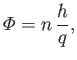 |
(3.108) |
where  is an integer. This effect is known as flux quantization.
is an integer. This effect is known as flux quantization.
A situation like that just described arises in the motion of electrons in a superconducting ring through which a magnetic field passes. Incidentally, superconductors contain no internal magnetic fields because they expel magnetic flux--this
behavior is called the Meissner effect [72]. Experimentally, the magnetic flux passing through a
superconducting ring is found to satisfy
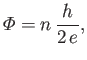 |
(3.109) |
where  is an integer, and
is an integer, and 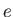 the magnitude of the electron charge [27,33]. This result
is consistent with our present understanding of the phenomenon of superconductivity, according to which
the fundamental charge carriers are correlated electron pairs known as Cooper pairs [6].
the magnitude of the electron charge [27,33]. This result
is consistent with our present understanding of the phenomenon of superconductivity, according to which
the fundamental charge carriers are correlated electron pairs known as Cooper pairs [6].
Consider an electron interference experiment in which a small solenoid containing magnetic flux is placed directly behind the
slits of a two-slit interference apparatus. The interference pattern at the screen is due
to the superposition of two parts of the wavefunction,
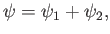 |
(3.110) |
where 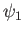 and
and 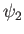 are the wavefunctions due to electrons that originate from the source, pass through the first and
second slits, respectively, and then strike the same point on the screen. When the solenoid is energized an additional phase shift
are the wavefunctions due to electrons that originate from the source, pass through the first and
second slits, respectively, and then strike the same point on the screen. When the solenoid is energized an additional phase shift
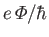 is introduced between
is introduced between 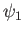 and
and 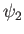 , where
, where
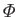 is the net flux passing through the solenoid. In other
words, the magnetic field internal to the solenoid affects the interference pattern seen on the screen, despite the
fact that neither electron beam ever directly experiences a magnetic field (because the field is internal to the
solenoid, and the two interfering beams are assumed to pass on either side of the solenoid.)
This phenomena is known as the Aharonov-Bohm effect [2], and has been observed experimentally [112].
is the net flux passing through the solenoid. In other
words, the magnetic field internal to the solenoid affects the interference pattern seen on the screen, despite the
fact that neither electron beam ever directly experiences a magnetic field (because the field is internal to the
solenoid, and the two interfering beams are assumed to pass on either side of the solenoid.)
This phenomena is known as the Aharonov-Bohm effect [2], and has been observed experimentally [112].



Next: Exercises
Up: Quantum Dynamics
Previous: Gauge Transformations in Electromagnetism
Richard Fitzpatrick
2016-01-22
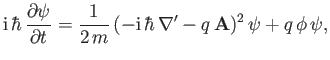
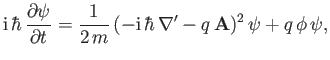

![$\displaystyle = \frac{q}{\hbar}\left[\int_1 d{\bf x}''\cdot{\bf A}({\bf x}'')-\int_2 d{\bf x}''\cdot{\bf A}({\bf x}'')\right]$](img814.png)
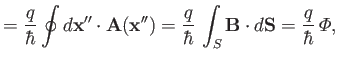
![]() passes. Upon completing a circuit around the hole, the
particle's wavefunction is multiplied by
passes. Upon completing a circuit around the hole, the
particle's wavefunction is multiplied by
![]() . The requirement that the wavefunction be
single-valued, and, hence, that the multiplication factor be unity, implies that the enclosed magnetic flux is quantized.
In fact.
. The requirement that the wavefunction be
single-valued, and, hence, that the multiplication factor be unity, implies that the enclosed magnetic flux is quantized.
In fact.