Next: Ionospheric Radio Wave Propagation Up: Waves in Inhomogeneous Plasmas Previous: Pulse Propagation Contents
A general wave problem can be written as a set of 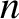 coupled, linear, homogeneous,
first-order, partial-differential equations, which take the form (Hazeltine and Waelbroeck 2004)
coupled, linear, homogeneous,
first-order, partial-differential equations, which take the form (Hazeltine and Waelbroeck 2004)
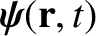 has
has 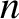 components (e.g.,
components (e.g.,
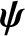 might consist of
might consist of 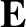 ,
, 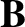 ,
,  , and
, and 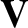 ) characterizing
some small disturbance, and
) characterizing
some small disturbance, and 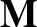 is an
is an 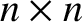 matrix characterizing the
undisturbed plasma.
matrix characterizing the
undisturbed plasma.
The lowest order WKB approximation is premised on the assumption that
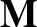 depends so weakly on
depends so weakly on 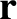 and
and 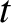 that all of the
spatial and temporal dependence of the components of
that all of the
spatial and temporal dependence of the components of
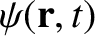 is specified by a common factor
is specified by a common factor
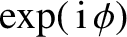 .
Thus,
Equation (6.87) reduces to
.
Thus,
Equation (6.87) reduces to
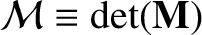 .
As is easily demonstrated (see Section 6.2), the WKB approximation is valid
provided that the characteristic
variation lengthscale and variation timescale of the plasma are much longer than
the wavelength,
.
As is easily demonstrated (see Section 6.2), the WKB approximation is valid
provided that the characteristic
variation lengthscale and variation timescale of the plasma are much longer than
the wavelength,  , and the period,
, and the period,
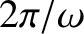 , respectively,
of the wave in question.
, respectively,
of the wave in question.
Let us concentrate on one particular solution of Equation (6.88) (e.g., on one particular type of plasma wave). For this solution, the dispersion relation (6.91) yields
that is, the dispersion relation yields a unique frequency for a wave of a given wavevector,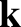 , located
at a given point,
, located
at a given point,
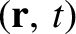 , in space and time. There is also a unique
, in space and time. There is also a unique
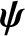 associated
with this frequency, which is obtained from Equation (6.88). To lowest order, we can
neglect the variation of
associated
with this frequency, which is obtained from Equation (6.88). To lowest order, we can
neglect the variation of
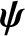 with
with 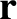 and
and 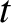 .
A general pulse solution is written
where (locally)
.
A general pulse solution is written
where (locally)
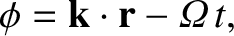 |
(6.94) |
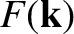 is a function that specifies the initial structure of the pulse
in
is a function that specifies the initial structure of the pulse
in 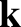 -space.
-space.
The integral (6.93) averages to zero, except at a point of stationary
phase, where
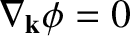 . (See Section 6.7.) Here,
. (See Section 6.7.) Here,
 is the
is the 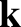 -space
gradient operator. It follows that the (instantaneous) trajectory of the pulse
matches that of a point of stationary phase:
-space
gradient operator. It follows that the (instantaneous) trajectory of the pulse
matches that of a point of stationary phase:
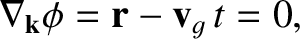 |
(6.95) |
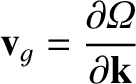 |
(6.96) |
Let us now determine how the wavevector, 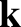 , and the angular frequency,
, and the angular frequency, 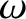 ,
of a pulse evolve as the pulse propagates through the
plasma. We start from the cross-differentiation rules
[see Equations (6.89) and (6.90)]:
,
of a pulse evolve as the pulse propagates through the
plasma. We start from the cross-differentiation rules
[see Equations (6.89) and (6.90)]:
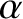 and
and 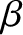 run from 1 to 3, and denote Cartesian components.
Equations (6.92), (6.97), and (6.98) yield [making use of the Einstein summation
convention (Riley 1974)]
run from 1 to 3, and denote Cartesian components.
Equations (6.92), (6.97), and (6.98) yield [making use of the Einstein summation
convention (Riley 1974)]
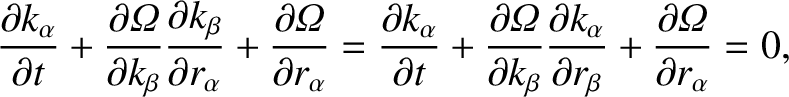 |
(6.99) |
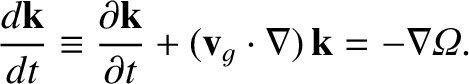 |
(6.100) |
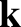 , as seen in a frame co-moving with
the pulse, is determined by the spatial gradients in
, as seen in a frame co-moving with
the pulse, is determined by the spatial gradients in
 .
.
Partial differentiation of Equation (6.92) with respect to 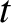 gives
gives
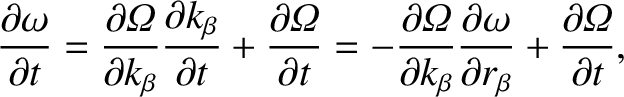 |
(6.101) |
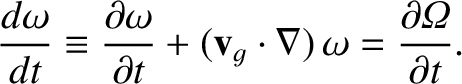 |
(6.102) |
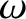 , as seen in a frame co-moving with
the pulse, is determined by the time variation of
, as seen in a frame co-moving with
the pulse, is determined by the time variation of
 .
.
According to the previous analysis, the evolution of a pulse propagating though a spatially and temporally non-uniform plasma can be determined by solving the ray equations:
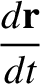 |
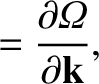 |
(6.103) |
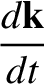 |
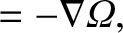 |
(6.104) |
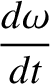 |
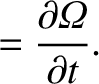 |
(6.105) |