


Next: Flux Freezing
Up: Magnetohydrodynamic Fluids
Previous: Introduction
The MHD equations can be combined with the Ampère- and Faraday-Maxwell equations,
respectively,
to form a closed set. The displacement current is neglected in
Equation (7.7) on the reasonable assumption that MHD motions are slow
compared to the velocity of light. Equation (7.8) guarantees
that
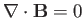 , provided that this relation is
presumed to hold initially. Furthermore, the assumption of
quasi-neutrality renders the Poisson-Maxwell equation,
, provided that this relation is
presumed to hold initially. Furthermore, the assumption of
quasi-neutrality renders the Poisson-Maxwell equation,
 , redundant.
, redundant.
Equations (7.2) and (7.7) can be combined to give the MHD equation
of motion:
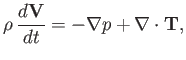 |
(7.9) |
where
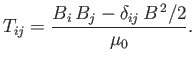 |
(7.10) |
Suppose that the magnetic field is approximately uniform, and
directed along the  -axis. In this case, the previous equation of
motion reduces to
-axis. In this case, the previous equation of
motion reduces to
 |
(7.11) |
where
![$\displaystyle {\bf P} = \left(\begin{array}{ccc} p + B^{\,2}/2\,\mu_0, & 0, &0\...
... B^{\,2}/2\,\mu_0,& 0\\ [0.5ex] 0,&0,& p - B^{\,2}/2\,\mu_0\end{array} \right).$](img2264.png) |
(7.12) |
It can be seen that the magnetic field increases the plasma pressure, by an
amount
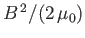 , in directions perpendicular to the magnetic field,
and decreases the plasma pressure, by the same amount, in the
parallel direction. Thus, the magnetic field gives rise to
a magnetic pressure,
, in directions perpendicular to the magnetic field,
and decreases the plasma pressure, by the same amount, in the
parallel direction. Thus, the magnetic field gives rise to
a magnetic pressure,
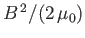 , acting perpendicular to field-lines,
and a magnetic tension,
, acting perpendicular to field-lines,
and a magnetic tension,
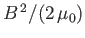 , acting
along field-lines.
Because, as will become apparent in the next section, the plasma is tied to magnetic field-lines,
it follows that magnetic field-lines embedded in an
MHD plasma act rather like
mutually repulsive elastic bands.
, acting
along field-lines.
Because, as will become apparent in the next section, the plasma is tied to magnetic field-lines,
it follows that magnetic field-lines embedded in an
MHD plasma act rather like
mutually repulsive elastic bands.



Next: Flux Freezing
Up: Magnetohydrodynamic Fluids
Previous: Introduction
Richard Fitzpatrick
2016-01-23
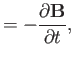
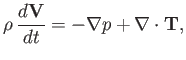
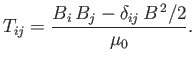
![]() -axis. In this case, the previous equation of
motion reduces to
-axis. In this case, the previous equation of
motion reduces to

![$\displaystyle {\bf P} = \left(\begin{array}{ccc} p + B^{\,2}/2\,\mu_0, & 0, &0\...
... B^{\,2}/2\,\mu_0,& 0\\ [0.5ex] 0,&0,& p - B^{\,2}/2\,\mu_0\end{array} \right).$](img2264.png)