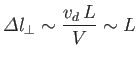Next: Langmuir Sheaths
Up: Plasma Fluid Theory
Previous: Drift Equations
Closure in Collisionless Magnetized Plasmas
Up to now, we have only considered fluid closure in collisional magnetized
plasmas.
Unfortunately, the majority of magnetized plasmas encountered in nature--in particular,
fusion, space, and astrophysical plasmas--are collisionless.
Let us consider what happens to the cold-plasma equations, the MHD equations,
and the drift equations, in the limit in which the mean-free-path goes
to infinity (i.e.,
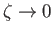 ).
).
In the limit
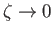 , the cold-plasma equations reduce to
, the cold-plasma equations reduce to
Here, we have neglected the resistivity term, because it is
 .
Note that none of the remaining terms in these equations depend
explicitly on collisions. Nevertheless, the absence of collisions
poses a serious problem. Whereas the magnetic field effectively confines
charged particles in directions perpendicular to magnetic field-lines,
by forcing them to execute tight Larmor orbits, we have now lost all
confinement along field-lines. But, does this matter?
.
Note that none of the remaining terms in these equations depend
explicitly on collisions. Nevertheless, the absence of collisions
poses a serious problem. Whereas the magnetic field effectively confines
charged particles in directions perpendicular to magnetic field-lines,
by forcing them to execute tight Larmor orbits, we have now lost all
confinement along field-lines. But, does this matter?
The typical frequency associated with fluid motion
is the transit frequency,  . However, according to Equation (4.173),
the cold-plasma ordering implies that the transit frequency is of similar magnitude to a
typical gyrofrequency:
. However, according to Equation (4.173),
the cold-plasma ordering implies that the transit frequency is of similar magnitude to a
typical gyrofrequency:
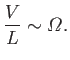 |
(4.239) |
So, how far is a charged particle likely to drift along a field-line in
an inverse transit frequency? The answer is
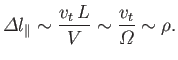 |
(4.240) |
In other words, the fluid motion in the cold-plasma limit is so fast that
charged particles only have time to drift a Larmor radius along field-lines
on a typical dynamical timescale. Under these circumstances, it does not
really matter that the particles are not localized along field-lines--the lack
of parallel confinement manifests itself too slowly
to affect the plasma dynamics. We conclude, therefore, that the cold-plasma
equations remain valid in the collisionless limit, provided, of course,
that the
plasma dynamics are sufficiently rapid for the basic cold-plasma ordering
(4.239) to apply. In fact, the only difference between the collisional and
collisionless cold-plasma equations is the absence of the resistivity term in Ohm's law in the
latter case.
Let us now consider the MHD limit. In this case, the typical
transit frequency is
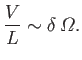 |
(4.241) |
Thus, charged particles typically drift a distance
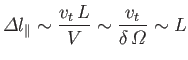 |
(4.242) |
along field-lines in an inverse transit frequency. In other words, the
fluid motion in the MHD limit is sufficiently slow that changed particles
have time to drift along field-lines all the way across the system
on a
typical dynamical time-scale. Thus, strictly speaking, the MHD equations
are invalidated by the lack of particle confinement along magnetic
field-lines.
In fact, in collisionless plasmas, MHD theory is replaced by a theory
known as kinetic-MHD (Kruskal and Oberman 1958; Rosenbluth and Rostoker 1959).
The latter theory is a combination of a
one-dimensional kinetic theory, describing particle motion along magnetic
field-lines, and a two-dimensional fluid theory, describing perpendicular
motion. Unfortunately, the equations of kinetic-MHD are
considerably more complicated that the conventional MHD equations.
Is there any situation in which we can salvage the simpler MHD equations in
a collisionless plasma? Fortunately, there
is one case in which this is possible.
It turns out that in both varieties of MHD the motion of the plasma
parallel to magnetic field-lines is associated with the dynamics of
sound waves, whereas the motion perpendicular to field-lines
is associated with the dynamics of a new type of wave called an Alfvén
wave. As we shall see, in Chapter 5, Alfvén
waves involve the ``twanging'' motion of magnetic field-lines--a bit
like the twanging of guitar strings. It is only the sound wave dynamics
that are significantly modified when we move from a collisional to a
collisionless plasma. It follows, therefore, that the MHD equations
remain a reasonable approximation in a collisionless plasma in situations where
the dynamics of sound waves, parallel to the magnetic field, are unimportant
compared to the dynamics of Alfvén waves, perpendicular to the field.
This situation arises whenever the parameter
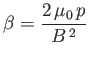 |
(4.243) |
(see Section 1.9) is much less than unity. In fact, it is easily
demonstrated that
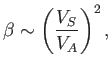 |
(4.244) |
where 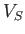 is the sound speed (i.e., thermal velocity), and
is the sound speed (i.e., thermal velocity), and 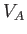 is the speed of an Alfvén wave. Thus, the inequality
is the speed of an Alfvén wave. Thus, the inequality
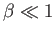 |
(4.245) |
ensures that the collisionless
parallel plasma dynamics are too slow to affect the
perpendicular dynamics.
We conclude, therefore, that in a low-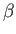 , collisionless, magnetized
plasma, the MHD equations,
, collisionless, magnetized
plasma, the MHD equations,
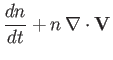 |
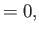 |
(4.246) |
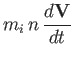 |
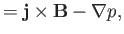 |
(4.247) |
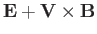 |
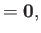 |
(4.248) |
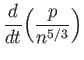 |
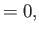 |
(4.249) |
describe fairly well plasma dynamics that satisfy the basic MHD ordering
(4.241).
Let us, finally, consider the drift limit. In this case, the typical
transit frequency is
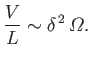 |
(4.250) |
Thus, charged particles
typically drift a distance
 |
(4.251) |
along field-lines in an inverse transit frequency. In other
words, the fluid motion in the drift limit is so slow
that charged particles drifting along
field-lines have time to traverse the system very many times on a typical
dynamical time-scale. In fact, in this limit we have to
draw a distinction between those particles that always drift along field-lines
in the same direction, and those particles that are trapped between magnetic
mirror points and, therefore, continually reverse their direction of motion
along field-lines. The former are termed passing particles, whereas
the latter are termed trapped particles.
Now, in the drift limit, the perpendicular drift velocity of
charged particles, which is a combination of
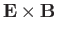 drift,
grad-
drift,
grad-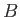 drift, and curvature drift (see Chapter 2), is approximately
drift, and curvature drift (see Chapter 2), is approximately
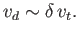 |
(4.252) |
Thus, charged particles typically drift a distance
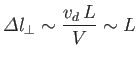 |
(4.253) |
across field-lines in an inverse transit time. In other words, the
fluid motion in the drift limit is so slow that charged particles
have time
to drift perpendicular to field-lines all the way across the system on a
typical dynamical time-scale.
It is, thus, clear that in the drift limit the absence of collisions implies
lack of confinement both parallel and perpendicular to the magnetic
field. This means that the collisional drift equations, (4.219)-(4.221) and
(4.222)-(4.224), are completely invalid in the long mean-free-path limit.
In fact, in collisionless plasmas, Braginskii-type transport theory--conventionally
known as classical transport theory--is replaced by a new theory--known as
neoclassical transport theory--which is a combination of a two-dimensional
kinetic theory, describing particle motion on drift surfaces, and
a one-dimensional fluid theory, describing motion perpendicular to the drift
surfaces (Bernstein 1974; Hinton and Hazeltine 1976). Here, a drift surface is a closed surface formed by the locus of a
charged particle's drift orbit (including
drifts parallel and perpendicular to the magnetic field).
Of course, the orbits only form closed surfaces if the plasma is confined,
but there is little point in examining transport in an unconfined plasma.
Unlike classical
transport theory, which is strictly local in nature,
neoclassical transport theory is nonlocal, in the sense that the
transport coefficients depend on the average values of
plasma properties taken over
drift surfaces.



Next: Langmuir Sheaths
Up: Plasma Fluid Theory
Previous: Drift Equations
Richard Fitzpatrick
2016-01-23
![]() , the cold-plasma equations reduce to
, the cold-plasma equations reduce to
![]() . However, according to Equation (4.173),
the cold-plasma ordering implies that the transit frequency is of similar magnitude to a
typical gyrofrequency:
. However, according to Equation (4.173),
the cold-plasma ordering implies that the transit frequency is of similar magnitude to a
typical gyrofrequency:
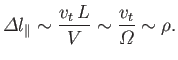
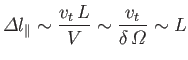
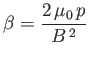
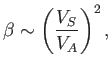
![]() , collisionless, magnetized
plasma, the MHD equations,
, collisionless, magnetized
plasma, the MHD equations,
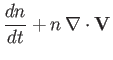
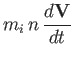
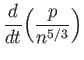
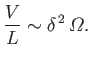

![]() drift,
grad-
drift,
grad-![]() drift, and curvature drift (see Chapter 2), is approximately
drift, and curvature drift (see Chapter 2), is approximately