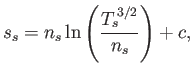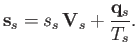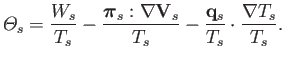Next: Fluid Closure
Up: Plasma Fluid Theory
Previous: Fluid Equations
It is instructive to rewrite the species- energy evolution equation, Equation (4.49), as
an entropy evolution equation (Hazeltine and Waelbroeck 2004). The fluid definition of entropy
density, which coincides with the thermodynamic entropy density in the
limit that the distribution function approaches a Maxwellian, is (Reif 1965)
energy evolution equation, Equation (4.49), as
an entropy evolution equation (Hazeltine and Waelbroeck 2004). The fluid definition of entropy
density, which coincides with the thermodynamic entropy density in the
limit that the distribution function approaches a Maxwellian, is (Reif 1965)
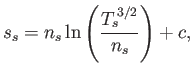 |
(4.51) |
where 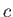 is a constant.
The corresponding entropy flux density is written
is a constant.
The corresponding entropy flux density is written
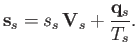 |
(4.52) |
Clearly, entropy is convected by the fluid flow, but is also carried by the
flow of heat, in accordance with the second law of thermodynamics (Reif 1965). After some
algebra, Equation (4.49) can be rearranged to give
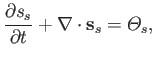 |
(4.53) |
where the right-hand side is given by
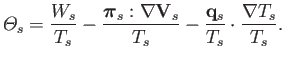 |
(4.54) |
It follows, from our previous discussion of conservation laws, that the
quantity
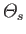 can be regarded as the entropy production rate
per unit volume for species
can be regarded as the entropy production rate
per unit volume for species  . Evidently, entropy is produced by
collisional heating, viscous heating, and heat flow down temperature
gradients.
. Evidently, entropy is produced by
collisional heating, viscous heating, and heat flow down temperature
gradients.



Next: Fluid Closure
Up: Plasma Fluid Theory
Previous: Fluid Equations
Richard Fitzpatrick
2016-01-23