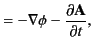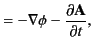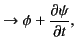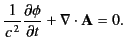Next: Retarded Potentials
Up: Relativity and Electromagnetism
Previous: Potential 4-Vector
Gauge Invariance
The electric and magnetic fields are obtained from the vector and
scalar potentials according to the prescription
These fields are important because they determine the electromagnetic forces
exerted on charged particles. Note that the previous prescription does not
uniquely determine the two potentials. It is possible to make the
following transformation, known as a gauge transformation, that
leaves the fields unaltered:
where
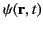 is a general scalar field. It is necessary
to adopt some form of convention, generally known as a gauge condition,
to fully specify the two potentials. In fact, there is only one gauge condition
that is consistent with Equations (1733). This is the Lorenz gauge
condition,
is a general scalar field. It is necessary
to adopt some form of convention, generally known as a gauge condition,
to fully specify the two potentials. In fact, there is only one gauge condition
that is consistent with Equations (1733). This is the Lorenz gauge
condition,
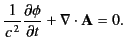 |
(1742) |
Note that this condition can be written in the Lorentz invariant form
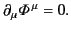 |
(1743) |
This implies that if the Lorenz gauge holds in one particular inertial
frame then it automatically holds in all
other inertial frames. A general gauge transformation
can be written
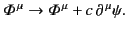 |
(1744) |
Note that, even after the Lorentz gauge has been adopted, the potentials
are undetermined to a gauge transformation using a scalar field, 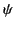 , that
satisfies the sourceless wave equation
, that
satisfies the sourceless wave equation
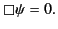 |
(1745) |
However, if we adopt sensible boundary conditions in both
space and time then the only solution to the previous equation is 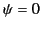 .
.



Next: Retarded Potentials
Up: Relativity and Electromagnetism
Previous: Potential 4-Vector
Richard Fitzpatrick
2014-06-27