


Next: Reflection Coefficient
Up: Wave Propagation in Inhomogeneous
Previous: Ionospheric Radio Wave Propagation
WKB Approximation
Consider a radio wave that is vertically incident, from below, on a
horizontally stratified ionosphere. Because the wave normal is initially aligned along
the  -axis,
and as
-axis,
and as 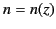 , we expect
all field components to be functions of
, we expect
all field components to be functions of  only, so that
only, so that
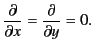 |
(1060) |
In this situation, Equations (1058)-(1061) reduce to
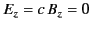 , with
, with
and
Note that Equations (1063)-(1064) and (1065)-(1066) are isomorphic and
completely independent of one another. It follows that, without loss
of generality, we can assume that the wave is linearly polarized with
its electric vector parallel to the 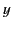 -axis. In other words, we need
only consider the solution of Equations (1063)-(1064). The solution of
Equations (1065)-(1066) is of exactly the same form, except that it describes
a linear polarized wave with its electric vector parallel to the
-axis. In other words, we need
only consider the solution of Equations (1063)-(1064). The solution of
Equations (1065)-(1066) is of exactly the same form, except that it describes
a linear polarized wave with its electric vector parallel to the  -axis.
-axis.
Equations (1063)-(1064) can be combined to give
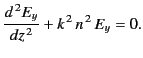 |
(1065) |
Incidentally, because 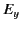 is a function of
is a function of  only, we now use the total derivative
sign
only, we now use the total derivative
sign 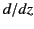 , instead of the partial derivative sign
, instead of the partial derivative sign
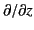 .
The solution of the previous equation for the case of a uniform medium, where
.
The solution of the previous equation for the case of a uniform medium, where
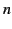 is constant, is straightforward:
is constant, is straightforward:
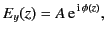 |
(1066) |
where 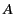 is a constant, and
is a constant, and
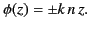 |
(1067) |
Note that the
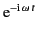 time dependence of all
wave quantities is taken as read during this investigation. The solution (1068)
represents a wave of constant amplitude
time dependence of all
wave quantities is taken as read during this investigation. The solution (1068)
represents a wave of constant amplitude 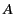 and phase
and phase 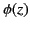 . According to Equation (1069),
there are, in fact, two independent waves that can propagate through the medium in question.
The upper sign corresponds to a wave that propagates vertically upwards,
whereas the lower sign corresponds to a wave that propagates vertically
downwards. Both waves propagate at the constant phase velocity
. According to Equation (1069),
there are, in fact, two independent waves that can propagate through the medium in question.
The upper sign corresponds to a wave that propagates vertically upwards,
whereas the lower sign corresponds to a wave that propagates vertically
downwards. Both waves propagate at the constant phase velocity 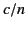 .
.
In general, if 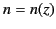 then the solution to Equation (1067) does not remotely resemble
the wave-like solution (1068). However, in the limit that
then the solution to Equation (1067) does not remotely resemble
the wave-like solution (1068). However, in the limit that 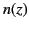 is
a ``slowly varying'' function of
is
a ``slowly varying'' function of  (exactly how slowly varying is something that
will be established later), we expect to recover wave-like solutions.
Let us suppose that
(exactly how slowly varying is something that
will be established later), we expect to recover wave-like solutions.
Let us suppose that 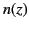 is indeed a slowly varying function, and let us try
substituting the wave solution (1068) into Equation (1067). We obtain
is indeed a slowly varying function, and let us try
substituting the wave solution (1068) into Equation (1067). We obtain
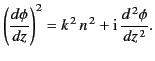 |
(1068) |
which is a non-linear differential equation that, in general, is very difficult
to solve. However, if 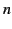 is a constant then
is a constant then
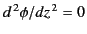 .
It is, therefore, reasonable to suppose that if
.
It is, therefore, reasonable to suppose that if 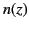 is a slowly varying function
then the last term on the right-hand side of the previous equation can be
regarded as small. Thus, to a first approximation, Equation (1070) yields
is a slowly varying function
then the last term on the right-hand side of the previous equation can be
regarded as small. Thus, to a first approximation, Equation (1070) yields
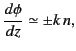 |
(1069) |
and
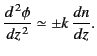 |
(1070) |
It is clear from a comparison between Equations (1070) and (1072) that 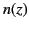 can
be regarded as a slowly varying function of
can
be regarded as a slowly varying function of  as long as its variation
length-scale is far longer than the wavelength of the wave.
In other words, provided
as long as its variation
length-scale is far longer than the wavelength of the wave.
In other words, provided
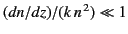 .
.
The second approximation to the solution is obtained by substituting Equation (1072) into
the right-hand side of Equation (1070):
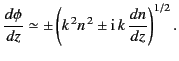 |
(1071) |
This gives
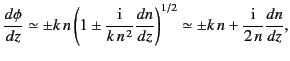 |
(1072) |
where use has been made of the binomial expansion. The previous expression can be integrated
to give
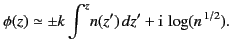 |
(1073) |
Substitution of Equation (1075) into Equation (1068) yields the final result
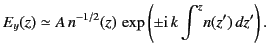 |
(1074) |
It follows from Equation (4.183) that
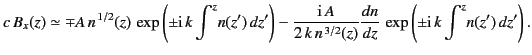 |
(1075) |
Note that the second term on the right-hand side of the previous expression is small compared to the first, and can usually be neglected.
Let us test to what extent the expression (1076) is a good solution
of Equation (1067) by substituting the former into the left-hand side
of the latter. The result is
![$\displaystyle \frac{A}{n^{\,1/2}}\left[ \frac{3}{4}\left(\frac{1}{n} \frac{dn}{...
...2} n}{dz^{\,2}}\right] {\rm exp}\left( \pm {\rm i}\,k\int^z\!n(z')\,dz'\right),$](img2258.png) |
(1076) |
which must be small compared with either term on the left-hand side
of (1067). Hence, the
condition for expression (1076) to be a good solution of Equation (1067)
becomes
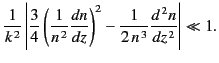 |
(1077) |
The solution
to the non-uniform wave equations (1063)-(1064) is usually
called the WKB solution,
in honor of G. Wentzel, H.A. Kramers, and L. Brillouin, who are credited with
independently discovering it
(in a quantum mechanical context) in 1926. Actually, H. Jeffries wrote
a paper on this solution
(in a wave propagation context) in 1923. Hence, some people call it the WKBJ
solution (or even the JWKB solution). In fact, this solution was
first discussed
by Liouville and Green in 1837, and again by Rayleigh in 1912.
In the following, we refer to (1080)-(1081)
as the WKB solution, because this is what it is generally called. However,
it should be understand that, in doing so,
we are not making any statement as to the credit due
to various scientists in discovering this solution.
As is well known, if a propagating electromagnetic wave is normally incident on an interface
where the
refractive index suddenly changes (for instance, when a light
wave propagating in the air is normally incident on a glass slab) then there is generally
significant reflection of the wave. However, according to the WKB solution,
(1080)-(1081), if a propagating wave is normally incident on a medium in which
the refractive index changes slowly along the direction of propagation of the
wave then the wave is not reflected at all. This is true
even if the refractive index
varies very substantially along the path of the wave,
as long as it varies slowly. The WKB
solution implies that as the wave propagates through the medium its wavelength
gradually changes. In fact, the wavelength at position  is approximately
is approximately
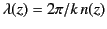 . Equations (1080)-(1081) also imply that the amplitude
of the wave gradually changes as it propagates. In fact, the amplitude of the electric
field component is inversely proportional to
. Equations (1080)-(1081) also imply that the amplitude
of the wave gradually changes as it propagates. In fact, the amplitude of the electric
field component is inversely proportional to 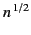 , whereas the amplitude of the
magnetic field component is directly proportional to
, whereas the amplitude of the
magnetic field component is directly proportional to 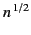 .
Note, however, that the energy
flux in the
.
Note, however, that the energy
flux in the  -direction, which is given by the the Poynting vector
-direction, which is given by the the Poynting vector
 , remains constant (assuming that
, remains constant (assuming that 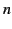 is predominately
real).
is predominately
real).
Of course, the WKB solution, (1080)-(1081), is only approximate. In reality,
a wave propagating into a medium in which the refractive index is a slowly
varying function of position is subject to a small amount of reflection.
However, it is easily demonstrated that the ratio of the reflected amplitude
to the incident amplitude is of order
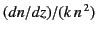 . Thus, as long as
the refractive index varies on a much longer length-scale than the wavelength
of the radiation, the reflected wave is negligibly small. This conclusion remains
valid as long as the inequality (1079) is satisfied. There are two
main reasons
why this inequality might fail to be satisfied. First of all, if there is
a localized region in the dielectric medium where the refractive index suddenly
changes (i.e., if there is an interface) then
(1079) is likely to break down in this region, allowing strong reflection
of the incident wave. Secondly, the inequality obviously
breaks down in the vicinity of a point where
. Thus, as long as
the refractive index varies on a much longer length-scale than the wavelength
of the radiation, the reflected wave is negligibly small. This conclusion remains
valid as long as the inequality (1079) is satisfied. There are two
main reasons
why this inequality might fail to be satisfied. First of all, if there is
a localized region in the dielectric medium where the refractive index suddenly
changes (i.e., if there is an interface) then
(1079) is likely to break down in this region, allowing strong reflection
of the incident wave. Secondly, the inequality obviously
breaks down in the vicinity of a point where 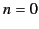 . We would, therefore,
expect strong reflection of the incident wave from such a point.
. We would, therefore,
expect strong reflection of the incident wave from such a point.



Next: Reflection Coefficient
Up: Wave Propagation in Inhomogeneous
Previous: Ionospheric Radio Wave Propagation
Richard Fitzpatrick
2014-06-27
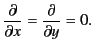
![]() then the solution to Equation (1067) does not remotely resemble
the wave-like solution (1068). However, in the limit that
then the solution to Equation (1067) does not remotely resemble
the wave-like solution (1068). However, in the limit that ![]() is
a ``slowly varying'' function of
is
a ``slowly varying'' function of ![]() (exactly how slowly varying is something that
will be established later), we expect to recover wave-like solutions.
Let us suppose that
(exactly how slowly varying is something that
will be established later), we expect to recover wave-like solutions.
Let us suppose that ![]() is indeed a slowly varying function, and let us try
substituting the wave solution (1068) into Equation (1067). We obtain
is indeed a slowly varying function, and let us try
substituting the wave solution (1068) into Equation (1067). We obtain
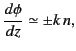
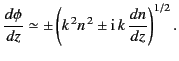
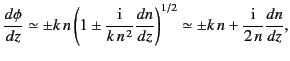
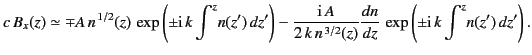
![$\displaystyle \frac{A}{n^{\,1/2}}\left[ \frac{3}{4}\left(\frac{1}{n} \frac{dn}{...
...2} n}{dz^{\,2}}\right] {\rm exp}\left( \pm {\rm i}\,k\int^z\!n(z')\,dz'\right),$](img2258.png)
![]() is approximately
is approximately
![]() . Equations (1080)-(1081) also imply that the amplitude
of the wave gradually changes as it propagates. In fact, the amplitude of the electric
field component is inversely proportional to
. Equations (1080)-(1081) also imply that the amplitude
of the wave gradually changes as it propagates. In fact, the amplitude of the electric
field component is inversely proportional to ![]() , whereas the amplitude of the
magnetic field component is directly proportional to
, whereas the amplitude of the
magnetic field component is directly proportional to ![]() .
Note, however, that the energy
flux in the
.
Note, however, that the energy
flux in the ![]() -direction, which is given by the the Poynting vector
-direction, which is given by the the Poynting vector
![]() , remains constant (assuming that
, remains constant (assuming that ![]() is predominately
real).
is predominately
real).
![]() . Thus, as long as
the refractive index varies on a much longer length-scale than the wavelength
of the radiation, the reflected wave is negligibly small. This conclusion remains
valid as long as the inequality (1079) is satisfied. There are two
main reasons
why this inequality might fail to be satisfied. First of all, if there is
a localized region in the dielectric medium where the refractive index suddenly
changes (i.e., if there is an interface) then
(1079) is likely to break down in this region, allowing strong reflection
of the incident wave. Secondly, the inequality obviously
breaks down in the vicinity of a point where
. Thus, as long as
the refractive index varies on a much longer length-scale than the wavelength
of the radiation, the reflected wave is negligibly small. This conclusion remains
valid as long as the inequality (1079) is satisfied. There are two
main reasons
why this inequality might fail to be satisfied. First of all, if there is
a localized region in the dielectric medium where the refractive index suddenly
changes (i.e., if there is an interface) then
(1079) is likely to break down in this region, allowing strong reflection
of the incident wave. Secondly, the inequality obviously
breaks down in the vicinity of a point where ![]() . We would, therefore,
expect strong reflection of the incident wave from such a point.
. We would, therefore,
expect strong reflection of the incident wave from such a point.