...Principa.

An excellent discussion of the historical development of Newtonian dynamics, as well as the physical and philosophical assumptions which underpin this theory, is given in The Discovery of Dynamics: A Study from a Machian Point of View of the Discovery and the Structure of Dynamical Theories, J.B. Barbour (Oxford University Press, Oxford UK, 2001).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... tensor.

A tensor is the two-dimensional generalization of a vector. However, for present purposes, we can simply think of a tensor as another name for a matrix.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... radius.

M.G. Stewart, American Jou. Physics 73, 730 (2005).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... years.

Note that this precession rate is about 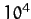$10^4$ times greater than any of the planetary perihelion precession rates discussed in Section 12.12.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... dynamics.

For more information on lunar theory, see An Elementary Treatise on the Lunar Theory, H. Godfray (Macmillan & Co., 1853); An Introductory Treatise on the Lunar Theory, E.W. Brown (Cambridge University Press, 1896); Lectures on the Lunar Theory, J.C. Adams (Cambridge University Press, 1900).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... month,

A synodic month, which is 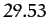$29.53$ days, is the mean period between successive new moons.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... scheme.

W.H. Press, S.A. Teukolsky, W.T. Vetterling, and B.P. Flannery, Numerical recipes in C: The art of scientific computing, 2nd Edition (Cambridge University Press, Cambridge UK, 1992), Section 16.1.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... respectively.

G.L. Baker, Control of the chaotic driven pendulum, Am. J. Phys. 63, 832 (1995).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... interactions.

E.S. Albers and B.W. Lee, Phys. Rep. 9C, 1 (1973).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... universe.

P. Coles, and F. Lucchin, Cosmology: The origin and evolution of cosmic structure, (J. Wiley & Sons, Chichester UK, 1995).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...fractal.

B.B. Mandelbrot, The fractal geometry of nature, (W.H. Freeman, New York NY, 1982).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... bifurcations.

M.J. Feigenbaum, Quantitative universality for a class of nonlinear transformations, J. Stat. Phys. 19, 25 (1978).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... bifurcations.

M.J. Feigenbaum, The universal metric properties of nonlinear transformations, J. Stat. Phys. 21, 69 (1979).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... systems.

P. Citanovic, Universality in chaos, (Adam Hilger, Bristol UK, 1989).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... ground.

E. Lorenz, Deterministic nonperiodic flow, J. Atmospheric Science 20, 130 (1963).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... increased.

N. Metropolis, M.L. Stein, and P.R. Stein, On finite limit sets for transformations on the unit interval, J. Combin. Theor. 15, 25 (1973).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... systems.

R.H. Simoyi, A. Wolf, and H.L. Swinney, One-dimensional dynamics in a multi-component chemical reaction, Phys. Rev. Lett. 49, 245 (1982).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.