


Next: Volume Integrals
Up: Vector Algebra and Vector
Previous: Line Integrals
A vector field is defined as a set of vectors associated with each point in space.
For instance, the velocity
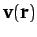 in a moving liquid
(e.g., a whirlpool) constitutes
a vector field. By analogy, a scalar field is a set of scalars associated with each
point in space. An example of a scalar field is the temperature distribution
in a moving liquid
(e.g., a whirlpool) constitutes
a vector field. By analogy, a scalar field is a set of scalars associated with each
point in space. An example of a scalar field is the temperature distribution 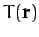 in
a furnace.
in
a furnace.
Consider a general vector field
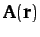 . Let
. Let
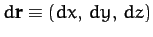 be the
vector element of line length. Vector line integrals often arise as
be the
vector element of line length. Vector line integrals often arise as
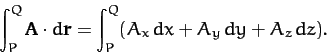 |
(1338) |
For instance, if 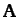 is a force-field then the line integral is the work done in going from
is a force-field then the line integral is the work done in going from
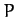 to
to 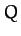 .
.
As an example, consider the work done by a repulsive inverse-square
central field,
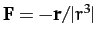 . The
element of work done is
. The
element of work done is
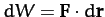 .
Take
.
Take
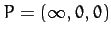 and
and 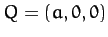 . Route 1 is along the
. Route 1 is along the 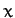 -axis, so
-axis, so
![\begin{displaymath}
W = \int_{\infty}^a \left(-\frac{1}{x^2}\right)\,dx = \left[\frac{1}{x}\right]_{\infty}^a
=\frac{1}{a}.
\end{displaymath}](img3413.png) |
(1339) |
The second route is, firstly, around a large circle (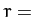 constant) to the point
(
constant) to the point
(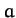 ,
, 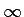 , 0), and then parallel to the
, 0), and then parallel to the 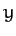 -axis--see Figure A.110. In the first part, no work is
done,
since
-axis--see Figure A.110. In the first part, no work is
done,
since 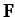 is perpendicular to
is perpendicular to 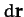 . In the second part,
. In the second part,
![\begin{displaymath}
W = \int_{\infty}^0 \frac{-y\,dy}{(a^2 + y^2)^{3/2}} = \left[\frac{1}{(y^2+a^2)^{1/2}}
\right]^0_\infty = \frac{1}{a}.
\end{displaymath}](img3416.png) |
(1340) |
In this case, the integral is independent of the path. However, not all vector line integrals
are path independent.
Figure A.110:
An example vector line integral.
 |



Next: Volume Integrals
Up: Vector Algebra and Vector
Previous: Line Integrals
Richard Fitzpatrick
2011-03-31
![]() . Let
. Let
![]() be the
vector element of line length. Vector line integrals often arise as
be the
vector element of line length. Vector line integrals often arise as
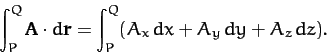
![]() . The
element of work done is
. The
element of work done is
![]() .
Take
.
Take
![]() and
and ![]() . Route 1 is along the
. Route 1 is along the ![]() -axis, so
-axis, so
![\begin{displaymath}
W = \int_{\infty}^a \left(-\frac{1}{x^2}\right)\,dx = \left[\frac{1}{x}\right]_{\infty}^a
=\frac{1}{a}.
\end{displaymath}](img3413.png)
![\begin{displaymath}
W = \int_{\infty}^0 \frac{-y\,dy}{(a^2 + y^2)^{3/2}} = \left[\frac{1}{(y^2+a^2)^{1/2}}
\right]^0_\infty = \frac{1}{a}.
\end{displaymath}](img3416.png)