


Next: Vector Area
Up: Vectors and Vector Fields
Previous: Coordinate Transformations
Scalar Product
A scalar quantity is invariant under all possible rotational transformations.
The individual components of a vector are not scalars because they change under
transformation. Can we form a scalar out of some combination of the components
of one, or more, vectors? Suppose that we were to define the
``percent'' product,
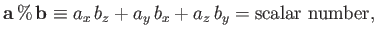 |
(A.23) |
for general vectors 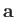 and
and 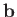 . Is
. Is
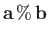 invariant under transformation, as must be the case if it is a scalar number?
Let us consider an example. Suppose that
invariant under transformation, as must be the case if it is a scalar number?
Let us consider an example. Suppose that
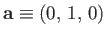 and
and
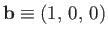 . It is easily seen that
. It is easily seen that
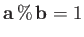 . Let
us now rotate the coordinate axes through
. Let
us now rotate the coordinate axes through 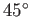 about
about 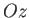 . In the new
coordinate system,
. In the new
coordinate system,
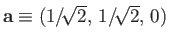 and
and
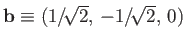 , giving
, giving
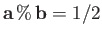 . Clearly,
. Clearly,
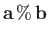 is not invariant under rotational transformation, so
the previous definition is a bad one.
is not invariant under rotational transformation, so
the previous definition is a bad one.
Consider, now,
the dot product or scalar product:
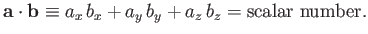 |
(A.24) |
Let us rotate the coordinate axes though  degrees about
degrees about  . According to
Equations (A.20)-(A.22),
. According to
Equations (A.20)-(A.22),
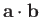 takes the form
takes the form
in the new coordinate system.
Thus,
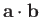 is invariant under rotation about
is invariant under rotation about 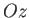 . It is easily
demonstrated that it is also invariant under rotation about
. It is easily
demonstrated that it is also invariant under rotation about 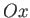 and
and 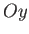 .
We conclude that
.
We conclude that
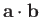 is a true scalar, and that the definition (A.24) is
a good one. Incidentally,
is a true scalar, and that the definition (A.24) is
a good one. Incidentally,
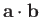 is the only
simple combination of
the components of two vectors that transforms like a scalar. It is readily
shown that the dot product is commutative and distributive: that is,
is the only
simple combination of
the components of two vectors that transforms like a scalar. It is readily
shown that the dot product is commutative and distributive: that is,
The associative property is meaningless for the dot product, because we cannot
have
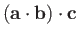 , as
, as
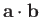 is scalar.
is scalar.
We have shown that the dot product
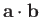 is coordinate independent.
But what is the geometric significance of this property? In the special case
where
is coordinate independent.
But what is the geometric significance of this property? In the special case
where
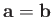 , we get
, we get
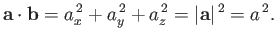 |
(A.27) |
So, the invariance of
 is equivalent to the invariance
of the magnitude of vector
is equivalent to the invariance
of the magnitude of vector 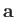 under transformation.
under transformation.
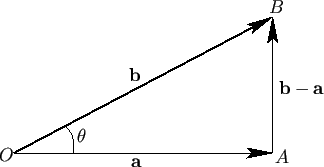
Let us now investigate the general case. The length squared of 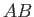 in the
vector triangle shown in Figure A.6 is
in the
vector triangle shown in Figure A.6 is
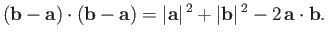 |
(A.28) |
However, according to the ``cosine rule'' of trigonometry,
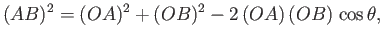 |
(A.29) |
where 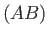 denotes the length of side
denotes the length of side 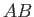 . It follows that
. It follows that
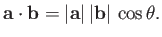 |
(A.30) |
In this case, the invariance of
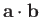 under transformation is equivalent
to the invariance of the angle subtended between the two vectors. Note that
if
under transformation is equivalent
to the invariance of the angle subtended between the two vectors. Note that
if
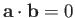 then either
then either
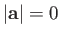 ,
,
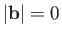 , or the vectors
, or the vectors
 and
and 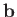 are mutually perpendicular. The angle subtended between two vectors
can easily be obtained from the dot product:
are mutually perpendicular. The angle subtended between two vectors
can easily be obtained from the dot product:
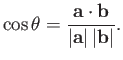 |
(A.31) |
Figure A.6:
A vector triangle.
 |
The work  performed by a constant force
performed by a constant force  that moves an object through a displacement
that moves an object through a displacement  is the product of the magnitude of
is the product of the magnitude of  times the displacement in the direction
of
times the displacement in the direction
of  . If the angle subtended between
. If the angle subtended between  and
and 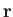 is
is  then
then
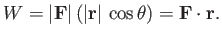 |
(A.32) |
The work 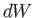 performed by a non-constant force
performed by a non-constant force 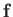 that moves an
object through an infinitesimal displacement
that moves an
object through an infinitesimal displacement 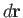 in a time interval
in a time interval 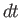 is
is
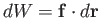 . Thus, the rate at which the force does
work on the object, which is usually referred to as the power, is
. Thus, the rate at which the force does
work on the object, which is usually referred to as the power, is
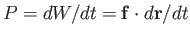 , or
, or
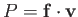 ,
where
,
where
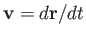 is the object's instantaneous velocity.
is the object's instantaneous velocity.



Next: Vector Area
Up: Vectors and Vector Fields
Previous: Coordinate Transformations
Richard Fitzpatrick
2016-01-22
![]() is coordinate independent.
But what is the geometric significance of this property? In the special case
where
is coordinate independent.
But what is the geometric significance of this property? In the special case
where
![]() , we get
, we get
![]() in the
vector triangle shown in Figure A.6 is
in the
vector triangle shown in Figure A.6 is
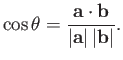
![]() performed by a constant force
performed by a constant force ![]() that moves an object through a displacement
that moves an object through a displacement ![]() is the product of the magnitude of
is the product of the magnitude of ![]() times the displacement in the direction
of
times the displacement in the direction
of ![]() . If the angle subtended between
. If the angle subtended between ![]() and
and ![]() is
is ![]() then
then
![]() performed by a non-constant force
performed by a non-constant force ![]() that moves an
object through an infinitesimal displacement
that moves an
object through an infinitesimal displacement ![]() in a time interval
in a time interval ![]() is
is
![]() . Thus, the rate at which the force does
work on the object, which is usually referred to as the power, is
. Thus, the rate at which the force does
work on the object, which is usually referred to as the power, is
![]() , or
, or
![]() ,
where
,
where
![]() is the object's instantaneous velocity.
is the object's instantaneous velocity.