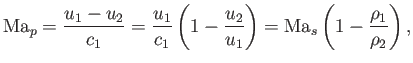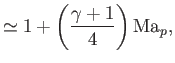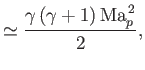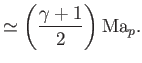Next: Piston-Generated Expansion Wave
Up: One-Dimensional Compressible Inviscid Flow
Previous: Normal Shocks
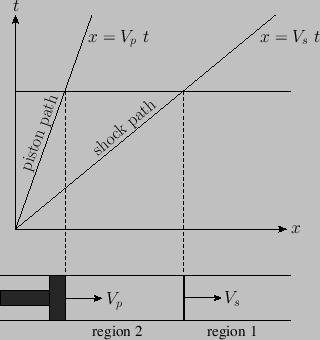
Consider the situation illustrated in Figure 14.2 in which, at  , a tight-fitting piston is suddenly pushed into a stationary gas, contained in a uniform tube, at the
steady speed
, a tight-fitting piston is suddenly pushed into a stationary gas, contained in a uniform tube, at the
steady speed 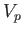 , generating a shock front that propagates away from the piston, and into the gas, at the speed
, generating a shock front that propagates away from the piston, and into the gas, at the speed 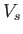 . Suppose that the
piston is located at
. Suppose that the
piston is located at  at
at  , and moves in the
, and moves in the 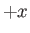 -direction.
The
gas can be divided into two regions. In region
-direction.
The
gas can be divided into two regions. In region 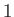 , which lies to the right of the shock wave (i.e.,
, which lies to the right of the shock wave (i.e., 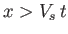 ), the gas remains
undisturbed. Hence, its velocity is
), the gas remains
undisturbed. Hence, its velocity is 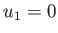 . Let its pressure, density, and temperature be
. Let its pressure, density, and temperature be 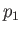 ,
, 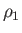 , and
, and 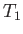 , respectively.
In region
, respectively.
In region 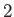 , which lies between the piston and the shock wave (i.e.,
, which lies between the piston and the shock wave (i.e.,
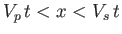 ), the gas is co-moving with the piston, so its velocity is
), the gas is co-moving with the piston, so its velocity is 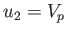 .
Let its pressure, density, and temperature, be
.
Let its pressure, density, and temperature, be 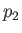 ,
, 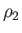 , and
, and 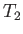 , respectively. Thus, the system is formally
the same as that discussed in the final paragraph of the previous section, provided that we make the identifications
, respectively. Thus, the system is formally
the same as that discussed in the final paragraph of the previous section, provided that we make the identifications 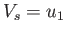 and
and
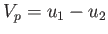 .
.
Figure 14.2:
A piston-generated shock wave.
 |
Let
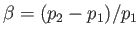 be our conventional measure of shock strength. It follows from Equations (14.111) and (14.112)
that
be our conventional measure of shock strength. It follows from Equations (14.111) and (14.112)
that
where 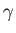 is the gas's ratio of specific heats.
We can define the Mach number of the shock wave as
is the gas's ratio of specific heats.
We can define the Mach number of the shock wave as
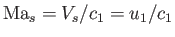 , where
, where 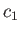 is the sound speed in the unperturbed gas. According to
Equation (14.110),
is the sound speed in the unperturbed gas. According to
Equation (14.110),
![$\displaystyle {\rm Ma}_s=\left[1+\left(\frac{\gamma+1}{2\,\gamma}\right)\beta\right]^{1/2}.$](img5533.png) |
(14.129) |
Finally, we can define the Mach number of the piston as
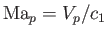 . Thus,
. Thus,
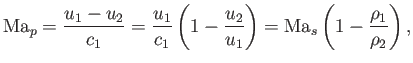 |
(14.130) |
where use has been made of Equation (14.111). Equations (14.127) and (14.129) can be combined with the previous equation to give
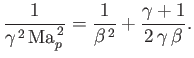 |
(14.131) |
Thus, Equations (14.127)-(14.129), and (14.131), fully determine the parameters of the shocked gas, as well as the
speed of the shock front, in terms of the parameters of the unperturbed gas and the piston speed. In particular,
for a weak shock (i.e.,
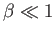 ),
),
whereas for a strong shock (i.e.,
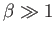 ),
),
Thus, a weak shock is associated with a subsonic piston (i.e.,
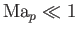 ), whereas a strong shock is associated with a
supersonic piston (i.e.,
), whereas a strong shock is associated with a
supersonic piston (i.e.,
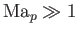 ).
).



Next: Piston-Generated Expansion Wave
Up: One-Dimensional Compressible Inviscid Flow
Previous: Normal Shocks
Richard Fitzpatrick
2016-01-22

![]() be our conventional measure of shock strength. It follows from Equations (14.111) and (14.112)
that
be our conventional measure of shock strength. It follows from Equations (14.111) and (14.112)
that
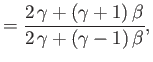
![$\displaystyle =(1+\beta)\left[\frac{2\,\gamma+(\gamma-1)\,\beta}{2\,\gamma+(\gamma+1)\,\beta}\right],$](img5531.png)