 |
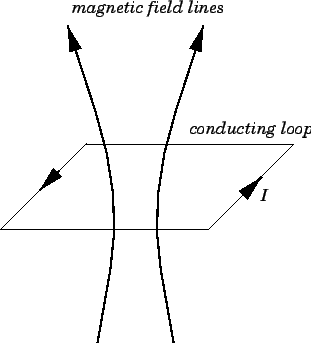
Consider a plane loop of conducting wire which is linked by magnetic flux. By convention, the direction in which current would have to flow around the loop in order to increase the magnetic flux linking the loop is termed the positive direction. Likewise, the direction in which current would have to flow around the loop in order to decrease the magnetic flux linking the loop is termed the negative direction. Suppose that the magnetic flux linking the loop is increased. In accordance with Faraday's law, an emf is generated around the loop. Does this emf act in the positive direction, so as to drive a current around the loop which further increases the magnetic flux, or does it act in the negative direction, so as to drive a current around the loop which decreases the magnetic flux? It is easily demonstrated experimentally that the emf acts in the negative direction. Thus:
The emf induced in an electric circuit always acts in such a direction that the current it drives around the circuit opposes the change in magnetic flux which produces the emf.This result is known as Lenz's law, after the nineteenth century Russian scientist Heinrich Lenz, who first formulated it. Faraday's law, combined with Lenz's law, is usually written
| (196) |