


Next: Electric Current
Up: Capacitance
Previous: Example 6.3: Equivalent capacitance
Question:
An air-filled parallel plate capacitor has a capacitance of 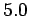 pF. A potential
of 100V is applied across the plates, which are
pF. A potential
of 100V is applied across the plates, which are 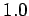 cm apart, using a
storage battery. What is the energy stored in the capacitor? Suppose that
the battery is disconnected, and the plates are moved until they are
cm apart, using a
storage battery. What is the energy stored in the capacitor? Suppose that
the battery is disconnected, and the plates are moved until they are 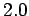 cm
apart. What now is the energy stored in the capacitor? Suppose, instead, that
the battery is left connected, and the plates are again moved until they are
cm
apart. What now is the energy stored in the capacitor? Suppose, instead, that
the battery is left connected, and the plates are again moved until they are
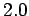 cm apart. What is the energy stored in the capacitor in this case?
cm apart. What is the energy stored in the capacitor in this case?
Answer: The initial energy stored in the
capacitor is
When the spacing between the plates is doubled, the capacitance of the capacitor
is halved to  pF. If the battery is disconnected then this process
takes place at constant charge
pF. If the battery is disconnected then this process
takes place at constant charge 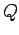 . Thus, it follows from the
formula
. Thus, it follows from the
formula
that the energy stored in the capacitor doubles. So, the
new energy is
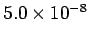 J. Incidentally, the increased energy
of the capacitor is accounted for by the work done in pulling
the capacitor plates apart (since these plates are oppositely charged,
they attract one another).
J. Incidentally, the increased energy
of the capacitor is accounted for by the work done in pulling
the capacitor plates apart (since these plates are oppositely charged,
they attract one another).
If the battery is left connected, then the capacitance is still halved, but now
the process takes place at constant voltage  . It follows from the
formula
. It follows from the
formula
that the energy stored in the capacitor is halved.
So, the new energy is
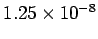 J. Incidentally, the energy lost
by the capacitor is given to the battery (in effect, it goes to re-charging the
battery). Likewise, the work done in pulling the plates apart is
also given to the battery.
J. Incidentally, the energy lost
by the capacitor is given to the battery (in effect, it goes to re-charging the
battery). Likewise, the work done in pulling the plates apart is
also given to the battery.



Next: Electric Current
Up: Capacitance
Previous: Example 6.3: Equivalent capacitance
Richard Fitzpatrick
2007-07-14
![]() pF. If the battery is disconnected then this process
takes place at constant charge
pF. If the battery is disconnected then this process
takes place at constant charge ![]() . Thus, it follows from the
formula
. Thus, it follows from the
formula
![]() . It follows from the
formula
. It follows from the
formula