


Next: Example 5.2: Motion of an
Up: Electric Potential
Previous: Worked Examples
Question: A charge of
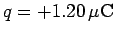 is placed in a
uniform
is placed in a
uniform 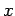 -directed electric field of magnitude
-directed electric field of magnitude
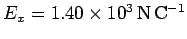 .
How much work must be performed in order to move the charge a distance
.
How much work must be performed in order to move the charge a distance 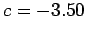 cm in the
cm in the
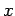 -direction? What is the potential difference between the initial and final positions
of the charge? If the electric field is produced by two oppositely
charged parallel plates
separated by a distance
-direction? What is the potential difference between the initial and final positions
of the charge? If the electric field is produced by two oppositely
charged parallel plates
separated by a distance 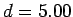 cm, what is the potential difference between the
plates?
cm, what is the potential difference between the
plates?
Solution: Let us denote the initial and final positions of the charge
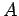 and
and 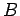 , respectively. The work which we must perform in order to move the
charge from
, respectively. The work which we must perform in order to move the
charge from 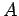 to
to 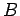 is minus the product of the electrostatic force on the
charge due to the electric field
(since the force we exert on the charge is minus this force)
and the distance that the charge moves in the
direction of this force [see Eq. (76)]. Thus,
is minus the product of the electrostatic force on the
charge due to the electric field
(since the force we exert on the charge is minus this force)
and the distance that the charge moves in the
direction of this force [see Eq. (76)]. Thus,
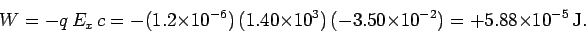
Note that the work is positive. This makes sense, because we would have to do real
work (i.e., we would lose energy) in order to move a positive charge in the opposite direction
to an electric field (i.e., against the direction of the electrostatic
force acting on the charge).
The work done on the charge goes to increase its electric
potential energy, so 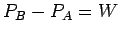 . By definition, this increase in
potential energy is equal to the product of the potential difference
. By definition, this increase in
potential energy is equal to the product of the potential difference
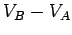 between points
between points 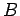 and
and 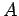 , and the magnitude of the charge
, and the magnitude of the charge 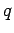 .
Thus,
.
Thus,
giving
Note that the electric field is directed from point 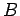 to point
to point 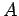 , and
that the former point is at a higher potential than the latter.
, and
that the former point is at a higher potential than the latter.
It is clear, from the above formulae, that the magnitude of the potential difference
between two points in a uniform electric field is simply the product of the
electric field-strength and the distance between the two points (in the direction
of the field). Thus, the potential difference between the two metal plates is
If the electric field is directed from plate 1 (the positively charged plate)
to plate 2 (the negatively charged plate) then the former plate is at the
higher potential.



Next: Example 5.2: Motion of an
Up: Electric Potential
Previous: Worked Examples
Richard Fitzpatrick
2007-07-14
![]() . By definition, this increase in
potential energy is equal to the product of the potential difference
. By definition, this increase in
potential energy is equal to the product of the potential difference
![]() between points
between points ![]() and
and ![]() , and the magnitude of the charge
, and the magnitude of the charge ![]() .
Thus,
.
Thus,