


Next: Capacitance
Up: Electric Potential
Previous: Example 5.3: Electric potential due
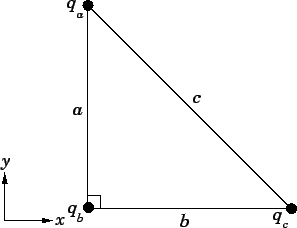
Question: Suppose that three point charges, 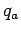 ,
, 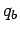 , and
, and 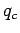 , are arranged at the
vertices of a right-angled triangle, as shown in the diagram. What is the absolute electric potential of the third charge if
, are arranged at the
vertices of a right-angled triangle, as shown in the diagram. What is the absolute electric potential of the third charge if
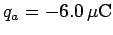 ,
,
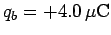 ,
,
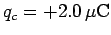 ,
,
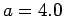 m, and
m, and 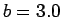 m? Suppose that the third charge, which is initially
at rest, is repelled to infinity by the
combined electric field of the other two charges, which are held fixed. What is the final kinetic energy
of the third charge?
m? Suppose that the third charge, which is initially
at rest, is repelled to infinity by the
combined electric field of the other two charges, which are held fixed. What is the final kinetic energy
of the third charge?
Solution: The absolute electric potential of the third charge due to the presence of the
first charge is
where use has been made of the Pythagorean theorem.
Likewise, the absolute electric potential of the third charge due to the presence of the second
charge is
The net absolute potential of the third charge 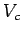 is simply the algebraic sum of the
potentials due to the other two charges taken in isolation. Thus,
is simply the algebraic sum of the
potentials due to the other two charges taken in isolation. Thus,
The change in electric potential energy of the third charge as it moves from its initial
position to infinity is the product of the third charge, 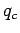 , and the difference in electric
potential (
, and the difference in electric
potential (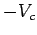 ) between infinity and the initial position. It follows that
) between infinity and the initial position. It follows that
This decrease in the potential energy of the
charge is offset by a corresponding increase
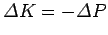 in its kinetic energy. Since the initial kinetic energy of the third charge is zero (because it
is initially at rest), the final kinetic energy is simply
in its kinetic energy. Since the initial kinetic energy of the third charge is zero (because it
is initially at rest), the final kinetic energy is simply



Next: Capacitance
Up: Electric Potential
Previous: Example 5.3: Electric potential due
Richard Fitzpatrick
2007-07-14

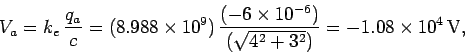
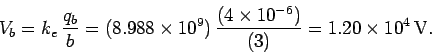
![]() , and the difference in electric
potential (
, and the difference in electric
potential (![]() ) between infinity and the initial position. It follows that
) between infinity and the initial position. It follows that