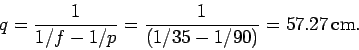Next: Example 13.4: Diverging lenses
Up: Paraxial Optics
Previous: Example 13.2: Convex mirrors
Question: An object of height 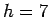 cm is placed
a distance
cm is placed
a distance 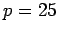 cm in front of a thin converging lens of focal length
cm in front of a thin converging lens of focal length
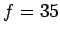 cm. What is the height, location, and nature
of the image? Suppose that the object is moved to a
new location a distance
cm. What is the height, location, and nature
of the image? Suppose that the object is moved to a
new location a distance 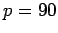 cm in front of the
lens. What now is the height, location, and nature of the image?
cm in front of the
lens. What now is the height, location, and nature of the image?
Answer: According to Eq. (367), the image distance  is given by
is given by
Thus, the image is virtual (since 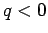 ), and is located
), and is located
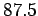 cm in front of the lens. According to Eq. (10.24),
the magnification
cm in front of the lens. According to Eq. (10.24),
the magnification 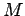 of the image is given by
of the image is given by
Thus, the image is upright (since 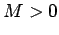 ), and magnified
by a factor of
), and magnified
by a factor of 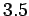 . It follows that the height
. It follows that the height 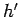 of the image
is given by
of the image
is given by
If the object is moved such that 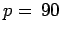 cm then the
new image distance is given by
cm then the
new image distance is given by
Thus, the new image is real (since 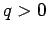 ), and is
located 57.27cm behind the lens. The new magnification is
given by
), and is
located 57.27cm behind the lens. The new magnification is
given by
Thus, the image is inverted (since 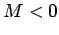 ), and
diminished by a factor of
), and
diminished by a factor of 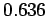 . It follows that the
new height of the image
is
. It follows that the
new height of the image
is
Note that the height is negative because the image is inverted.



Next: Example 13.4: Diverging lenses
Up: Paraxial Optics
Previous: Example 13.2: Convex mirrors
Richard Fitzpatrick
2007-07-14
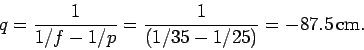
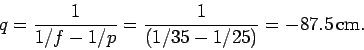
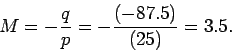
![]() cm then the
new image distance is given by
cm then the
new image distance is given by