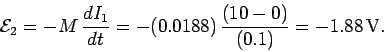Next: Example 10.2: Energy density
Up: Inductance
Previous: Worked Examples
Question: Suppose that two insulated wires are wound onto a common
cylindrical former of length 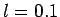 m and cross-sectional area
m and cross-sectional area
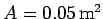 . The number of turns in the first wire is
. The number of turns in the first wire is 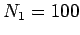 , and
the number of turns in the second wire is
, and
the number of turns in the second wire is 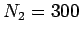 . What is the mutual
inductance of the two wires? If the current
. What is the mutual
inductance of the two wires? If the current 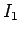 flowing in the
first wire increases uniformly from 0 to
flowing in the
first wire increases uniformly from 0 to 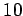 A in
A in 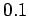 s, what emf is
generated in the second wire? Does this emf act to drive a current in the
second wire which circulates in the same sense as the current in the first wire,
or the opposite sense?
s, what emf is
generated in the second wire? Does this emf act to drive a current in the
second wire which circulates in the same sense as the current in the first wire,
or the opposite sense?
Answer: From Eq. (240), the mutual inductance of the two wires is
From Eq. (236), the emf generated around the second loop by the changing current
in the first loop is
The minus sign indicates that this emf acts so as to drive a current in the
second wire which circulates in the opposite sense to the current flowing
in the first wire, in accordance with Lenz's law. If the current in the
first wire were decreased, instead of increased, then the emf in the second
wire would act to drive a current which circulates in the same sense as
the former current.



Next: Example 10.2: Energy density
Up: Inductance
Previous: Worked Examples
Richard Fitzpatrick
2007-07-14