Historical background

Humankind has always been fascinated by the night sky, and, in particular, by the movements of the Sun, the Moon, and the objects which the ancient Greeks called plantai (``wanderers''), and which we call planets. In ancient times, much of this interest was of a practical nature. The Sun and the Moon were important for determining the calendar, and also for navigation. Moreover, the planets were vital to astrology: i.e., the belief--almost universally prevalent in the ancient world--that the positions of the planets in the sky could be used to foretell important events.

Actually, there were only seven ``wandering'' heavenly bodies visible to ancient peoples: the Sun, the Moon, and the five planets--Mercury, Venus, Mars, Jupiter, and Saturn. The ancients believed that the stars were fixed to a ``celestial sphere'' which formed the outer boundary of the Universe. However, it was recognized that the wandering bodies were located within this sphere: e.g., because the Moon clearly passes in front of, and blocks the light from, stars in its path. It was also recognized that some bodies were closer to the Earth than others. For instance, ancient astronomers noted that the Moon occasionally passes in front of the Sun and each of the planets. Moreover, Mercury and Venus can sometimes be seen to transit in front of the Sun.

The first scientific model of the Solar System was outlined by the Greek philosopher Eudoxas of Cnidus (409-356BC). According to this model, the Sun, the Moon, and the planets all execute uniform circular orbits around the Earth--which is fixed, and non-rotating. The order of the orbits is as follows: Moon, Mercury, Venus, Sun, Mars, Jupiter, Saturn--with the Moon closest to the Earth. For obvious reasons, Eudoxas' model became known as the geocentric model of the Solar System. Note that orbits are circular in this model for philosophical reasons. The ancients believed the heavens to be the realm of perfection. Since a circle is the most ``perfect'' imaginable shape, it follows that heavenly objects must execute circular orbits.

A second Greek philosopher, Aristarchus of Samos (310-230BC), proposed an alternative model in which the Earth and the planets execute uniform circular orbits around the Sun--which is fixed. Moreover, the Moon orbits around the Earth, and the Earth rotates daily about a North-South axis. The order of the planetary orbits is as follows: Mercury, Venus, Earth, Mars, Jupiter, Saturn--with Mercury closest to the Sun. This model became known as the heliocentric model of the Solar System.

The heliocentric model was generally rejected by the ancient philosophers for three main reasons:

1. If the Earth is rotating about its axis, and orbiting around the Sun, then the Earth must be in motion. However, we cannot ``feel'' this motion. Nor does this motion give rise to any obvious observational consequences. Hence, the Earth must be stationary.
2. If the Earth is executing a circular orbit around the Sun then the positions of the stars should be slightly different when the Earth is on opposite sides of the Sun. This effect is known as parallax. Since no stellar parallax is observable (at least, with the naked eye), the Earth must be stationary. In order to appreciate the force of this argument, it is important to realize that ancient astronomers did not suppose the stars to be significantly further away from the Earth than the planets. The celestial sphere was assumed to lie just beyond the orbit of Saturn.
3. The geocentric model is far more philosophically attractive than the heliocentric model, since in the former model the Earth occupies a privileged position in the Universe.

The geocentric model was first converted into a proper scientific theory, capable of accurate predictions, by the Alexandrian philosopher Claudius Ptolemy (85-165AD). The theory that Ptolemy proposed in his famous book, now known as the Almagest, remained the dominant scientific picture of the Solar System for over a millennium. Basically, Ptolemy acquired and extended the extensive set of planetary observations of his predecessor Hipparchus, and then constructed a geocentric model capable of accounting for them. However, in order to fit the observations, Ptolemy was forced to make some significant modifications to the original model of Eudoxas. Let us discuss these modifications.

Figure 100: The Ptolemaic system.

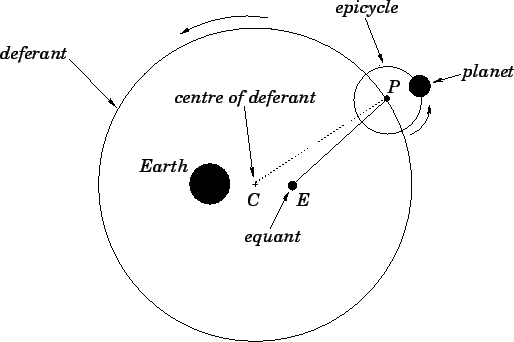
First, we need to introduce some terminology. As shown in Fig. 100, deferants are large circles centred on the Earth, and epicyles are small circles whose centres move around the circumferences of the deferants. In the Ptolemaic system, instead of traveling around deferants, the planets move around the circumference of epicycles, which, in turn, move around the circumference of deferants. Ptolemy found, however, that this modification was insufficient to completely account for all of his data. Ptolemy's second modification to Eudoxas' model was to displace the Earth slightly from the common centre of the deferants. Moreover, Ptolemy assumed that the Sun, Moon, and planets rotate uniformly about an imaginary point, called the equant, which is displaced an equal distance in the opposite direction to the Earth from the centre of the deferants. In other words, Ptolemy assumed that the line $EP$, in Fig. 100, rotates uniformly, rather than the line $CP$.

Figure 101 shows more details of the Ptolemaic model.² Note that this diagram is not drawn to scale, and the displacement of the Earth from the centre of the deferants has been omitted for the sake of clarity. It can be seen that the Moon and the Sun do not possess epicyles. Moreover, the motions of the inferior planets (i.e., Mercury and Venus) are closely linked to the motion of the Sun. In fact, the centres of the inferior planet epicycles move on an imaginary line connecting the Earth and the Sun. Furthermore, the radius vectors connecting the superior planets (i.e., Mars, Jupiter, and Saturn) to the centres of their epicycles are always parallel to the geometric line connecting the Earth and the Sun. Note that, in addition to the motion indicated in the diagram, all of the heavenly bodies (including the stars) rotate clockwise (assuming that we are looking down on the Earth's North pole in Fig. 101) with a period of 1 day. Finally, there are epicycles within the epicycles shown in the diagram. In fact, some planets need as many as 28 epicycles to account for all the details of their motion. These subsidiary epicycles are not shown in the diagram, for the sake of clarity.

As is quite apparent, the Ptolemaic model of the Solar System is extremely complicated. However, it successfully accounted for the relatively crude naked eye observations made by the ancient Greeks. The Sun-linked epicyles of the inferior planets are needed to explain why these objects always remain close to the Sun in the sky. The epicycles of the superior planets are needed to account for their occasional bouts of retrograde motion: i.e., motion in the opposite direction to their apparent direction of rotation around the Earth. Finally, the displacement of the Earth from the centre of the deferants, as well as the introduction of the equant as the centre of uniform rotation, is needed to explain why the planets speed up slightly when they are close to the Earth (and, hence, appear brighter in the night sky), and slow down when they are further away.

Figure 101: The Ptolemaic model of the Solar System.

Ptolemy's model of the Solar System was rescued from the wreck of ancient European civilization by the Roman Catholic Church, which, unfortunately, converted it into a minor article of faith, on the basis of a few references in the Bible which seemed to imply that the Earth is stationary and the Sun is moving (e.g., Joshua 10:12-13, Habakkuk 3:11). Consequently, this model was not subject to proper scientific criticism for over a millennium. Having said this, few medieval or renaissance philosophers were entirely satisfied with Ptolemy's model. Their dissatisfaction focused, not on the many epicycles (which to the modern eye seem rather absurd), but on the displacement of the Earth from the centre of the deferants, and the introduction of the equant as the centre of uniform rotation. Recall, that the only reason planetary orbits are constructed from circles in Ptolemy's model is to preserve the assumed ideal symmetry of the heavens. Unfortunately, this symmetry is severely compromised when the Earth is displaced from the apparent centre of the Universe. This problem so perplexed the Polish priest-astronomer Nicolaus Copernicus (1473-1543) that he eventually decided to reject the geocentric model, and revive the heliocentric model of Aristarchus. After many years of mathematical calculations, Copernicus published a book entitled De revolutionibus orbium coelestium (On the revolutions of the celestial spheres) in 1543 which outlined his new heliocentric theory.

Figure 102: The Copernican model of the Solar System.

Copernicus' model is illustrated in Fig. 102. Again, this diagram is not to scale. The planets execute uniform circular orbits about the Sun, and the Moon orbits about the Earth. Finally, the Earth revolves about its axis daily. Note that there is no displacement of the Sun from the centres of the planetary orbits, and there is no equant. Moreover, in this model, the inferior planets remain close to the Sun in the sky without any special synchronization of their orbits. Furthermore, the occasional retrograde motion of the superior planets has a more natural explanation than in Ptolemy's model. Since the Earth orbits more rapidly than the superior planets, it occasionally ``overtakes'' them, and they appear to move backward in the night sky, in much the same manner that slow moving cars on a freeway appears to move backward to a driver overtaking them. Copernicus accounted for the lack of stellar parallax, due to the Earth's motion, by postulating that the stars were a lot further away than had previously been supposed, rendering any parallax undetectably small. Unfortunately, Copernicus insisted on retaining uniform circular motion in his model (after all, he was trying to construct a more symmetric model than that of Ptolemy). Consequently, Copernicus also had to resort to epicycles to fit the data. In fact, Copernicus' model ended up with more epicycles than Ptolemy's!

The real breakthrough in the understanding of planetary motion occurred--as most breakthroughs in physics occur--when better data became available. The data in question was produced by the Dane Tycho Brahe (1546-1601), who devoted his life to making naked eye astronomical observations of unprecedented accuracy and detail. This data was eventually inherited by Brahe's pupil and assistant, the German scientist Johannes Kepler (1571-1630). Kepler fully accepted Copernicus' heliocentric theory of the Solar System. Moreover, he was just as firm a believer as Copernicus in the perfection of the heavens, and the consequent need for circular motion of planetary bodies. The main difference was that Kepler's observational data was considerably better than Copernicus'. After years of fruitless effort, Kepler eventually concluded that no combination of circular deferants and epicycles could completely account for his data. At this stage, he started to think the unthinkable. Maybe, planetary motion was not circular after all? After more calculations, Kepler was eventually able to formulate three extraordinarily simple laws which completely accounted for Brahe's observations. These laws are as follows:

1. The planets move in elliptical orbits with the Sun at one focus.
2. A line from the Sun to any given planet sweeps out equal areas in equal time intervals.
3. The square of a planet's period is proportional to the cube of the planet's mean distance from the Sun.

Note that there are no epicyles or equants in Kepler's model of the Solar System.

Figure 103 illustrates Kepler's second law. Here, the ellipse represents a planetary orbit, and $S$ represents the Sun, which is located at one of the focii of the ellipse. Suppose that the planet moves from point $A$ to point $B$ in the same time it takes to move from point $C$ to point $D$. According the Kepler's second law, the areas of the elliptic segments $ASB$ and $CSD$ are equal. Note that this law basically mandates that planets speed up when they move closer to the Sun.

Figure 103: Kepler's second law.

Table 5 illustrates Kepler's third law. The mean distance, $a$, and orbital period, $T$, as well as the ratio $a^3/T^2$, are listed for each of the first six planets in the Solar System. It can be seen that the ratio $a^3/T^2$ is indeed constant from planet to planet.

Table 5: Kepler's third law. Here, $a$ is the mean distance from the Sun, measured in Astronomical Units (1 AU is the mean Earth-Sun distance), and $T$ is the orbital period, measured in years.

Planet	$a({\rm AU})$	$T({\rm yr})$	$a^3/T^2$
Mercury	0.387	0.241	0.998
Venus	0.723	0.615	0.999
Earth	1.000	1.000	1.000
Mars	1.524	1.881	1.000
Jupiter	5.203	11.862	1.001
Saturn	9.516	29.458	0.993

Since we have now definitely adopted a heliocentric model of the Solar System, let us discuss the ancient Greek objections to such a model, listed earlier. We have already dealt with the second objection (the absence of stellar parallax) by stating that the stars are a lot further away from the Earth than the ancient Greeks supposed. The third objection (that it is philosophically more attractive to have the Earth at the centre of the Universe) is not a valid scientific criticism. What about the first objection? If the Earth is rotating about its axis, and also orbiting the Sun, why do we not ``feel'' this motion? At first sight, this objection appears to have some force. After all, the rotation velocity of the Earth's surface is about $460 {\rm m/s}$. Moreover, the Earth's orbital velocity is approximately $30 {\rm km/s}$. Surely, we would notice if we were moving this rapidly? Of course, this reasoning is faulty because we know, from Newton's laws of motion, that we only ``feel'' the acceleration associated with motion, not the motion itself. It turns out that the acceleration at the Earth's surface due to its axial rotation is only about $0.034 {\rm m/s^2}$. Moreover, the Earth's acceleration due to its orbital motion is only $0.0059 {\rm m/s^2}$. Nowadays, we can detect such small accelerations, but the ancient Greeks certainly could not.

Kepler correctly formulated the three laws of planetary motion in 1619. Almost seventy years later, in 1687, Isaac Newton published his Principia, in which he presented, for the first time, a universal theory of motion. Newton then went on to illustrate his theory by using it to deriving Kepler's laws from first principles. Let us now discuss Newton's monumental achievement in more detail.