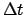 (JD) (JD) |
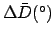 |
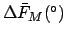 |
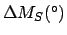 |
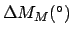 |
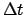 (JD) (JD) |
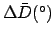 |
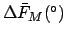 |
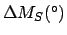 |
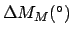 |
| |
|
|
|
|
|
|
|
|
|
| 10,000 |
227.491 |
173.503 |
136.002 |
329.930 |
1,000 |
310.749 |
269.350 |
265.600 |
104.993 |
| 20,000 |
94.982 |
347.005 |
272.005 |
299.859 |
2,000 |
261.498 |
178.701 |
171.200 |
209.986 |
| 30,000 |
322.473 |
160.508 |
48.007 |
269.788 |
3,000 |
212.247 |
88.051 |
76.801 |
314.979 |
| 40,000 |
189.964 |
334.011 |
184.010 |
239.718 |
4,000 |
162.996 |
357.401 |
342.401 |
59.972 |
| 50,000 |
57.455 |
147.513 |
320.012 |
209.648 |
5,000 |
113.746 |
266.751 |
248.001 |
164.965 |
| 60,000 |
284.947 |
321.016 |
96.015 |
179.577 |
6,000 |
64.495 |
176.102 |
153.601 |
269.958 |
| 70,000 |
152.438 |
134.519 |
232.017 |
149.506 |
7,000 |
15.244 |
85.452 |
59.202 |
14.951 |
| 80,000 |
19.929 |
308.022 |
8.020 |
119.436 |
8,000 |
325.993 |
354.802 |
324.802 |
119.944 |
| 90,000 |
247.420 |
121.524 |
144.022 |
89.366 |
9,000 |
276.742 |
264.152 |
230.402 |
224.937 |
| |
|
|
|
|
|
|
|
|
|
| 100 |
139.075 |
242.935 |
98.560 |
226.499 |
10 |
121.907 |
132.294 |
9.856 |
130.650 |
| 200 |
278.150 |
125.870 |
197.120 |
92.999 |
20 |
243.815 |
264.587 |
19.712 |
261.300 |
| 300 |
57.225 |
8.805 |
295.680 |
319.498 |
30 |
5.722 |
36.881 |
29.568 |
31.950 |
| 400 |
196.300 |
251.740 |
34.240 |
185.997 |
40 |
127.630 |
169.174 |
39.424 |
162.600 |
| 500 |
335.375 |
134.675 |
132.800 |
52.496 |
50 |
249.537 |
301.468 |
49.280 |
293.250 |
| 600 |
114.449 |
17.610 |
231.360 |
278.996 |
60 |
11.445 |
73.761 |
59.136 |
63.900 |
| 700 |
253.524 |
260.545 |
329.920 |
145.495 |
70 |
133.352 |
206.055 |
68.992 |
194.550 |
| 800 |
32.599 |
143.480 |
68.480 |
11.994 |
80 |
255.260 |
338.348 |
78.848 |
325.199 |
| 900 |
171.674 |
26.415 |
167.040 |
238.494 |
90 |
17.167 |
110.642 |
88.704 |
95.849 |
| |
|
|
|
|
|
|
|
|
|
| 1 |
12.191 |
13.229 |
0.986 |
13.065 |
0.1 |
1.219 |
1.323 |
0.099 |
1.306 |
| 2 |
24.381 |
26.459 |
1.971 |
26.130 |
0.2 |
2.438 |
2.646 |
0.197 |
2.613 |
| 3 |
36.572 |
39.688 |
2.957 |
39.195 |
0.3 |
3.657 |
3.969 |
0.296 |
3.919 |
| 4 |
48.763 |
52.917 |
3.942 |
52.260 |
0.4 |
4.876 |
5.292 |
0.394 |
5.226 |
| 5 |
60.954 |
66.147 |
4.928 |
65.325 |
0.5 |
6.095 |
6.615 |
0.493 |
6.532 |
| 6 |
73.144 |
79.376 |
5.914 |
78.390 |
0.6 |
7.314 |
7.938 |
0.591 |
7.839 |
| 7 |
85.335 |
92.605 |
6.899 |
91.455 |
0.7 |
8.534 |
9.261 |
0.690 |
9.145 |
| 8 |
97.526 |
105.835 |
7.885 |
104.520 |
0.8 |
9.753 |
10.583 |
0.788 |
10.452 |
| 9 |
109.717 |
119.064 |
8.870 |
117.585 |
0.9 |
10.972 |
11.906 |
0.887 |
11.758 |
|