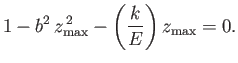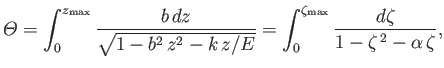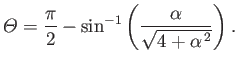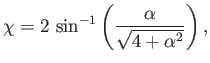Next: Rutherford Scattering Cross-Section
Up: Collisions
Previous: Boltzmann H-Theorem
Two-Body Coulomb Collisions
Consider a two-body Coulomb collision between a particle of species 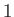 , with mass
, with mass 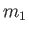 and charge
and charge 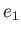 , and a particle of species
, and a particle of species 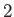 ,
with mass
,
with mass 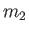 and charge
and charge 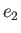 . The equations of motion of the two particles take the form
. The equations of motion of the two particles take the form
where
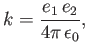 |
(3.58) |
Here, 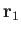 and
and 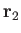 are the respective position vectors, and
are the respective position vectors, and
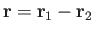 is
the relative position vector.
It is easily demonstrated that
is
the relative position vector.
It is easily demonstrated that
where
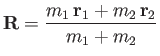 |
(3.61) |
is the vector position of the center of mass (which does not accelerate), and
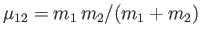 the reduced mass. Equations (3.56) and (3.57) can be combined to give a single
equation of relative motion,
the reduced mass. Equations (3.56) and (3.57) can be combined to give a single
equation of relative motion,
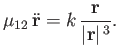 |
(3.62) |
Two relations that immediately follow from the previous equation are
where
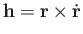 |
(3.65) |
is the conserved angular momentum per unit mass, and
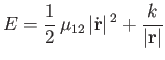 |
(3.66) |
the conserved energy.
Equation (3.65) implies that
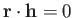 . This is the equation of a plane that passes through the origin, and whose normal is parallel
to the constant vector
. This is the equation of a plane that passes through the origin, and whose normal is parallel
to the constant vector 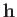 . We, therefore, conclude that the relative position vector
. We, therefore, conclude that the relative position vector 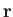 is constrained to lie in this plane,
which implies that the trajectories of both colliding particles are coplanar.
Let the plane
is constrained to lie in this plane,
which implies that the trajectories of both colliding particles are coplanar.
Let the plane
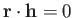 coincide with the
coincide with the 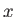 -
-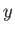 plane, so that we can write
plane, so that we can write
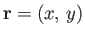 . It is convenient to
define the standard plane polar coordinates
. It is convenient to
define the standard plane polar coordinates
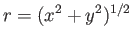 and
and
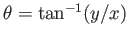 .
When expressed in terms of these coordinates, the conserved angular momentum per unit mass becomes
.
When expressed in terms of these coordinates, the conserved angular momentum per unit mass becomes
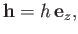 |
(3.67) |
where
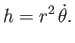 |
(3.68) |
Furthermore, the conserved energy takes the form
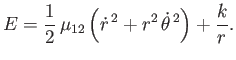 |
(3.69) |
Suppose that
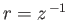 , where
, where
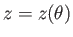 and
and
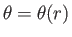 . It follows that
. It follows that
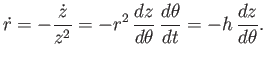 |
(3.70) |
Hence, Equation (3.69) transforms to give
![$\displaystyle E = \frac{1}{2}\,\mu_{12}\,h^2\left[\left(\frac{dz}{d\theta}\right)^2 + z^2\right] + k\,z.$](img730.png) |
(3.71) |
It is convenient to define the relative velocity at large distances,
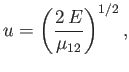 |
(3.72) |
as well as the impact parameter,
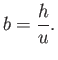 |
(3.73) |
The latter parameter is simply the distance of closest approach of the two particles in the situation in which there is no Coulomb force acting between them,
and they, consequently, move in straight-lines. (See Figure 3.1.) The previous three equations can be combined to give
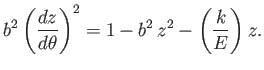 |
(3.74) |
Figure 3.1:
A two-body Coulomb collision.
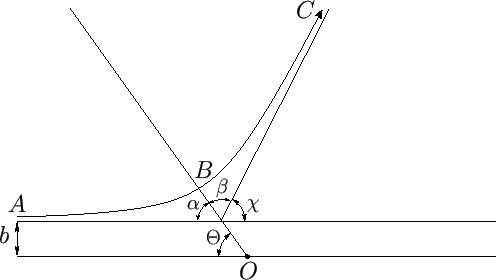 |
Figure 3.1 shows the collision in a frame of reference in which particle  remains stationary at the origin,
remains stationary at the origin, 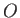 ,
whereas particle
,
whereas particle 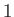 traces out the path
traces out the path 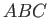 . Point
. Point 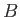 corresponds to the closest approach of the
two particles. It follows, by symmetry (because Coulomb collisions are reversible), that the angles
corresponds to the closest approach of the
two particles. It follows, by symmetry (because Coulomb collisions are reversible), that the angles 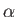 and
and 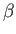 shown in the figure are equal to one another. Hence, we deduce that
shown in the figure are equal to one another. Hence, we deduce that
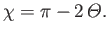 |
(3.75) |
Here, 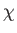 is the angle through which the path of particle
is the angle through which the path of particle 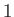 (or particle
(or particle 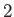 ) is deviated as a consequence of the collision,
whereas
) is deviated as a consequence of the collision,
whereas
 is the angle through which the relative position vector,
is the angle through which the relative position vector, 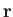 , rotates as particle
, rotates as particle 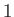 moves from point
moves from point 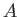 (which is assumed to be infinity far from point
(which is assumed to be infinity far from point 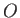 ) to point
) to point 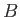 . Suppose that point
. Suppose that point 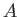 corresponds to
corresponds to 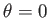 . It follows that
. It follows that
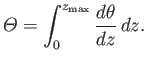 |
(3.76) |
Here,
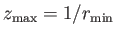 , where
, where
 is the distance of closest approach.
Now, by symmetry,
is the distance of closest approach.
Now, by symmetry,
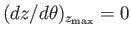 , so Equation (3.74) implies that
, so Equation (3.74) implies that
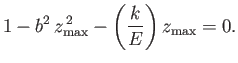 |
(3.77) |
Combining Equations (3.74) and (3.76), we obtain
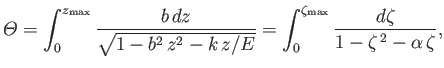 |
(3.78) |
where
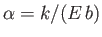 , and
, and
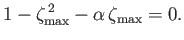 |
(3.79) |
Integration (Speigel, Liu, and Lipschutz 1999) yields
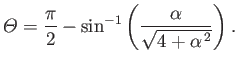 |
(3.80) |
Hence, from Equation (3.75), we get
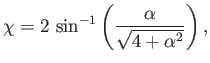 |
(3.81) |
which can be rearranged to give
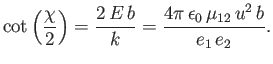 |
(3.82) |



Next: Rutherford Scattering Cross-Section
Up: Collisions
Previous: Boltzmann H-Theorem
Richard Fitzpatrick
2016-01-23
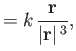

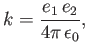
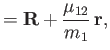
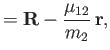
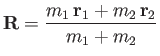
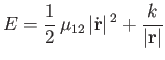
![]() . This is the equation of a plane that passes through the origin, and whose normal is parallel
to the constant vector
. This is the equation of a plane that passes through the origin, and whose normal is parallel
to the constant vector ![]() . We, therefore, conclude that the relative position vector
. We, therefore, conclude that the relative position vector ![]() is constrained to lie in this plane,
which implies that the trajectories of both colliding particles are coplanar.
Let the plane
is constrained to lie in this plane,
which implies that the trajectories of both colliding particles are coplanar.
Let the plane
![]() coincide with the
coincide with the ![]() -
-![]() plane, so that we can write
plane, so that we can write
![]() . It is convenient to
define the standard plane polar coordinates
. It is convenient to
define the standard plane polar coordinates
![]() and
and
![]() .
When expressed in terms of these coordinates, the conserved angular momentum per unit mass becomes
.
When expressed in terms of these coordinates, the conserved angular momentum per unit mass becomes
![]() , where
, where
![]() and
and
![]() . It follows that
. It follows that
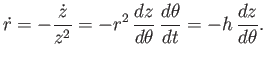
![$\displaystyle E = \frac{1}{2}\,\mu_{12}\,h^2\left[\left(\frac{dz}{d\theta}\right)^2 + z^2\right] + k\,z.$](img730.png)
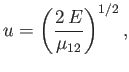
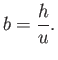
![]() remains stationary at the origin,
remains stationary at the origin, ![]() ,
whereas particle
,
whereas particle ![]() traces out the path
traces out the path ![]() . Point
. Point ![]() corresponds to the closest approach of the
two particles. It follows, by symmetry (because Coulomb collisions are reversible), that the angles
corresponds to the closest approach of the
two particles. It follows, by symmetry (because Coulomb collisions are reversible), that the angles ![]() and
and ![]() shown in the figure are equal to one another. Hence, we deduce that
shown in the figure are equal to one another. Hence, we deduce that