


Next: Exercises
Up: Magnetohydrodynamic Fluids
Previous: Perpendicular MHD Shocks
Oblique MHD Shocks
Let us now consider the general case in which the plasma velocities and
the magnetic fields on each side of the shock are neither parallel nor
perpendicular to the shock front. It is convenient to transform
into the so-called de Hoffmann-Teller frame in which
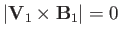 , or
, or
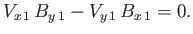 |
(7.278) |
In other words, it is convenient to transform to a frame that moves at the local
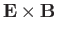 velocity of the plasma.
It immediately follows from the jump condition (7.251) that
velocity of the plasma.
It immediately follows from the jump condition (7.251) that
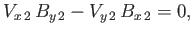 |
(7.279) |
or
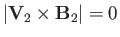 . Thus, in the de Hoffmann-Teller frame, the upstream plasma
flow is parallel to the upstream magnetic field, and the downstream plasma
flow is also parallel to the downstream magnetic field. Furthermore, the magnetic contribution to the jump
condition (7.255) becomes identically zero, which is a considerable simplification.
. Thus, in the de Hoffmann-Teller frame, the upstream plasma
flow is parallel to the upstream magnetic field, and the downstream plasma
flow is also parallel to the downstream magnetic field. Furthermore, the magnetic contribution to the jump
condition (7.255) becomes identically zero, which is a considerable simplification.
Equations (7.278) and (7.279) can be combined with the
general jump conditions (7.250)-(7.255)
to give
where
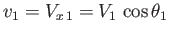 is the component of the upstream velocity normal to the
shock front,
and
is the component of the upstream velocity normal to the
shock front,
and 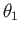 is the angle subtended between the upstream plasma flow and the
shock front normal. Finally, given the compression ratio,
is the angle subtended between the upstream plasma flow and the
shock front normal. Finally, given the compression ratio, 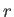 , the square of the normal
upstream velocity,
, the square of the normal
upstream velocity, 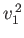 , is a real root of a cubic equation
known as the shock adiabatic:
, is a real root of a cubic equation
known as the shock adiabatic:
As before, the second law of thermodynamics mandates that 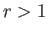 .
.
Let us first consider the weak shock limit
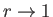 . In this case, it is easily seen that the three roots of the
shock adiabatic reduce to
. In this case, it is easily seen that the three roots of the
shock adiabatic reduce to
However, from Section 7.4, we recognize these velocities as belonging to slow, intermediate
(or shear-Alfvén), and fast waves, respectively, propagating in the normal direction to the
shock front. We conclude that slow, intermediate, and fast MHD shocks degenerate into the
associated MHD waves in the limit of small shock amplitude. Conversely, we can think of
the various MHD shocks as nonlinear versions of the associated MHD waves. Now, it can be demonstrated that
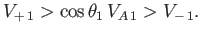 |
(7.290) |
In other words, a fast wave travels faster than an intermediate wave, which travels faster than a slow
wave. It is reasonable to suppose that the same is true of the associated MHD
shocks, at least at relatively low shock strength.
It follows from Equation (7.282) that
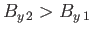 for a fast shock,
whereas
for a fast shock,
whereas
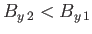 for a slow shock. For the case of an intermediate shock, we
can show, after a little algebra, that
for a slow shock. For the case of an intermediate shock, we
can show, after a little algebra, that
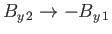 in the limit
in the limit
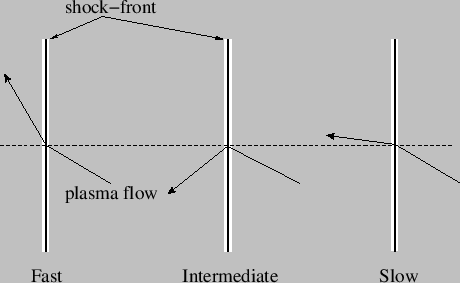
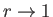 . We conclude that (in the de Hoffmann-Teller frame) fast shocks refract the magnetic field and plasma
flow (recall that they are parallel in our adopted frame of the reference) away from
the normal to the shock front, whereas slow shocks refract these quantities toward
the normal. Moreover, the tangential magnetic field and plasma flow generally reverse
across an intermediate shock front. This is illustrated in Figure 7.12.
. We conclude that (in the de Hoffmann-Teller frame) fast shocks refract the magnetic field and plasma
flow (recall that they are parallel in our adopted frame of the reference) away from
the normal to the shock front, whereas slow shocks refract these quantities toward
the normal. Moreover, the tangential magnetic field and plasma flow generally reverse
across an intermediate shock front. This is illustrated in Figure 7.12.
Figure 7.12:
Characteristic plasma flow patterns across the three different types of MHD shock
in the de Hoffmann-Teller frame.
 |
When 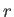 is slightly larger than unity, it is easily demonstrated that the conditions for the
existence of a slow, intermediate, and fast shock are
is slightly larger than unity, it is easily demonstrated that the conditions for the
existence of a slow, intermediate, and fast shock are
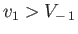 ,
,
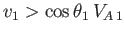 , and
, and
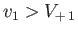 , respectively.
, respectively.
Let us now consider the strong shock limit,
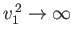 . In this case, the shock
adiabatic yields
. In this case, the shock
adiabatic yields
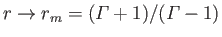 , and
, and
![$\displaystyle v_1^{\,2} \simeq \frac{r_m}{{\mit\Gamma}-1}\,\frac{2\,V_{S\,1}^{\...
...\sin^2\theta_1\,[{\mit\Gamma} + (2-{\mit\Gamma})\,r_m]\,V_{A\,1}^{\,2}}{r_m-r}.$](img2937.png) |
(7.291) |
There are no other real roots. The previous root is clearly a type of
fast shock. The fact that there is only one real root suggests that there exists a critical
shock strength above which the slow and intermediate shock solutions cease to exist. In fact,
they merge and annihilate one another (Gurnett and Bhattacharjee 2005).
In other words, there is a limit to the strength of a slow or an intermediate shock.
On the other hand, there is no limit to the strength of a fast shock. Note, however, that
the plasma density and tangential
magnetic field cannot be compressed by more than a
factor
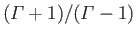 by any type of MHD shock.
by any type of MHD shock.
Consider the special case
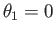 in which both the plasma flow and the
magnetic field are normal to the shock front. In this case, the three roots of the shock adiabatic are
in which both the plasma flow and the
magnetic field are normal to the shock front. In this case, the three roots of the shock adiabatic are
We recognize the first of these roots as the hydrodynamic shock discussed in Section 7.19 [see Equation (7.260)].
This shock is classified as a slow shock when
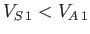 , and as a fast shock
when
, and as a fast shock
when
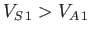 . The other two roots are identical, and correspond to
shocks that propagate at the velocity
. The other two roots are identical, and correspond to
shocks that propagate at the velocity
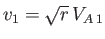 and ``switch-on" the tangential
components of the plasma flow and the magnetic field: that is, it can be seen from
Equations (7.282) and (7.284) that
and ``switch-on" the tangential
components of the plasma flow and the magnetic field: that is, it can be seen from
Equations (7.282) and (7.284) that
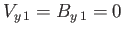 while
while
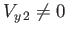 and
and
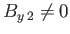 for these types of shock. Incidentally, it is also
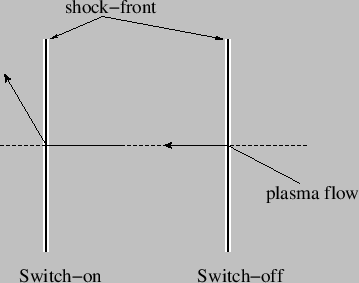
possible to have a ``switch-off'' shock that eliminates the tangential components
of the plasma flow and the magnetic field. According to Equations (7.282) and (7.284),
such a shock propagates at the velocity
for these types of shock. Incidentally, it is also
possible to have a ``switch-off'' shock that eliminates the tangential components
of the plasma flow and the magnetic field. According to Equations (7.282) and (7.284),
such a shock propagates at the velocity
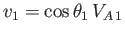 . Switch-on and
switch-off shocks are illustrated in Figure 7.13.
. Switch-on and
switch-off shocks are illustrated in Figure 7.13.
Figure 7.13:
Characteristic plasma flow patterns across switch-on and switch-off shocks
in the de Hoffmann-Teller frame.
 |
Let us, finally, consider the special case
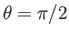 . As is easily demonstrated, the three roots of the
shock adiabatic are
. As is easily demonstrated, the three roots of the
shock adiabatic are
The first of these roots is clearly a fast shock, and is identical to the perpendicular
shock discussed in Section 7.20, except that there is no plasma flow across the shock
front in this case. The fact that the two other roots are zero indicates
that, like the corresponding MHD waves, slow and intermediate MHD shocks do
not propagate perpendicular to the magnetic field.
MHD shocks have been observed in a large variety of situations. For instance, shocks are
known to be formed by supernova explosions, by strong stellar winds, by solar flares, and
by the solar wind upstream of planetary magnetospheres (Gurnett and Bhattacharjee 2005).



Next: Exercises
Up: Magnetohydrodynamic Fluids
Previous: Perpendicular MHD Shocks
Richard Fitzpatrick
2016-01-23
![]() . In this case, it is easily seen that the three roots of the
shock adiabatic reduce to
. In this case, it is easily seen that the three roots of the
shock adiabatic reduce to
![$\displaystyle =V_{-\,1}^{\,2}\equiv \frac{V_{A\,1}^{\,2}+V_{S\,1}^{\,2}- [(V_{A...
..._{S\,1})^{\,2} -4\,\cos^2\theta_1\,V_{S\,1}^{\,2}\,V_{A\,1}^{\,2}]^{\,1/2}}{2},$](img2924.png)
![$\displaystyle =V_{+\,1}^{\,2}\equiv \frac{V_{A\,1}^{\,2}+V_{S\,1}^{\,2} + [(V_{...
..._{S\,1})^{\,2} -4\,\cos^2\theta_1\,V_{S\,1}^{\,2}\,V_{A\,1}^{\,2}]^{\,1/2}}{2}.$](img2926.png)

![]() is slightly larger than unity, it is easily demonstrated that the conditions for the
existence of a slow, intermediate, and fast shock are
is slightly larger than unity, it is easily demonstrated that the conditions for the
existence of a slow, intermediate, and fast shock are
![]() ,
,
![]() , and
, and
![]() , respectively.
, respectively.
![]() . In this case, the shock
adiabatic yields
. In this case, the shock
adiabatic yields
![]() , and
, and
![$\displaystyle v_1^{\,2} \simeq \frac{r_m}{{\mit\Gamma}-1}\,\frac{2\,V_{S\,1}^{\...
...\sin^2\theta_1\,[{\mit\Gamma} + (2-{\mit\Gamma})\,r_m]\,V_{A\,1}^{\,2}}{r_m-r}.$](img2937.png)
![]() in which both the plasma flow and the
magnetic field are normal to the shock front. In this case, the three roots of the shock adiabatic are
in which both the plasma flow and the
magnetic field are normal to the shock front. In this case, the three roots of the shock adiabatic are
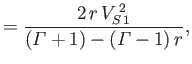

![]() . As is easily demonstrated, the three roots of the
shock adiabatic are
. As is easily demonstrated, the three roots of the
shock adiabatic are
![$\displaystyle = r \left(\frac{2\,V_{S\,1}^{\,2} + [{\mit\Gamma}+(2-{\mit\Gamma})\,r]\,V_{A\,1}^{\,2}} {[{\mit\Gamma}+1]-[{\mit\Gamma}-1]\,r}\right) ,$](img2950.png)