


Next: Transmission lines
Up: Magnetic induction
Previous: Magnetic energy
Alternating current (AC) circuits are made up of e.m.f. sources and three
different types of passive element: resistors, inductors,
and capacitors, Resistors satisfy Ohm's law:
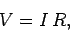 |
(968) |
where 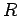 is the resistance,
is the resistance, 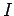 the current flowing through the resistor, and
the current flowing through the resistor, and
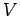 the voltage drop across the resistor (in the direction in which the current
flows). Inductors satisfy
the voltage drop across the resistor (in the direction in which the current
flows). Inductors satisfy
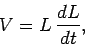 |
(969) |
where 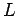 is the inductance. Finally, capacitors obey
is the inductance. Finally, capacitors obey
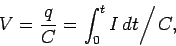 |
(970) |
where 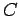 is the capacitance,
is the capacitance, 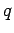 is the charge stored on the plate with the most
positive potential, and
is the charge stored on the plate with the most
positive potential, and 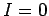 for
for 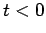 . Note that any
passive component of a real electrical
circuit can always be represented as a combination of ideal resistors, inductors, and
capacitors.
. Note that any
passive component of a real electrical
circuit can always be represented as a combination of ideal resistors, inductors, and
capacitors.
Let us consider the classic LCR circuit, which consists of an inductor, 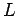 , a
capacitor,
, a
capacitor, 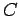 , and a resistor,
, and a resistor, 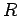 , all connected in series with an e.m.f. source,
, all connected in series with an e.m.f. source,
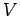 . The circuit equation is obtained by setting the input voltage
. The circuit equation is obtained by setting the input voltage 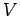 equal to
the sum of the voltage drops across the three passive elements in the circuit.
Thus,
equal to
the sum of the voltage drops across the three passive elements in the circuit.
Thus,
 |
(971) |
This is an integro-differential equation which, in general, is quite tricky to
solve. Suppose, however, that both the voltage and the current
oscillate at some fixed angular frequency 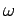 , so that
, so that
where the physical solution is understood to be the real part of
the above expressions. The assumed behaviour of the voltage and current is
clearly relevant to electrical
circuits powered by the mains voltage (which oscillates at 60 hertz).
Equations (971)-(973) yield
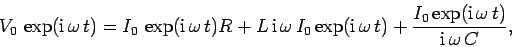 |
(974) |
giving
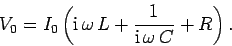 |
(975) |
It is helpful to define the impedance of the circuit:
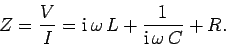 |
(976) |
Impedance is a generalization of the concept of resistance. In general, the impedance
of an AC circuit is a complex quantity.
The average power output of the e.m.f. source is
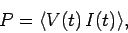 |
(977) |
where the average is taken over one period of the oscillation. Let us, first of all,
calculate the power using real (rather than complex) voltages and currents.
We can write
where 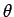 is the phase-lag of the current with respect to the voltage.
It follows that
is the phase-lag of the current with respect to the voltage.
It follows that
giving
 |
(981) |
since
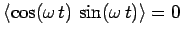 and
and
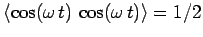 .
In complex representation, the voltage and the current are written
.
In complex representation, the voltage and the current are written
Note that
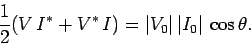 |
(984) |
It follows that
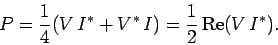 |
(985) |
Making use of Eq. (976), we find that
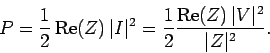 |
(986) |
Note that power dissipation is associated with the real part of the impedance.
For the special case of an LCR circuit,
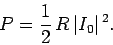 |
(987) |
It is clear that only the resistor dissipates energy in this circuit. The inductor and
the capacitor both store energy, but they eventually return it to the circuit
without dissipation.
According to Eq. (976), the amplitude of the current which flows in an LCR circuit
for a given amplitude of the input voltage is
given by
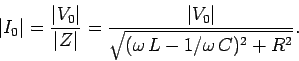 |
(988) |
The response of the circuit is
clearly resonant, peaking at
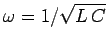 , and reaching
, and reaching
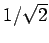 of the peak value at
of the peak value at
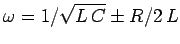 (assuming that
(assuming that
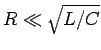 ). In fact, LCR circuits are used in radio tuners to filter out
signals whose frequencies fall outside a given band.
). In fact, LCR circuits are used in radio tuners to filter out
signals whose frequencies fall outside a given band.
The phase-lag of the current with respect to the voltage is given by
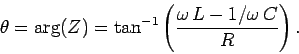 |
(989) |
The phase-lag varies from 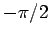 for frequencies significantly below the resonant
frequency, to zero at the resonant frequency (
for frequencies significantly below the resonant
frequency, to zero at the resonant frequency (
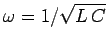 ), to
), to
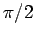 for frequencies significantly above the resonant frequency.
for frequencies significantly above the resonant frequency.
It is clear that in conventional AC circuits the circuit equation reduces to a
simple algebraic equation, and the behaviour of the circuit is summed up
by the complex impedance 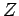 . The real part of
. The real part of 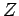 tells us the power dissipated in
the circuit, the magnitude of
tells us the power dissipated in
the circuit, the magnitude of 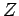 gives the ratio of the peak current to the
peak voltage, and the argument of
gives the ratio of the peak current to the
peak voltage, and the argument of 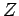 gives the phase-lag of the current
with respect to the voltage.
gives the phase-lag of the current
with respect to the voltage.



Next: Transmission lines
Up: Magnetic induction
Previous: Magnetic energy
Richard Fitzpatrick
2006-02-02
![]() , a
capacitor,
, a
capacitor, ![]() , and a resistor,
, and a resistor, ![]() , all connected in series with an e.m.f. source,
, all connected in series with an e.m.f. source,
![]() . The circuit equation is obtained by setting the input voltage
. The circuit equation is obtained by setting the input voltage ![]() equal to
the sum of the voltage drops across the three passive elements in the circuit.
Thus,
equal to
the sum of the voltage drops across the three passive elements in the circuit.
Thus,
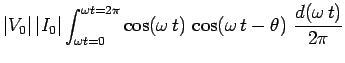
![$\displaystyle \vert V_0\vert \vert I_0\vert \int_{\omega t =0}^{\omega t = 2\...
...s\theta + \sin(\omega t) \sin \theta\right]
\frac{d(\omega t)}{2\pi},$](img2019.png)
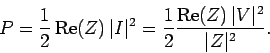
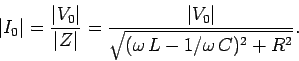
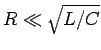 ). In fact, LCR circuits are used in radio tuners to filter out
signals whose frequencies fall outside a given band.
). In fact, LCR circuits are used in radio tuners to filter out
signals whose frequencies fall outside a given band.
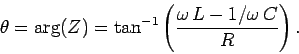
![]() . The real part of
. The real part of ![]() tells us the power dissipated in
the circuit, the magnitude of
tells us the power dissipated in
the circuit, the magnitude of ![]() gives the ratio of the peak current to the
peak voltage, and the argument of
gives the ratio of the peak current to the
peak voltage, and the argument of ![]() gives the phase-lag of the current
with respect to the voltage.
gives the phase-lag of the current
with respect to the voltage.