


Next: Self-inductance
Up: Magnetic induction
Previous: Introduction
We have learned about e.m.f., resistance, and capacitance. Let us now investigate inductance.
Electrical engineers like to reduce all pieces of electrical apparatus to an
equivalent circuit consisting only of e.m.f. sources (e.g., batteries),
inductors, capacitors, and resistors. Clearly, once we understand inductors, we shall
be ready to apply the laws of electromagnetism to electrical circuits.
Consider two stationary loops of wire, labeled 1 and 2. Let us run a steady current
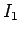 around the first loop to produce a magnetic field
around the first loop to produce a magnetic field 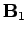 . Some of the field lines
of
. Some of the field lines
of 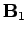 will pass through the second loop. Let
will pass through the second loop. Let 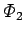 be the flux
of
be the flux
of 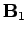 through loop 2:
through loop 2:
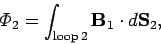 |
(896) |
where 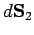 is a surface element of loop 2.
This flux is generally quite difficult to calculate exactly (unless the two loops
have a particularly simple geometry). However, we can infer from the Biot-Savart law,
is a surface element of loop 2.
This flux is generally quite difficult to calculate exactly (unless the two loops
have a particularly simple geometry). However, we can infer from the Biot-Savart law,
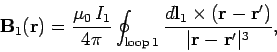 |
(897) |
that the magnitude of 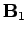 is proportional to the current
is proportional to the current 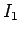 .
This is
ultimately a consequence of the linearity of Maxwell's equations.
Here,
.
This is
ultimately a consequence of the linearity of Maxwell's equations.
Here, 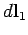 is a line element of loop 1 located at position
vector
is a line element of loop 1 located at position
vector 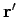 .
It follows that
the flux
.
It follows that
the flux 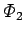 must also be proportional to
must also be proportional to 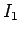 . Thus, we can write
. Thus, we can write
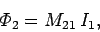 |
(898) |
where 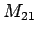 is the constant of proportionality. This constant is called
the mutual inductance of the two loops.
is the constant of proportionality. This constant is called
the mutual inductance of the two loops.
Let us write the magnetic field 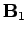 in terms of a vector potential
in terms of a vector potential 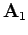 , so that
, so that
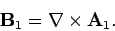 |
(899) |
It follows from Stokes' theorem that
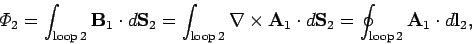 |
(900) |
where 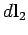 is a line element of loop 2.
However, we know that
is a line element of loop 2.
However, we know that
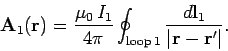 |
(901) |
The above equation is just a special case of the more general law,
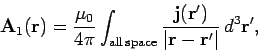 |
(902) |
for
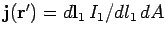 and
and
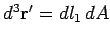 , where
, where
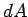 is the cross-sectional area of loop 1. Thus,
is the cross-sectional area of loop 1. Thus,
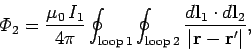 |
(903) |
where 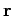 is now the position vector of the line element
is now the position vector of the line element 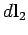 of loop 2. The above equation implies that
of loop 2. The above equation implies that
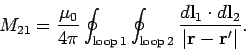 |
(904) |
In fact, mutual
inductances are rarely worked out from first principles--it is usually
too difficult. However, the above formula tells us two important things.
Firstly, the mutual inductance of two loops is a purely geometric quantity,
having to do with the sizes, shapes, and relative orientations of the loops.
Secondly, the integral is unchanged if we switch the roles of loops 1 and 2.
In other words,
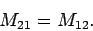 |
(905) |
In fact, we can drop the subscripts, and just call these quantities 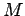 .
This is a rather surprising result. It implies that no matter what the shapes and
relative positions of the two loops, the magnetic flux through loop 2 when we run a
current
.
This is a rather surprising result. It implies that no matter what the shapes and
relative positions of the two loops, the magnetic flux through loop 2 when we run a
current 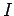 around loop 1 is exactly the same as the flux through loop 1
when we send the same current around loop 2.
around loop 1 is exactly the same as the flux through loop 1
when we send the same current around loop 2.
We have seen that a current 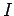 flowing around some loop, 1, generates a magnetic
flux linking some other loop, 2. However, flux is also generated through the
first loop. As before, the magnetic field, and, therefore, the flux
flowing around some loop, 1, generates a magnetic
flux linking some other loop, 2. However, flux is also generated through the
first loop. As before, the magnetic field, and, therefore, the flux 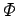 ,
is proportional to the current, so we can write
,
is proportional to the current, so we can write
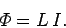 |
(906) |
The constant of proportionality 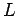 is called the self-inductance. Like
is called the self-inductance. Like
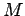 it only depends on the geometry of the loop.
it only depends on the geometry of the loop.
Inductance is measured in S.I. units called henries (H): 1 henry is 1 volt-second
per ampere. The henry, like the farad, is a rather unwieldy unit, since most real-life inductors have a inductances of order a micro-henry.



Next: Self-inductance
Up: Magnetic induction
Previous: Introduction
Richard Fitzpatrick
2006-02-02
![]() around the first loop to produce a magnetic field
around the first loop to produce a magnetic field ![]() . Some of the field lines
of
. Some of the field lines
of ![]() will pass through the second loop. Let
will pass through the second loop. Let ![]() be the flux
of
be the flux
of ![]() through loop 2:
through loop 2:
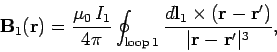
![]() in terms of a vector potential
in terms of a vector potential ![]() , so that
, so that
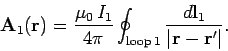
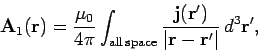
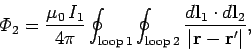
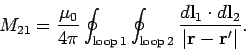
![]() flowing around some loop, 1, generates a magnetic
flux linking some other loop, 2. However, flux is also generated through the
first loop. As before, the magnetic field, and, therefore, the flux
flowing around some loop, 1, generates a magnetic
flux linking some other loop, 2. However, flux is also generated through the
first loop. As before, the magnetic field, and, therefore, the flux ![]() ,
is proportional to the current, so we can write
,
is proportional to the current, so we can write