


Next: Ferromagnetism
Up: Dielectric and magnetic media
Previous: Magnetization
In a large class of materials, there exists an approximately linear
relationship between 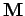 and
and  . If the material
is isotropic then
. If the material
is isotropic then
 |
(866) |
where 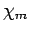 is called the magnetic susceptibility. If
is called the magnetic susceptibility. If 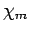 is
positive then the material is called paramagnetic, and the magnetic field
is strengthened by the presence of the material. On the other hand, if
is
positive then the material is called paramagnetic, and the magnetic field
is strengthened by the presence of the material. On the other hand, if 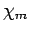 is
negative then the material is diamagnetic, and the magnetic field
is weakened in the presence of the material. The magnetic
susceptibilities of paramagnetic and diamagnetic materials are
generally extremely small. A few sample values are given in Table. 2.1
is
negative then the material is diamagnetic, and the magnetic field
is weakened in the presence of the material. The magnetic
susceptibilities of paramagnetic and diamagnetic materials are
generally extremely small. A few sample values are given in Table. 2.1
Table 2:
| Material |
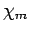 |
| Aluminium |
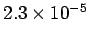 |
| Copper |
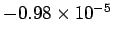 |
| Diamond |
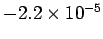 |
| Tungsten |
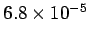 |
| Hydrogen (1 atm) |
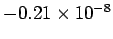 |
| Oxygen (1 atm) |
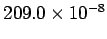 |
| Nitrogen (1 atm) |
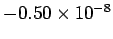 |
|
A linear relationship between 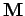 and
and  also
implies a linear relationship between
also
implies a linear relationship between 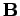 and
and  .
In fact, we can write
.
In fact, we can write
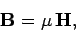 |
(867) |
where
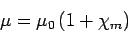 |
(868) |
is termed the magnetic permeability of the material
in question. (Likewise,
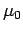 is termed the permeability of free space.) Note that
is termed the permeability of free space.) Note that 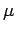 has the same units as
has the same units as 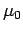 .
It is clear from
Table 2 that the permeabilities of common diamagnetic and paramagnetic
materials do not differ substantially from the permeability of free space. In fact,
to all intents and purposes, the magnetic properties of such materials
can be safely neglected (i.e.,
.
It is clear from
Table 2 that the permeabilities of common diamagnetic and paramagnetic
materials do not differ substantially from the permeability of free space. In fact,
to all intents and purposes, the magnetic properties of such materials
can be safely neglected (i.e., 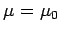 ).
).



Next: Ferromagnetism
Up: Dielectric and magnetic media
Previous: Magnetization
Richard Fitzpatrick
2006-02-02
![]() and
and ![]() also
implies a linear relationship between
also
implies a linear relationship between ![]() and
and ![]() .
In fact, we can write
.
In fact, we can write