


Next: Worked Examples
Up: Electric Current
Previous: Energy in DC Circuits
Power and Internal Resistance
Consider a simple circuit in which a battery of emf 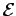 and internal
resistance
and internal
resistance 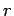 drives a current
drives a current 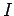 through an external resistor of resistance
through an external resistor of resistance 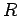 (see Fig. 17). The external resistor is usually referred
to as the load resistor. It could stand for either an electric light,
an electric heating element, or, maybe, an electric motor. The
basic purpose of
the circuit is to transfer energy from the battery to the load, where it actually
does something useful for us (e.g., lighting
a light bulb, or lifting a weight). Let us see to what extent the internal resistance
of the battery interferes with this process.
(see Fig. 17). The external resistor is usually referred
to as the load resistor. It could stand for either an electric light,
an electric heating element, or, maybe, an electric motor. The
basic purpose of
the circuit is to transfer energy from the battery to the load, where it actually
does something useful for us (e.g., lighting
a light bulb, or lifting a weight). Let us see to what extent the internal resistance
of the battery interferes with this process.
The equivalent resistance of the circuit is 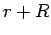 (since the load resistance is
in series with the internal resistance), so the current flowing in the
circuit is given by
(since the load resistance is
in series with the internal resistance), so the current flowing in the
circuit is given by
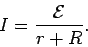 |
(145) |
The power output of the emf is simply
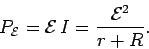 |
(146) |
The power dissipated as heat by the internal resistance of the battery is
 |
(147) |
Likewise, the power transferred to the load is
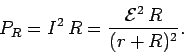 |
(148) |
Note that
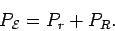 |
(149) |
Thus, some of the power output of the battery is immediately lost as heat dissipated by the
internal resistance of the battery. The remainder is transmitted to the load.
Let
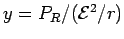 and
and 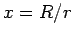 . It follows from
Eq. (148) that
. It follows from
Eq. (148) that
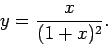 |
(150) |
The function 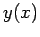 increases monotonically from zero for
increasing
increases monotonically from zero for
increasing 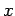 in the range
in the range 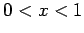 , attains
a maximum value of
, attains
a maximum value of 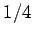 at
at 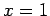 , and then decreases monotonically with increasing
, and then decreases monotonically with increasing
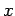 in the range
in the range 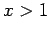 . In other words, if the load resistance
. In other words, if the load resistance 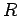 is varied at
constant
is varied at
constant 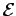 and
and 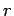 then the transferred power attains a maximum
value of
then the transferred power attains a maximum
value of
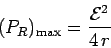 |
(151) |
when 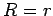 . This is a very important result in electrical engineering.
Power transfer between a voltage source and an external load is at its most efficient when the
resistance of the load matches the internal resistance of the voltage source.
If the load resistance is too low then most of the power output of the voltage
source is dissipated as heat inside the source itself. If the load resistance
is too high then the current which flows in the circuit is too low to
transfer energy to the load at an appreciable rate. Note that in the optimum case,
. This is a very important result in electrical engineering.
Power transfer between a voltage source and an external load is at its most efficient when the
resistance of the load matches the internal resistance of the voltage source.
If the load resistance is too low then most of the power output of the voltage
source is dissipated as heat inside the source itself. If the load resistance
is too high then the current which flows in the circuit is too low to
transfer energy to the load at an appreciable rate. Note that in the optimum case,
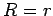 , only half of the power output of the voltage source
is transmitted to the load. The other half is dissipated as heat inside
the source.
Incidentally, electrical engineers call the process by which the resistance of
a load is matched to that of the power supply impedance matching
(impedance is just a fancy name for resistance).
, only half of the power output of the voltage source
is transmitted to the load. The other half is dissipated as heat inside
the source.
Incidentally, electrical engineers call the process by which the resistance of
a load is matched to that of the power supply impedance matching
(impedance is just a fancy name for resistance).



Next: Worked Examples
Up: Electric Current
Previous: Energy in DC Circuits
Richard Fitzpatrick
2007-07-14
![]() (since the load resistance is
in series with the internal resistance), so the current flowing in the
circuit is given by
(since the load resistance is
in series with the internal resistance), so the current flowing in the
circuit is given by

![]() and
and ![]() . It follows from
Eq. (148) that
. It follows from
Eq. (148) that